Question Number 88580 by jagoll last updated on 11/Apr/20
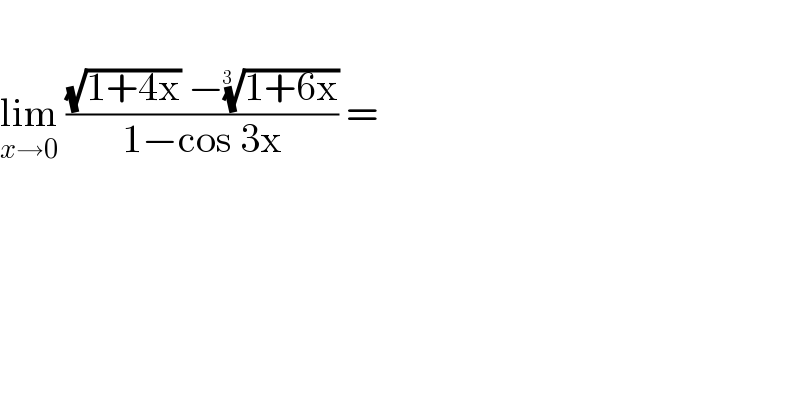
$$ \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{1}+\mathrm{4x}}\:−\sqrt[{\mathrm{3}\:\:}]{\mathrm{1}+\mathrm{6x}}}{\mathrm{1}−\mathrm{cos}\:\mathrm{3x}}\:= \\ $$
Commented by mathmax by abdo last updated on 11/Apr/20
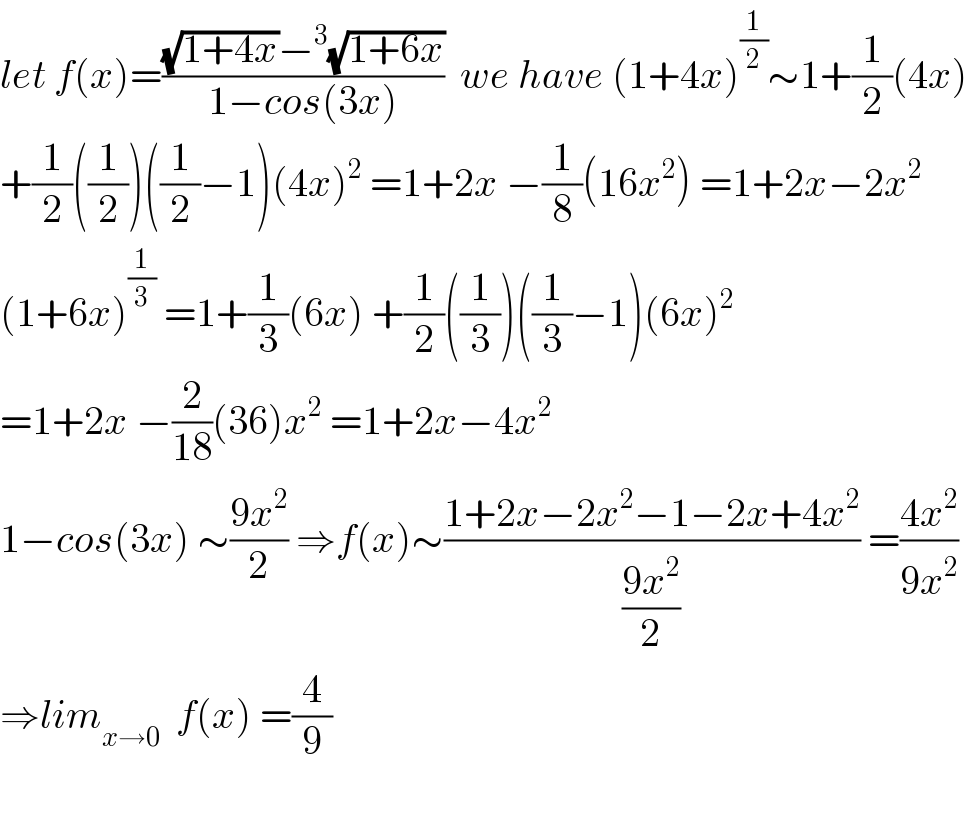
$${let}\:{f}\left({x}\right)=\frac{\sqrt{\mathrm{1}+\mathrm{4}{x}}−^{\mathrm{3}} \sqrt{\mathrm{1}+\mathrm{6}{x}}}{\mathrm{1}−{cos}\left(\mathrm{3}{x}\right)}\:\:{we}\:{have}\:\left(\mathrm{1}+\mathrm{4}{x}\right)^{\frac{\mathrm{1}}{\mathrm{2}}} \sim\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{4}{x}\right) \\ $$$$+\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\left(\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}\right)\left(\mathrm{4}{x}\right)^{\mathrm{2}} \:=\mathrm{1}+\mathrm{2}{x}\:−\frac{\mathrm{1}}{\mathrm{8}}\left(\mathrm{16}{x}^{\mathrm{2}} \right)\:=\mathrm{1}+\mathrm{2}{x}−\mathrm{2}{x}^{\mathrm{2}} \\ $$$$\left(\mathrm{1}+\mathrm{6}{x}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{6}{x}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{3}}\right)\left(\frac{\mathrm{1}}{\mathrm{3}}−\mathrm{1}\right)\left(\mathrm{6}{x}\right)^{\mathrm{2}} \\ $$$$=\mathrm{1}+\mathrm{2}{x}\:−\frac{\mathrm{2}}{\mathrm{18}}\left(\mathrm{36}\right){x}^{\mathrm{2}} \:=\mathrm{1}+\mathrm{2}{x}−\mathrm{4}{x}^{\mathrm{2}} \\ $$$$\mathrm{1}−{cos}\left(\mathrm{3}{x}\right)\:\sim\frac{\mathrm{9}{x}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow{f}\left({x}\right)\sim\frac{\mathrm{1}+\mathrm{2}{x}−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{1}−\mathrm{2}{x}+\mathrm{4}{x}^{\mathrm{2}} }{\frac{\mathrm{9}{x}^{\mathrm{2}} }{\mathrm{2}}}\:=\frac{\mathrm{4}{x}^{\mathrm{2}} }{\mathrm{9}{x}^{\mathrm{2}} } \\ $$$$\Rightarrow{lim}_{{x}\rightarrow\mathrm{0}} \:\:{f}\left({x}\right)\:=\frac{\mathrm{4}}{\mathrm{9}} \\ $$$$ \\ $$
Commented by jagoll last updated on 11/Apr/20

$$\mathrm{i}\:\mathrm{got}\:\mathrm{0}.\:\mathrm{it}\:\mathrm{correct}? \\ $$
Commented by jagoll last updated on 11/Apr/20
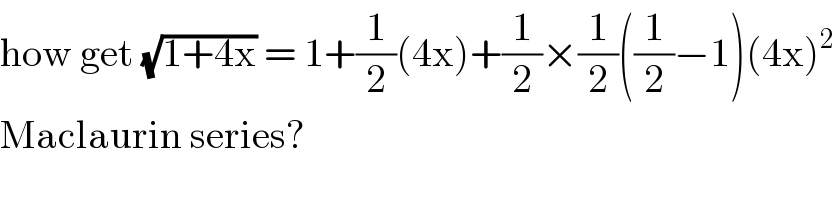
$$\mathrm{how}\:\mathrm{get}\:\sqrt{\mathrm{1}+\mathrm{4x}}\:=\:\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{4x}\right)+\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}\right)\left(\mathrm{4x}\right)^{\mathrm{2}} \\ $$$$\mathrm{Maclaurin}\:\mathrm{series}? \\ $$
Commented by mathmax by abdo last updated on 11/Apr/20
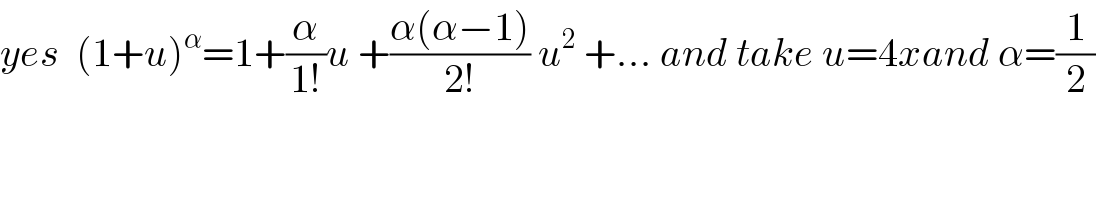
$${yes}\:\:\left(\mathrm{1}+{u}\right)^{\alpha} =\mathrm{1}+\frac{\alpha}{\mathrm{1}!}{u}\:+\frac{\alpha\left(\alpha−\mathrm{1}\right)}{\mathrm{2}!}\:{u}^{\mathrm{2}} \:+…\:{and}\:{take}\:{u}=\mathrm{4}{xand}\:\alpha=\frac{\mathrm{1}}{\mathrm{2}} \\ $$
