Menu Close

lim-x-0-1-cos-5-x-cos-3-2x-cos-3-3x-22x-2-

Tinku Tara 0 Comments
Pin




Question Number 105104 by bemath last updated on 26/Jul/20
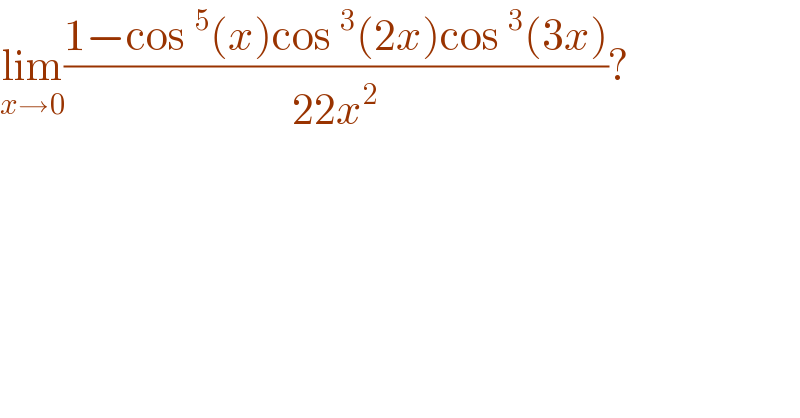
lim_(x→0) ((1−cos^5 (x)cos^3 (2x)cos^3 (3x))/(22x^2 ))?
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\mathrm{cos}\:^{\mathrm{5}} \left({x}\right)\mathrm{cos}\:^{\mathrm{3}} \left(\mathrm{2}{x}\right)\mathrm{cos}\:^{\mathrm{3}} \left(\mathrm{3}{x}\right)}{\mathrm{22}{x}^{\mathrm{2}} }? \\ $$
Answered by bramlex last updated on 26/Jul/20
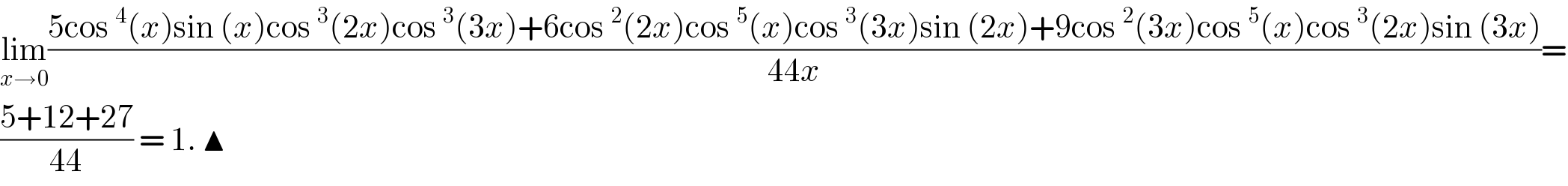
lim_(x→0) ((5cos^4 (x)sin (x)cos^3 (2x)cos^3 (3x)+6cos^2 (2x)cos^5 (x)cos^3 (3x)sin (2x)+9cos^2 (3x)cos^5 (x)cos^3 (2x)sin (3x))/(44x))= ((5+12+27)/(44)) = 1. ▲
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{5cos}\:^{\mathrm{4}} \left({x}\right)\mathrm{sin}\:\left({x}\right)\mathrm{cos}\:^{\mathrm{3}} \left(\mathrm{2}{x}\right)\mathrm{cos}\:^{\mathrm{3}} \left(\mathrm{3}{x}\right)+\mathrm{6cos}\:^{\mathrm{2}} \left(\mathrm{2}{x}\right)\mathrm{cos}\:^{\mathrm{5}} \left({x}\right)\mathrm{cos}\:^{\mathrm{3}} \left(\mathrm{3}{x}\right)\mathrm{sin}\:\left(\mathrm{2}{x}\right)+\mathrm{9cos}\:^{\mathrm{2}} \left(\mathrm{3}{x}\right)\mathrm{cos}\:^{\mathrm{5}} \left({x}\right)\mathrm{cos}\:^{\mathrm{3}} \left(\mathrm{2}{x}\right)\mathrm{sin}\:\left(\mathrm{3}{x}\right)}{\mathrm{44}{x}}= \\ $$$$\frac{\mathrm{5}+\mathrm{12}+\mathrm{27}}{\mathrm{44}}\:=\:\mathrm{1}.\:\blacktriangle \\ $$
Answered by bobhans last updated on 26/Jul/20
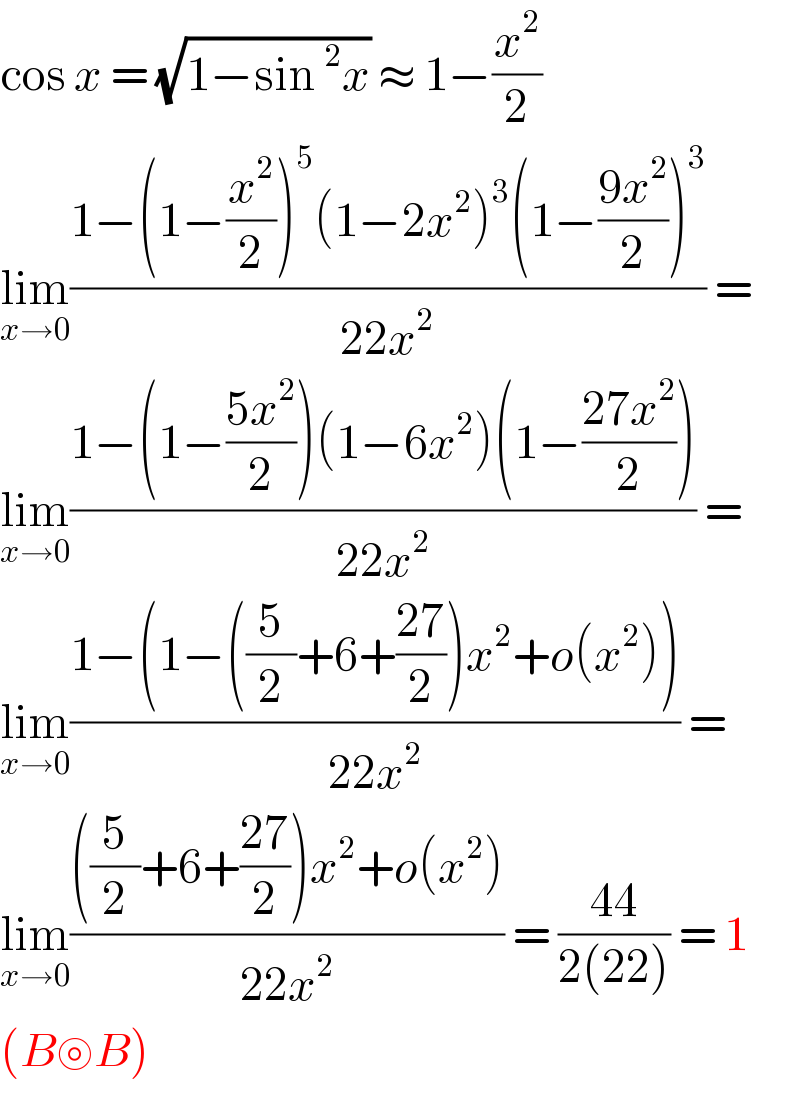
cos x = (√(1−sin^2 x)) ≈ 1−(x^2 /2) lim_(x→0) ((1−(1−(x^2 /2))^5 (1−2x^2 )^3 (1−((9x^2 )/2))^3 )/(22x^2 )) = lim_(x→0) ((1−(1−((5x^2 )/2))(1−6x^2 )(1−((27x^2 )/2)))/(22x^2 )) = lim_(x→0) ((1−(1−((5/2)+6+((27)/2))x^2 +o(x^2 )))/(22x^2 )) = lim_(x→0) ((((5/2)+6+((27)/2))x^2 +o(x^2 ))/(22x^2 )) = ((44)/(2(22))) = 1 (B⊚B)
$$\mathrm{cos}\:{x}\:=\:\sqrt{\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} {x}}\:\approx\:\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\right)^{\mathrm{5}} \left(\mathrm{1}−\mathrm{2}{x}^{\mathrm{2}} \right)^{\mathrm{3}} \left(\mathrm{1}−\frac{\mathrm{9}{x}^{\mathrm{2}} }{\mathrm{2}}\right)^{\mathrm{3}} }{\mathrm{22}{x}^{\mathrm{2}} }\:= \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\left(\mathrm{1}−\frac{\mathrm{5}{x}^{\mathrm{2}} }{\mathrm{2}}\right)\left(\mathrm{1}−\mathrm{6}{x}^{\mathrm{2}} \right)\left(\mathrm{1}−\frac{\mathrm{27}{x}^{\mathrm{2}} }{\mathrm{2}}\right)}{\mathrm{22}{x}^{\mathrm{2}} }\:= \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}−\left(\mathrm{1}−\left(\frac{\mathrm{5}}{\mathrm{2}}+\mathrm{6}+\frac{\mathrm{27}}{\mathrm{2}}\right){x}^{\mathrm{2}} +{o}\left({x}^{\mathrm{2}} \right)\right)}{\mathrm{22}{x}^{\mathrm{2}} }\:= \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left(\frac{\mathrm{5}}{\mathrm{2}}+\mathrm{6}+\frac{\mathrm{27}}{\mathrm{2}}\right){x}^{\mathrm{2}} +{o}\left({x}^{\mathrm{2}} \right)}{\mathrm{22}{x}^{\mathrm{2}} }\:=\:\frac{\mathrm{44}}{\mathrm{2}\left(\mathrm{22}\right)}\:=\:\mathrm{1} \\ $$$$\left({B}\circledcirc{B}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *