Question Number 104667 by bobhans last updated on 23/Jul/20
![lim_(x→0) [(3^(πx) /(tan x)) − ((cos^2 x)/(sin x))] ?](https://www.tinkutara.com/question/Q104667.png)
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left[\frac{\mathrm{3}^{\pi{x}} }{\mathrm{tan}\:{x}}\:−\:\frac{\mathrm{cos}\:^{\mathrm{2}} {x}}{\mathrm{sin}\:{x}}\right]\:? \\ $$
Answered by bramlex last updated on 23/Jul/20
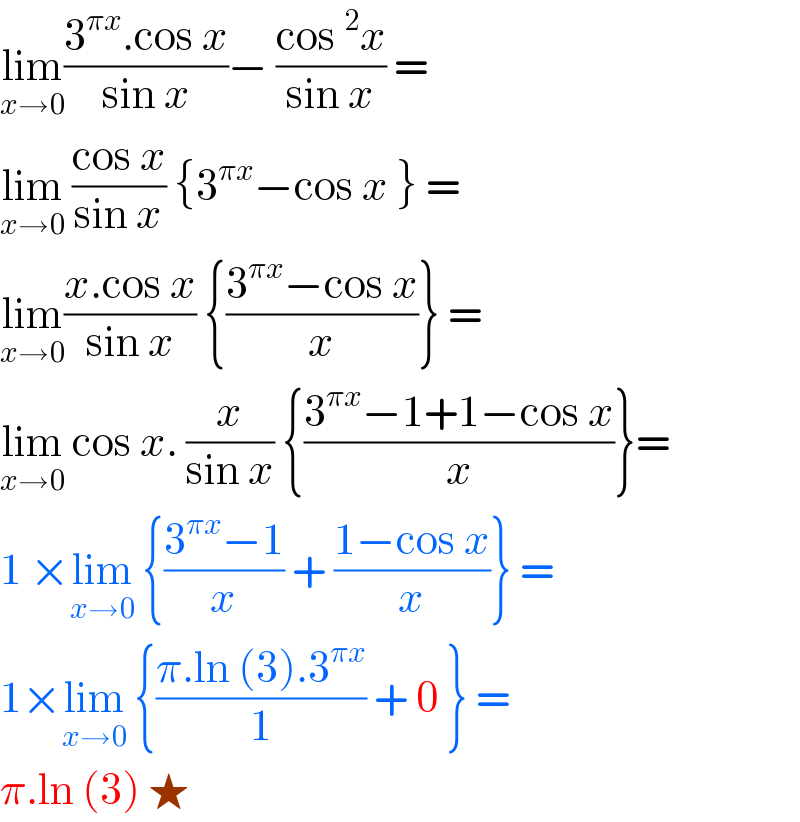
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{3}^{\pi{x}} .\mathrm{cos}\:{x}}{\mathrm{sin}\:{x}}−\:\frac{\mathrm{cos}\:^{\mathrm{2}} {x}}{\mathrm{sin}\:{x}}\:= \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:{x}}{\mathrm{sin}\:{x}}\:\left\{\mathrm{3}^{\pi{x}} −\mathrm{cos}\:{x}\:\right\}\:=\: \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{x}.\mathrm{cos}\:{x}}{\mathrm{sin}\:{x}}\:\left\{\frac{\mathrm{3}^{\pi{x}} −\mathrm{cos}\:{x}}{{x}}\right\}\:= \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{cos}\:{x}.\:\frac{{x}}{\mathrm{sin}\:{x}}\:\left\{\frac{\mathrm{3}^{\pi{x}} −\mathrm{1}+\mathrm{1}−\mathrm{cos}\:{x}}{{x}}\right\}= \\ $$$$\mathrm{1}\:×\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left\{\frac{\mathrm{3}^{\pi{x}} −\mathrm{1}}{{x}}\:+\:\frac{\mathrm{1}−\mathrm{cos}\:{x}}{{x}}\right\}\:= \\ $$$$\mathrm{1}×\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left\{\frac{\pi.\mathrm{ln}\:\left(\mathrm{3}\right).\mathrm{3}^{\pi{x}} }{\mathrm{1}}\:+\:\mathrm{0}\:\right\}\:= \\ $$$$\pi.\mathrm{ln}\:\left(\mathrm{3}\right)\:\bigstar\: \\ $$
Commented by bobhans last updated on 23/Jul/20

$${very}\:{nice}\:!\:\overset{{o}} {\smile}\:! \\ $$
