Menu Close

lim-x-0-3-x-5-x-6-x-3-1-x-

Tinku Tara 0 Comments
Pin




Question Number 147169 by mathdanisur last updated on 18/Jul/21
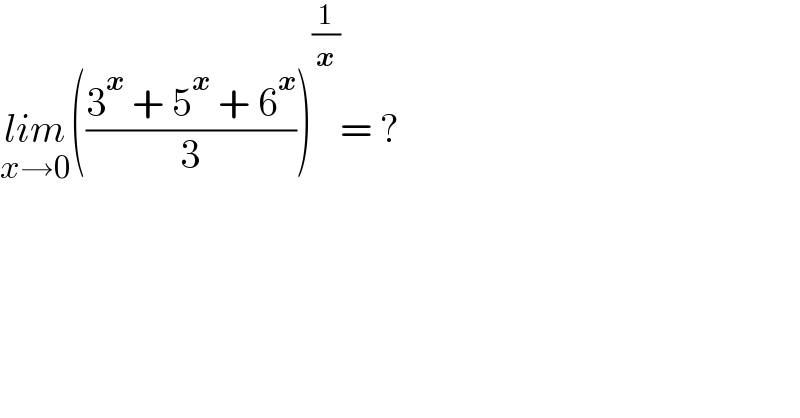
lim_(x→0) (((3^x + 5^x + 6^x )/3))^(1/x) = ?
$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\left(\frac{\mathrm{3}^{\boldsymbol{{x}}} \:+\:\mathrm{5}^{\boldsymbol{{x}}} \:+\:\mathrm{6}^{\boldsymbol{{x}}} }{\mathrm{3}}\right)^{\frac{\mathrm{1}}{\boldsymbol{{x}}}} =\:? \\ $$
Commented by gsk2684 last updated on 18/Jul/21
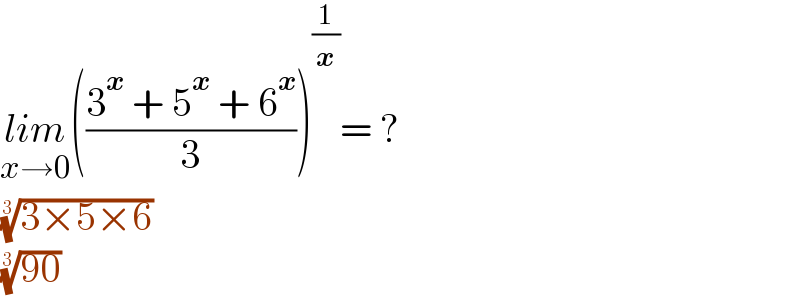
lim_(x→0) (((3^x + 5^x + 6^x )/3))^(1/x) = ? ((3×5×6))^(1/3) ((90))^(1/3)
$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\left(\frac{\mathrm{3}^{\boldsymbol{{x}}} \:+\:\mathrm{5}^{\boldsymbol{{x}}} \:+\:\mathrm{6}^{\boldsymbol{{x}}} }{\mathrm{3}}\right)^{\frac{\mathrm{1}}{\boldsymbol{{x}}}} =\:? \\ $$$$\sqrt[{\mathrm{3}}]{\mathrm{3}×\mathrm{5}×\mathrm{6}} \\ $$$$\sqrt[{\mathrm{3}}]{\mathrm{90}} \\ $$
Commented by mathdanisur last updated on 19/Jul/21
Thankyou Ser How did you get the answer is there a formula?
$${Thankyou}\:{Ser} \\ $$$${How}\:{did}\:{you}\:{get}\:{the}\:{answer}\:{is}\:{there} \\ $$$${a}\:{formula}? \\ $$
Commented by gsk2684 last updated on 21/Jul/21
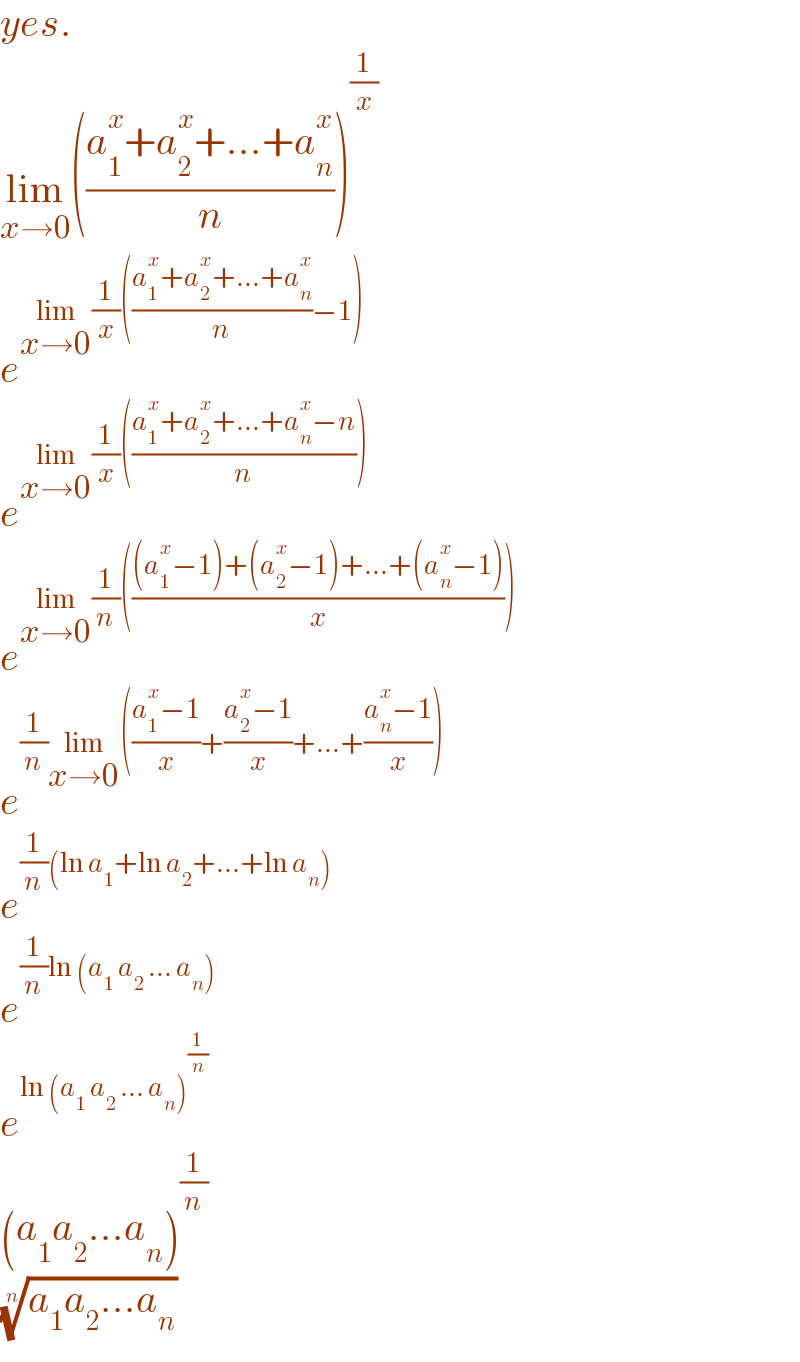
yes. lim_(x→0) (((a_1 ^x +a_2 ^x +...+a_n ^x )/n))^(1/x) e^(lim_(x→0) (1/x)(((a_1 ^x +a_2 ^x +...+a_n ^x )/n)−1)) e^(lim_(x→0) (1/x)(((a_1 ^x +a_2 ^x +...+a_n ^x −n)/n))) e^(lim_(x→0) (1/n)((((a_1 ^x −1)+(a_2 ^x −1)+...+(a_n ^x −1))/x))) e^((1/n)lim_(x→0) (((a_1 ^x −1)/x)+((a_2 ^x −1)/x)+...+((a_n ^x −1)/x))) e^((1/n)(ln a_1 +ln a_2 +...+ln a_n )) e^((1/n)ln (a_1 a_2 ... a_n )) e^(ln (a_1 a_2 ... a_n )^(1/n) ) (a_1 a_2 ...a_n )^(1/n) ((a_1 a_2 ...a_n ))^(1/n)
$${yes}. \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{{a}_{\mathrm{1}} ^{{x}} +{a}_{\mathrm{2}} ^{{x}} +…+{a}_{{n}} ^{{x}} }{{n}}\right)^{\frac{\mathrm{1}}{{x}}} \\ $$$${e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{{x}}\left(\frac{{a}_{\mathrm{1}} ^{{x}} +{a}_{\mathrm{2}} ^{{x}} +…+{a}_{{n}} ^{{x}} }{{n}}−\mathrm{1}\right)} \\ $$$${e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{{x}}\left(\frac{{a}_{\mathrm{1}} ^{{x}} +{a}_{\mathrm{2}} ^{{x}} +…+{a}_{{n}} ^{{x}} −{n}}{{n}}\right)} \\ $$$${e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{{n}}\left(\frac{\left({a}_{\mathrm{1}} ^{{x}} −\mathrm{1}\right)+\left({a}_{\mathrm{2}} ^{{x}} −\mathrm{1}\right)+…+\left({a}_{{n}} ^{{x}} −\mathrm{1}\right)}{{x}}\right)} \\ $$$${e}^{\frac{\mathrm{1}}{{n}}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{{a}_{\mathrm{1}} ^{{x}} −\mathrm{1}}{{x}}+\frac{{a}_{\mathrm{2}} ^{{x}} −\mathrm{1}}{{x}}+…+\frac{{a}_{{n}} ^{{x}} −\mathrm{1}}{{x}}\right)} \\ $$$${e}^{\frac{\mathrm{1}}{{n}}\left(\mathrm{ln}\:{a}_{\mathrm{1}} +\mathrm{ln}\:{a}_{\mathrm{2}} +…+\mathrm{ln}\:{a}_{{n}} \right)} \\ $$$${e}^{\frac{\mathrm{1}}{{n}}\mathrm{ln}\:\left({a}_{\mathrm{1}} \:{a}_{\mathrm{2}} \:…\:{a}_{{n}} \right)} \\ $$$${e}^{\mathrm{ln}\:\left({a}_{\mathrm{1}} \:{a}_{\mathrm{2}} \:…\:{a}_{{n}} \right)^{\frac{\mathrm{1}}{{n}}} } \\ $$$$\left({a}_{\mathrm{1}} {a}_{\mathrm{2}} …{a}_{{n}} \right)^{\frac{\mathrm{1}}{{n}}} \\ $$$$\sqrt[{{n}}]{{a}_{\mathrm{1}} {a}_{\mathrm{2}} …{a}_{{n}} } \\ $$
Commented by mathdanisur last updated on 21/Jul/21
Thank you Ser, cool
$${Thank}\:{you}\:{Ser},\:{cool} \\ $$
Answered by mathmax by abdo last updated on 18/Jul/21
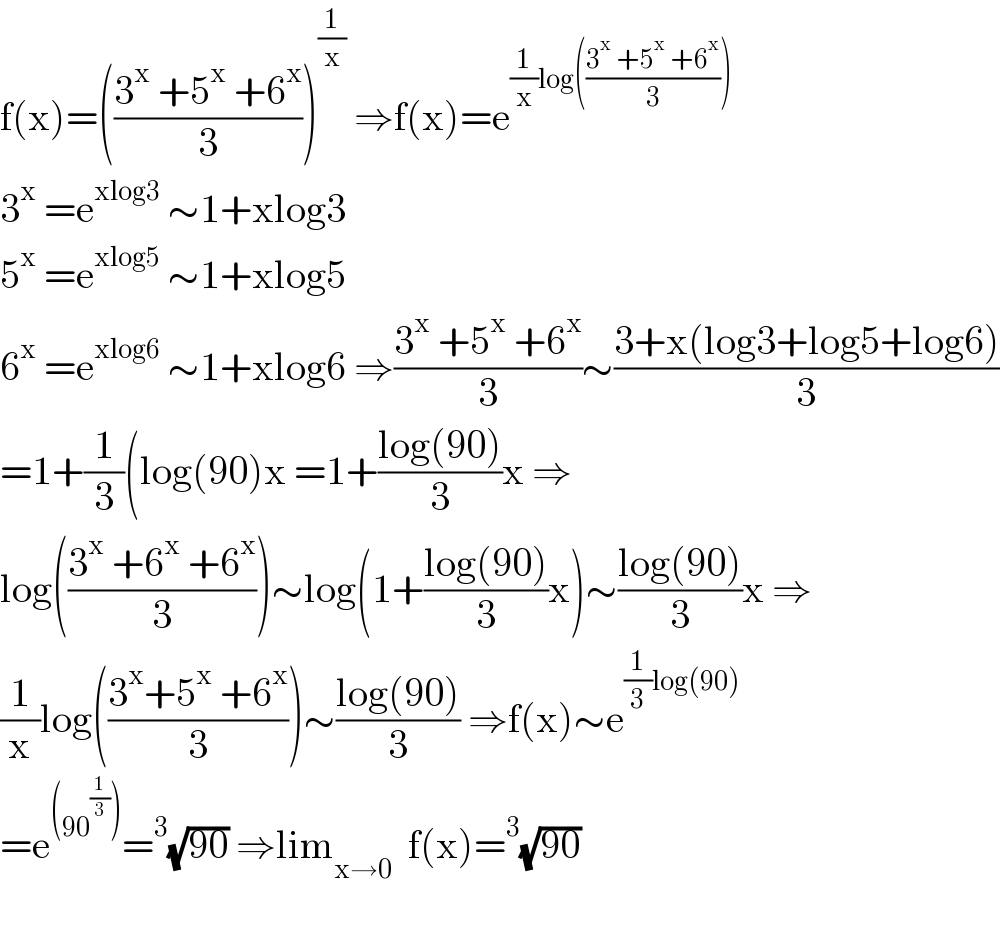
f(x)=(((3^x +5^x +6^x )/3))^(1/x) ⇒f(x)=e^((1/x)log(((3^(x ) +5^x +6^x )/3))) 3^x =e^(xlog3) ∼1+xlog3 5^x =e^(xlog5) ∼1+xlog5 6^x =e^(xlog6) ∼1+xlog6 ⇒((3^x +5^x +6^x )/3)∼((3+x(log3+log5+log6))/3) =1+(1/3)(log(90)x =1+((log(90))/3)x ⇒ log(((3^x +6^x +6^x )/3))∼log(1+((log(90))/3)x)∼((log(90))/3)x ⇒ (1/x)log(((3^x +5^x +6^x )/3))∼((log(90))/3) ⇒f(x)∼e^((1/3)log(90)) =e^((90^(1/3) )) =^3 (√(90)) ⇒lim_(x→0) f(x)=^3 (√(90))
$$\mathrm{f}\left(\mathrm{x}\right)=\left(\frac{\mathrm{3}^{\mathrm{x}} \:+\mathrm{5}^{\mathrm{x}} \:+\mathrm{6}^{\mathrm{x}} }{\mathrm{3}}\right)^{\frac{\mathrm{1}}{\mathrm{x}}} \:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)=\mathrm{e}^{\frac{\mathrm{1}}{\mathrm{x}}\mathrm{log}\left(\frac{\mathrm{3}^{\mathrm{x}\:} +\mathrm{5}^{\mathrm{x}} \:+\mathrm{6}^{\mathrm{x}} }{\mathrm{3}}\right)} \\ $$$$\mathrm{3}^{\mathrm{x}} \:=\mathrm{e}^{\mathrm{xlog3}} \:\sim\mathrm{1}+\mathrm{xlog3} \\ $$$$\mathrm{5}^{\mathrm{x}} \:=\mathrm{e}^{\mathrm{xlog5}} \:\sim\mathrm{1}+\mathrm{xlog5} \\ $$$$\mathrm{6}^{\mathrm{x}} \:=\mathrm{e}^{\mathrm{xlog6}} \:\sim\mathrm{1}+\mathrm{xlog6}\:\Rightarrow\frac{\mathrm{3}^{\mathrm{x}} \:+\mathrm{5}^{\mathrm{x}} \:+\mathrm{6}^{\mathrm{x}} }{\mathrm{3}}\sim\frac{\mathrm{3}+\mathrm{x}\left(\mathrm{log3}+\mathrm{log5}+\mathrm{log6}\right)}{\mathrm{3}} \\ $$$$=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{log}\left(\mathrm{90}\right)\mathrm{x}\:=\mathrm{1}+\frac{\mathrm{log}\left(\mathrm{90}\right)}{\mathrm{3}}\mathrm{x}\:\Rightarrow\right. \\ $$$$\mathrm{log}\left(\frac{\mathrm{3}^{\mathrm{x}} \:+\mathrm{6}^{\mathrm{x}} \:+\mathrm{6}^{\mathrm{x}} }{\mathrm{3}}\right)\sim\mathrm{log}\left(\mathrm{1}+\frac{\mathrm{log}\left(\mathrm{90}\right)}{\mathrm{3}}\mathrm{x}\right)\sim\frac{\mathrm{log}\left(\mathrm{90}\right)}{\mathrm{3}}\mathrm{x}\:\Rightarrow \\ $$$$\frac{\mathrm{1}}{\mathrm{x}}\mathrm{log}\left(\frac{\mathrm{3}^{\mathrm{x}} +\mathrm{5}^{\mathrm{x}} \:+\mathrm{6}^{\mathrm{x}} }{\mathrm{3}}\right)\sim\frac{\mathrm{log}\left(\mathrm{90}\right)}{\mathrm{3}}\:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)\sim\mathrm{e}^{\frac{\mathrm{1}}{\mathrm{3}}\mathrm{log}\left(\mathrm{90}\right)} \\ $$$$=\mathrm{e}^{\left(\mathrm{90}^{\frac{\mathrm{1}}{\mathrm{3}}} \right)} =^{\mathrm{3}} \sqrt{\mathrm{90}}\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \:\:\mathrm{f}\left(\mathrm{x}\right)=^{\mathrm{3}} \sqrt{\mathrm{90}} \\ $$$$ \\ $$
Commented by mathdanisur last updated on 19/Jul/21
thank you Ser
$${thank}\:{you}\:{Ser} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *