Question Number 111914 by bemath last updated on 05/Sep/20
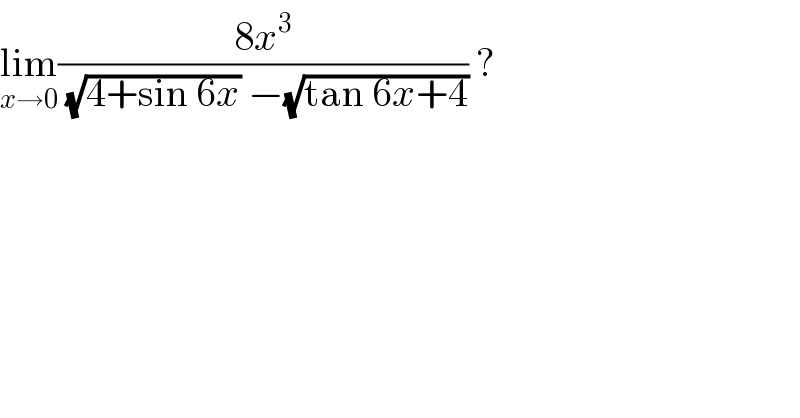
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{8}{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{4}+\mathrm{sin}\:\mathrm{6}{x}}\:−\sqrt{\mathrm{tan}\:\mathrm{6}{x}+\mathrm{4}}}\:? \\ $$
Answered by bobhans last updated on 05/Sep/20
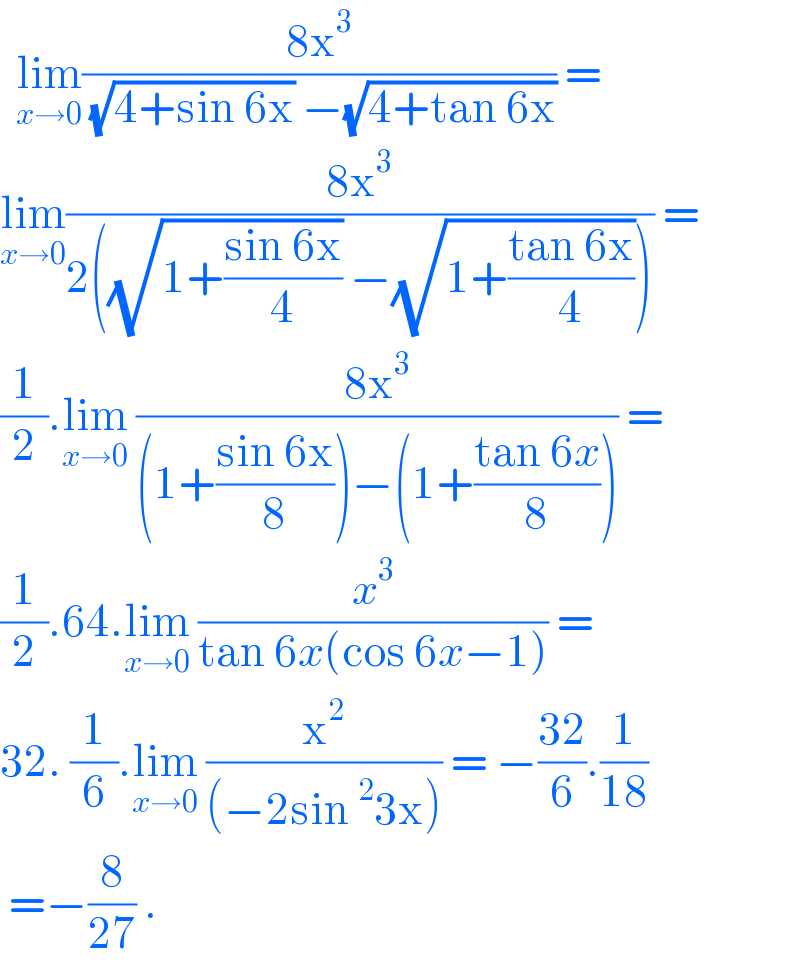
$$\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{8x}^{\mathrm{3}} }{\:\sqrt{\mathrm{4}+\mathrm{sin}\:\mathrm{6x}}\:−\sqrt{\mathrm{4}+\mathrm{tan}\:\mathrm{6x}}}\:=\: \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{8x}^{\mathrm{3}} }{\mathrm{2}\left(\sqrt{\mathrm{1}+\frac{\mathrm{sin}\:\mathrm{6x}}{\mathrm{4}}}\:−\sqrt{\mathrm{1}+\frac{\mathrm{tan}\:\mathrm{6x}}{\mathrm{4}}}\right)}\:= \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}.\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{8x}^{\mathrm{3}} }{\left(\mathrm{1}+\frac{\mathrm{sin}\:\mathrm{6x}}{\mathrm{8}}\right)−\left(\mathrm{1}+\frac{\mathrm{tan}\:\mathrm{6}{x}}{\mathrm{8}}\right)}\:= \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}.\mathrm{64}.\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{3}} }{\mathrm{tan}\:\mathrm{6}{x}\left(\mathrm{cos}\:\mathrm{6}{x}−\mathrm{1}\right)}\:= \\ $$$$\mathrm{32}.\:\frac{\mathrm{1}}{\mathrm{6}}.\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{x}^{\mathrm{2}} }{\left(−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{3x}\right)}\:=\:−\frac{\mathrm{32}}{\mathrm{6}}.\frac{\mathrm{1}}{\mathrm{18}}\: \\ $$$$\:=−\frac{\mathrm{8}}{\mathrm{27}}\:.\: \\ $$
