Menu Close

lim-x-0-arctan-x-arcsin-x-sin-3-x-

Tinku Tara 0 Comments
Pin




Question Number 147738 by liberty last updated on 23/Jul/21
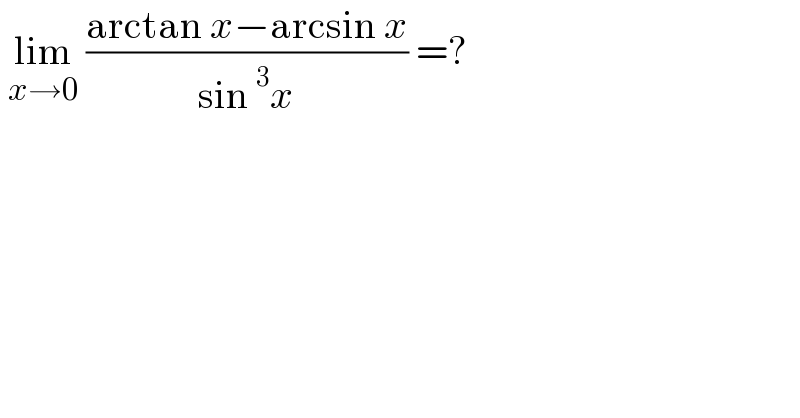
lim_(x→0) ((arctan x−arcsin x)/(sin^3 x)) =?
$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{arctan}\:{x}−\mathrm{arcsin}\:{x}}{\mathrm{sin}\:^{\mathrm{3}} {x}}\:=? \\ $$
Answered by gsk2684 last updated on 23/Jul/21
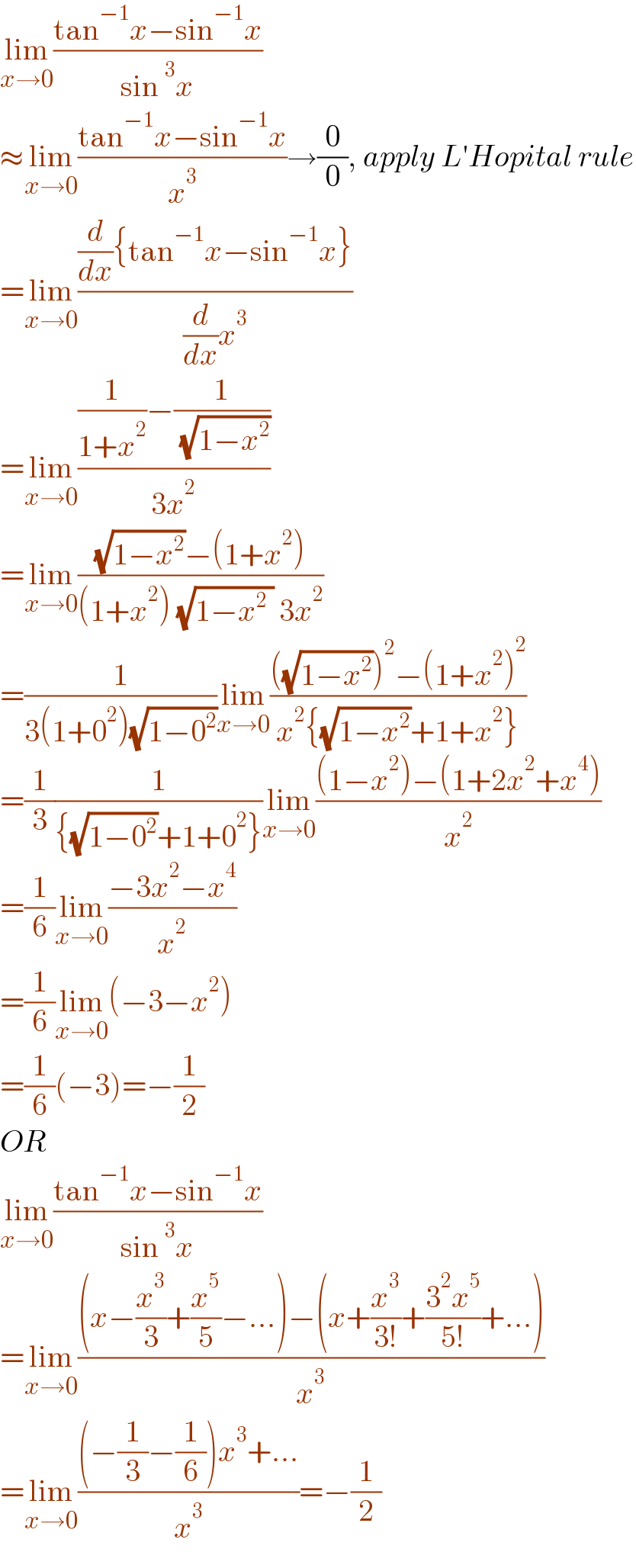
lim_(x→0) ((tan^(−1) x−sin^(−1) x)/(sin^3 x)) ≈lim_(x→0) ((tan^(−1) x−sin^(−1) x)/x^3 )→(0/0), apply L′Hopital rule =lim_(x→0) (((d/dx){tan^(−1) x−sin^(−1) x})/((d/dx)x^3 )) =lim_(x→0) (((1/(1+x^2 ))−(1/( (√(1−x^2 )))))/(3x^2 )) =lim_(x→0) (((√(1−x^2 ))−(1+x^2 ))/((1+x^2 ) (√(1−x^2 )) 3x^2 )) =(1/(3(1+0^2 )(√(1−0^2 ))))lim_(x→0) ((((√(1−x^2 )))^2 −(1+x^2 )^2 )/(x^2 {(√(1−x^2 ))+1+x^2 })) =(1/3)(1/({(√(1−0^2 ))+1+0^2 }))lim_(x→0) (((1−x^2 )−(1+2x^2 +x^4 ))/x^2 ) =(1/6)lim_(x→0) ((−3x^2 −x^4 )/x^2 ) =(1/6)lim_(x→0) (−3−x^2 ) =(1/6)(−3)=−(1/2) OR lim_(x→0) ((tan^(−1) x−sin^(−1) x)/(sin^3 x)) =lim_(x→0) (((x−(x^3 /3)+(x^5 /5)−...)−(x+(x^3 /(3!))+((3^2 x^5 )/(5!))+...))/x^3 ) =lim_(x→0) (((−(1/3)−(1/6))x^3 +...)/x^3 )=−(1/2)
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{tan}^{−\mathrm{1}} {x}−\mathrm{sin}^{−\mathrm{1}} {x}}{\mathrm{sin}\:^{\mathrm{3}} {x}} \\ $$$$\approx\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{tan}^{−\mathrm{1}} {x}−\mathrm{sin}^{−\mathrm{1}} {x}}{{x}^{\mathrm{3}} }\rightarrow\frac{\mathrm{0}}{\mathrm{0}},\:{apply}\:{L}'{Hopital}\:{rule} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\frac{{d}}{{dx}}\left\{\mathrm{tan}^{−\mathrm{1}} {x}−\mathrm{sin}^{−\mathrm{1}} {x}\right\}}{\frac{{d}}{{dx}}{x}^{\mathrm{3}} } \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }−\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}}{\mathrm{3}{x}^{\mathrm{2}} } \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }−\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} \:}\:\mathrm{3}{x}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}\left(\mathrm{1}+\mathrm{0}^{\mathrm{2}} \right)\sqrt{\mathrm{1}−\mathrm{0}^{\mathrm{2}} }}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left(\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\right)^{\mathrm{2}} −\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{{x}^{\mathrm{2}} \left\{\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }+\mathrm{1}+{x}^{\mathrm{2}} \right\}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\frac{\mathrm{1}}{\left\{\sqrt{\mathrm{1}−\mathrm{0}^{\mathrm{2}} }+\mathrm{1}+\mathrm{0}^{\mathrm{2}} \right\}}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)−\left(\mathrm{1}+\mathrm{2}{x}^{\mathrm{2}} +{x}^{\mathrm{4}} \right)}{{x}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{6}}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\mathrm{3}{x}^{\mathrm{2}} −{x}^{\mathrm{4}} }{{x}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{6}}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(−\mathrm{3}−{x}^{\mathrm{2}} \right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{6}}\left(−\mathrm{3}\right)=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${OR} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{tan}^{−\mathrm{1}} {x}−\mathrm{sin}^{−\mathrm{1}} {x}}{\mathrm{sin}\:^{\mathrm{3}} {x}} \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left({x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}}−…\right)−\left({x}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+\frac{\mathrm{3}^{\mathrm{2}} {x}^{\mathrm{5}} }{\mathrm{5}!}+…\right)}{{x}^{\mathrm{3}} } \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left(−\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{6}}\right){x}^{\mathrm{3}} +…}{{x}^{\mathrm{3}} }=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Answered by mathmax by abdo last updated on 23/Jul/21
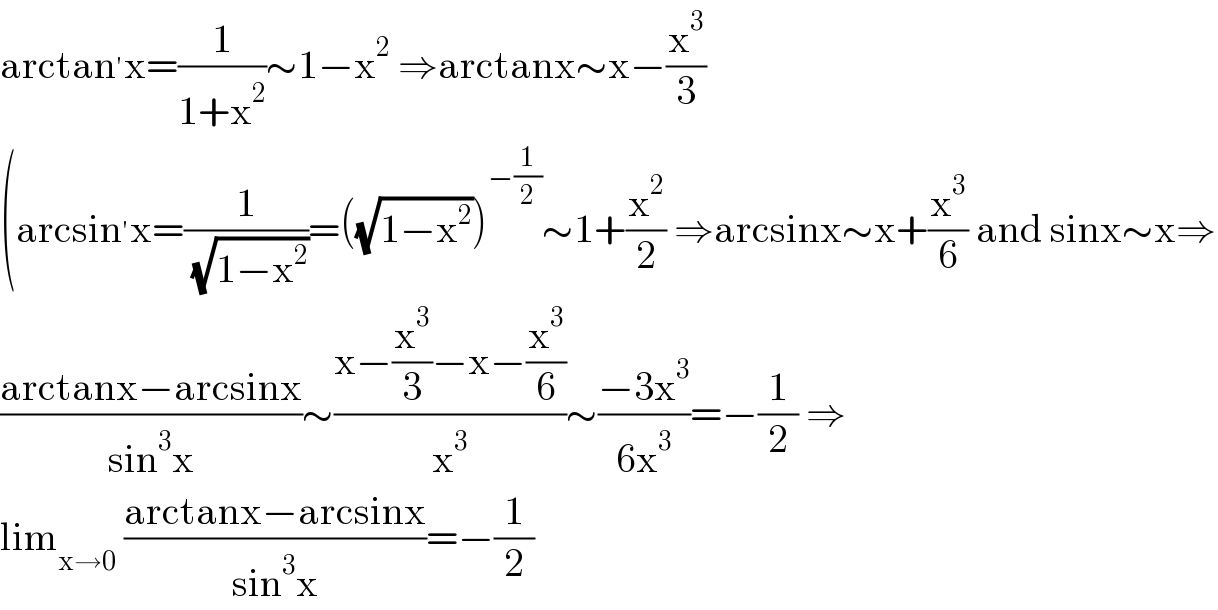
arctan^′ x=(1/(1+x^2 ))∼1−x^2 ⇒arctanx∼x−(x^3 /3) (arcsin^′ x=(1/( (√(1−x^2 ))))=((√(1−x^2 )))^(−(1/2)) ∼1+(x^2 /2) ⇒arcsinx∼x+(x^3 /6) and sinx∼x⇒ ((arctanx−arcsinx)/(sin^3 x))∼((x−(x^3 /3)−x−(x^3 /6))/x^3 )∼((−3x^3 )/(6x^3 ))=−(1/2) ⇒ lim_(x→0) ((arctanx−arcsinx)/(sin^3 x))=−(1/2)
$$\mathrm{arctan}^{'} \mathrm{x}=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\sim\mathrm{1}−\mathrm{x}^{\mathrm{2}} \:\Rightarrow\mathrm{arctanx}\sim\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}} \\ $$$$\left(\mathrm{arcsin}^{'} \mathrm{x}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}=\left(\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \sim\mathrm{1}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}\:\Rightarrow\mathrm{arcsinx}\sim\mathrm{x}+\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}\:\mathrm{and}\:\mathrm{sinx}\sim\mathrm{x}\Rightarrow\right. \\ $$$$\frac{\mathrm{arctanx}−\mathrm{arcsinx}}{\mathrm{sin}^{\mathrm{3}} \mathrm{x}}\sim\frac{\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}−\mathrm{x}−\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{6}}}{\mathrm{x}^{\mathrm{3}} }\sim\frac{−\mathrm{3x}^{\mathrm{3}} }{\mathrm{6x}^{\mathrm{3}} }=−\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow \\ $$$$\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \:\frac{\mathrm{arctanx}−\mathrm{arcsinx}}{\mathrm{sin}^{\mathrm{3}} \mathrm{x}}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *