Question Number 90060 by jagoll last updated on 21/Apr/20
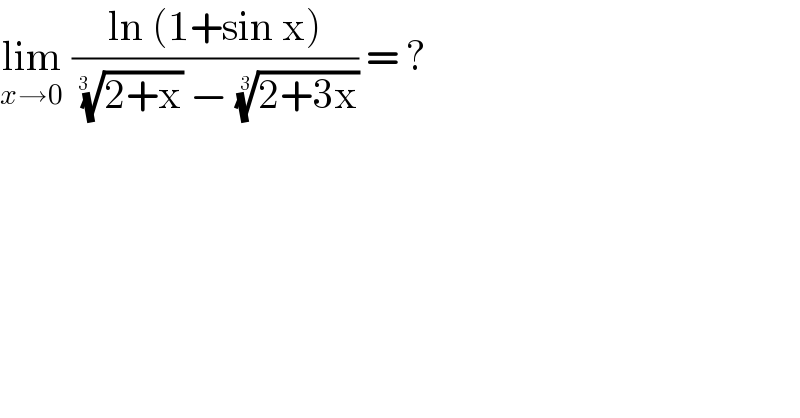
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{ln}\:\left(\mathrm{1}+\mathrm{sin}\:\mathrm{x}\right)}{\:\sqrt[{\mathrm{3}\:\:}]{\mathrm{2}+\mathrm{x}}\:−\:\sqrt[{\mathrm{3}}]{\mathrm{2}+\mathrm{3x}}}\:=\:? \\ $$
Commented by john santu last updated on 21/Apr/20
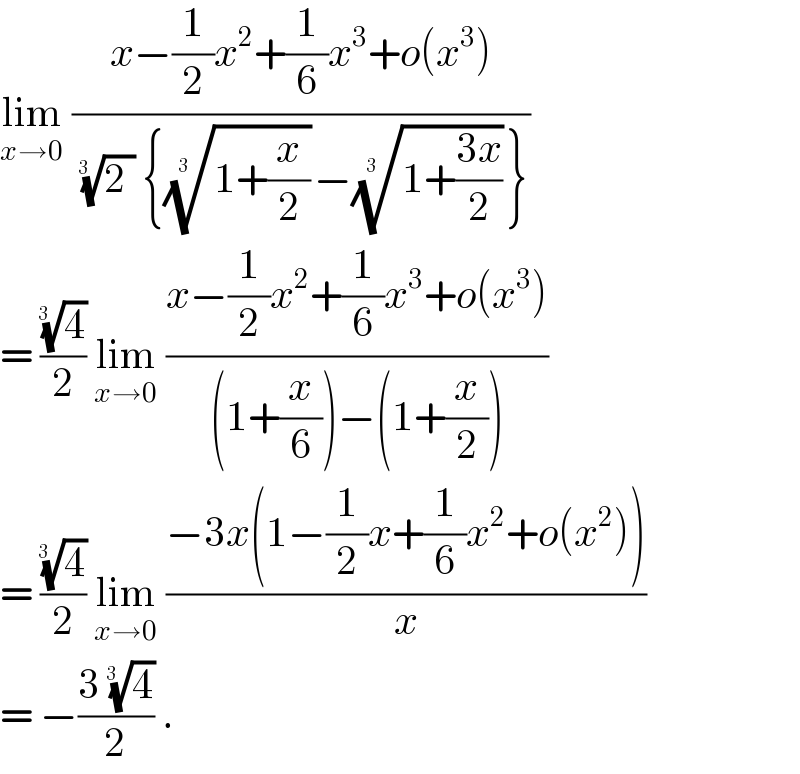
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}−\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{3}} +{o}\left({x}^{\mathrm{3}} \right)}{\:\sqrt[{\mathrm{3}\:\:}]{\mathrm{2}\:}\:\left\{\sqrt[{\mathrm{3}\:\:}]{\mathrm{1}+\frac{{x}}{\mathrm{2}}}−\sqrt[{\mathrm{3}\:\:}]{\mathrm{1}+\frac{\mathrm{3}{x}}{\mathrm{2}}}\right\}} \\ $$$$=\:\frac{\sqrt[{\mathrm{3}\:\:}]{\mathrm{4}}}{\mathrm{2}}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}−\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{3}} +{o}\left({x}^{\mathrm{3}} \right)}{\left(\mathrm{1}+\frac{{x}}{\mathrm{6}}\right)−\left(\mathrm{1}+\frac{{x}}{\mathrm{2}}\right)} \\ $$$$=\:\frac{\sqrt[{\mathrm{3}\:\:}]{\mathrm{4}}}{\mathrm{2}}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{−\mathrm{3}{x}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}{x}+\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{2}} +{o}\left({x}^{\mathrm{2}} \right)\right)}{{x}} \\ $$$$=\:−\frac{\mathrm{3}\:\sqrt[{\mathrm{3}\:\:}]{\mathrm{4}}}{\mathrm{2}}\:.\: \\ $$
