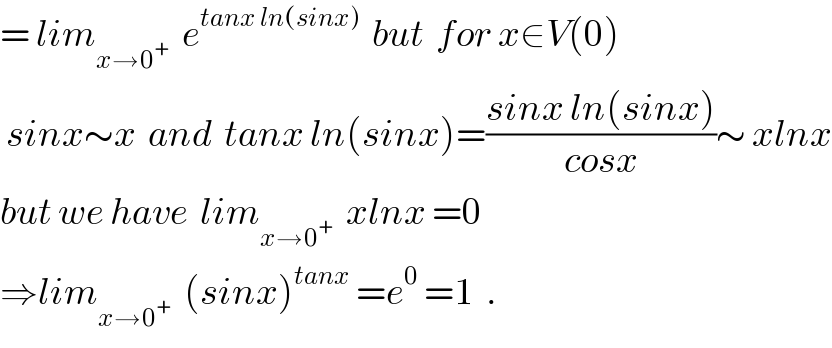lim-x-0-sinx-tanx-

Question Number 28088 by tawa tawa last updated on 20/Jan/18

\lim_{x \to 0^{+}} {(sinx)}^{(tanx)}

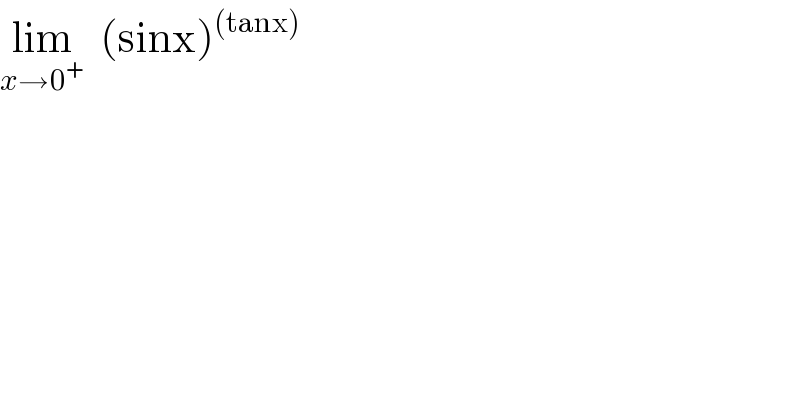
Commented by çhëý böý last updated on 20/Jan/18

\lim_{x \to 0^{+}} e^{\ln \sin x^{t a n x}}

e^{\lim_{x \to 0^{+}} \tan x \ln x}

l^{'} h o p i t a l

e^{\lim_{x \to 0 +} - \frac{\frac{c o s x}{s i n x}}{\frac{{s e c}^{2} x}{{t a n}^{2} x}}}

s i m p l i f y

e^{\lim_{x \to 0^{+}} - \frac{s i n x}{{c o s}^{3} x}}

e^{\lim_{x \to 0^{+}} - \frac{s i n (0)}{c o s {(0)}^{3}} = \frac{- 0}{1} = 0}

e^{0} = 1

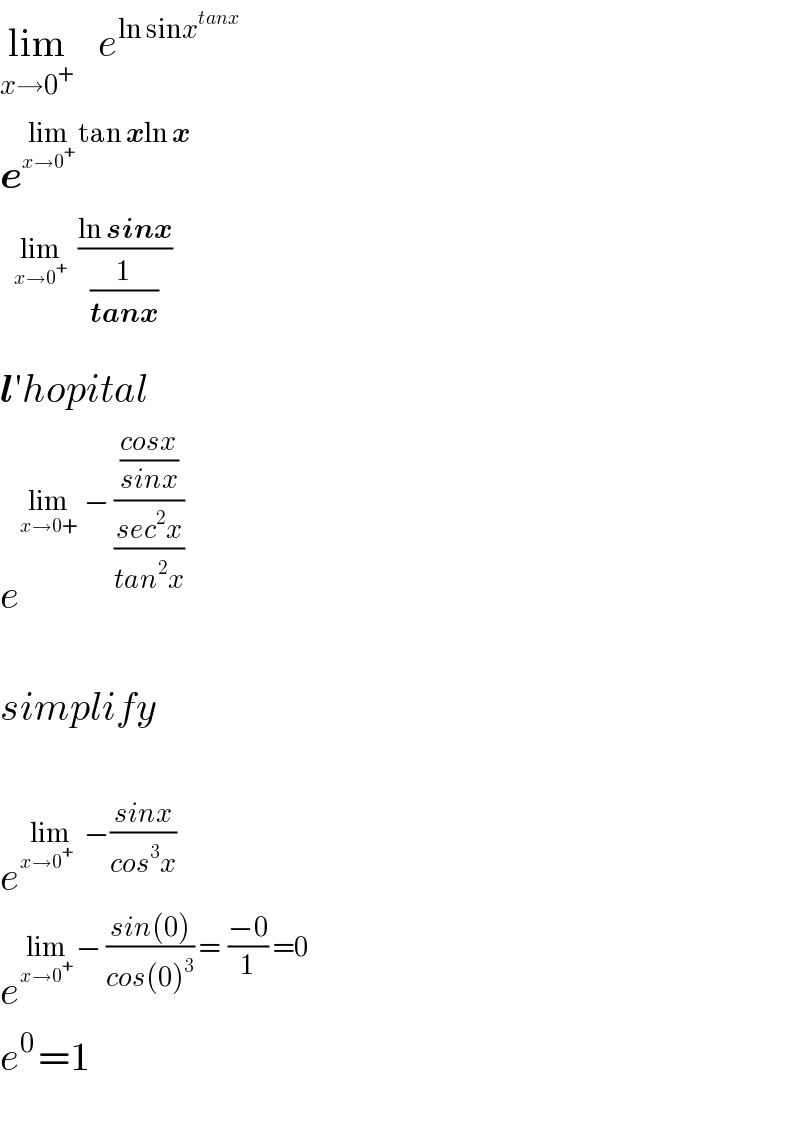
Commented by abdo imad last updated on 20/Jan/18

x \to 0^{+} m e a n x i s m o s t n e a r f r o m 0 a n d x > 0

x \to 0 - m e a n t h a t x i s m o s t n e a r f r o m 0 a n d x < 0 .

Commented by tawa tawa last updated on 20/Jan/18

God bless you sir

Commented by tawa tawa last updated on 20/Jan/18

please sir . what is the meaning of 0^{+} or 0^{-}

i mean the one at the limit .

Commented by çhëý böý last updated on 20/Jan/18

i t m e a n t h e l i m i t {c a n}^{^{'}} t a t 0 s o 0^{+} m e a n

u a r a p p r o c h i n g f r o m r i g h t [p o s i t i v e] s i d e

Commented by abdo imad last updated on 20/Jan/18

= {l i m}_{x \to 0^{+}} e^{t a n x l n (s i n x)} b u t f o r x \in V (0)

s i n x \sim x a n d t a n x l n (s i n x) = \frac{s i n x l n (s i n x)}{c o s x} \sim x l n x

b u t w e h a v e {l i m}_{x \to 0^{+}} x l n x = 0

\Rightarrow {l i m}_{x \to 0^{+}} {(s i n x)}^{t a n x} = e^{0} = 1 .