Question Number 149251 by john_santu last updated on 04/Aug/21
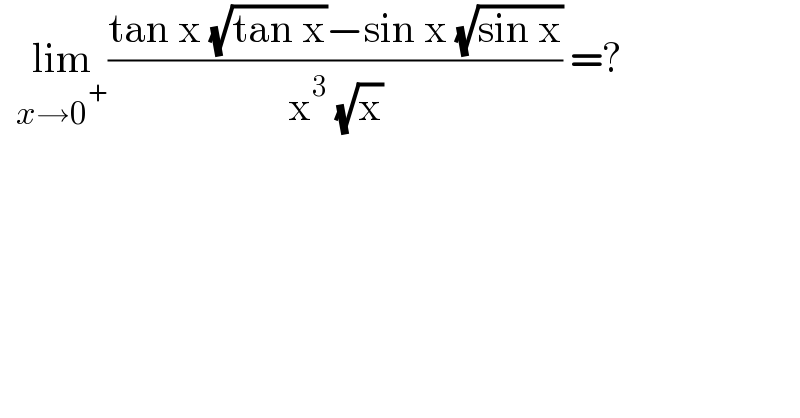
$$\:\:\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\frac{\mathrm{tan}\:\mathrm{x}\:\sqrt{\mathrm{tan}\:\mathrm{x}}−\mathrm{sin}\:\mathrm{x}\:\sqrt{\mathrm{sin}\:\mathrm{x}}}{\mathrm{x}^{\mathrm{3}} \:\sqrt{\mathrm{x}}}\:=? \\ $$$$ \\ $$
Answered by dumitrel last updated on 04/Aug/21
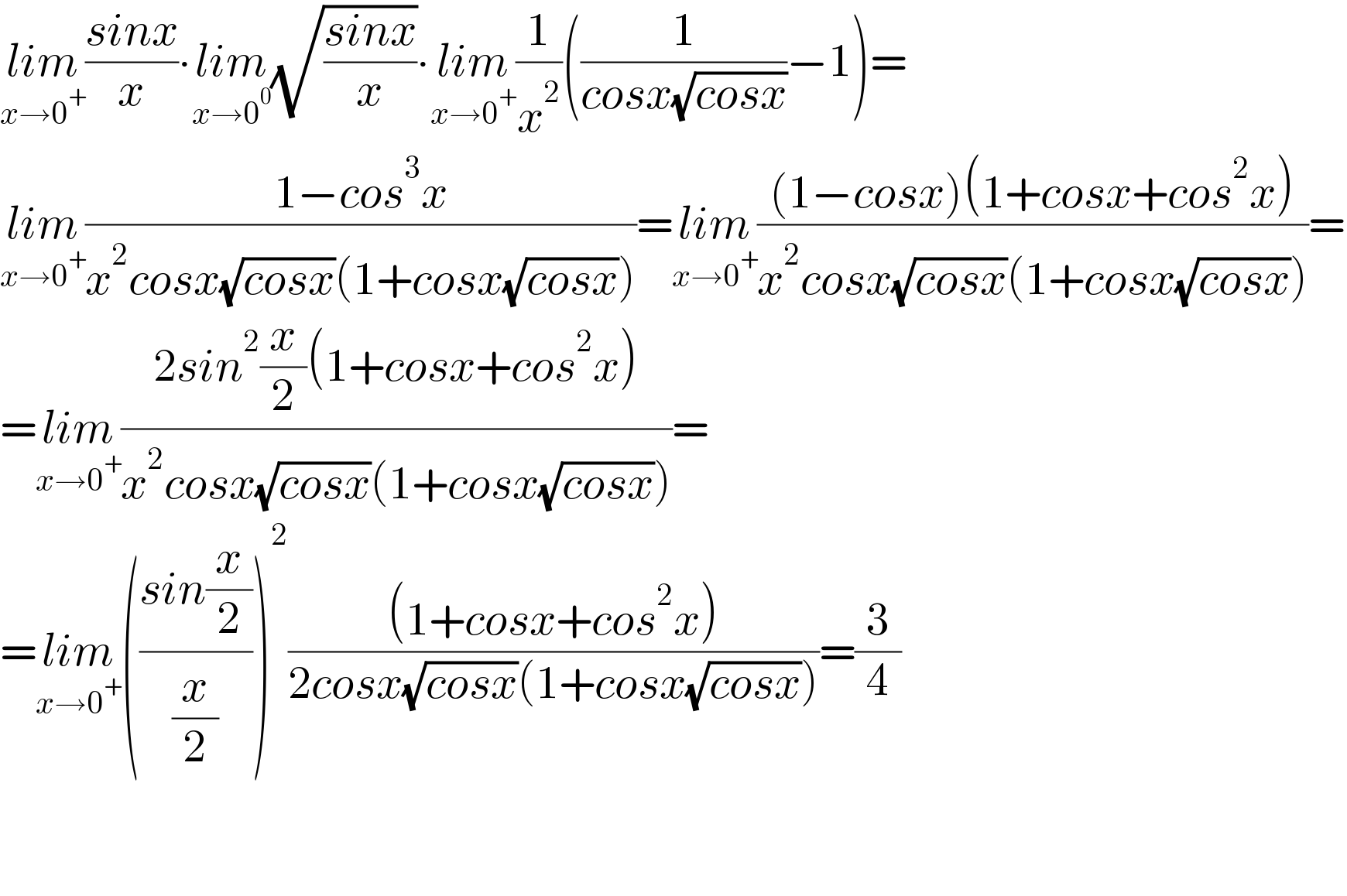
$$\underset{{x}\rightarrow\mathrm{0}^{+} } {{lim}}\frac{{sinx}}{{x}}\centerdot\underset{{x}\rightarrow\mathrm{0}^{\mathrm{0}} } {{lim}}\sqrt{\frac{{sinx}}{{x}}}\centerdot\underset{{x}\rightarrow\mathrm{0}^{+} } {{lim}}\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\left(\frac{\mathrm{1}}{{cosx}\sqrt{{cosx}}}−\mathrm{1}\right)= \\ $$$$\underset{{x}\rightarrow\mathrm{0}^{+} } {{lim}}\frac{\mathrm{1}−{cos}^{\mathrm{3}} {x}}{{x}^{\mathrm{2}} {cosx}\sqrt{{cosx}}\left(\mathrm{1}+{cosx}\sqrt{{cosx}}\right)}=\underset{{x}\rightarrow\mathrm{0}^{+} } {{lim}}\frac{\left(\mathrm{1}−{cosx}\right)\left(\mathrm{1}+{cosx}+{cos}^{\mathrm{2}} {x}\right)}{{x}^{\mathrm{2}} {cosx}\sqrt{{cosx}}\left(\mathrm{1}+{cosx}\sqrt{{cosx}}\right)}= \\ $$$$=\underset{{x}\rightarrow\mathrm{0}^{+} } {{lim}}\frac{\mathrm{2}{sin}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}\left(\mathrm{1}+{cosx}+{cos}^{\mathrm{2}} {x}\right)}{{x}^{\mathrm{2}} {cosx}\sqrt{{cosx}}\left(\mathrm{1}+{cosx}\sqrt{{cosx}}\right)}= \\ $$$$=\underset{{x}\rightarrow\mathrm{0}^{+} } {{lim}}\left(\frac{{sin}\frac{{x}}{\mathrm{2}}}{\frac{{x}}{\mathrm{2}}}\right)^{\mathrm{2}} \frac{\left(\mathrm{1}+{cosx}+{cos}^{\mathrm{2}} {x}\right)}{\mathrm{2}{cosx}\sqrt{{cosx}}\left(\mathrm{1}+{cosx}\sqrt{{cosx}}\right)}=\frac{\mathrm{3}}{\mathrm{4}} \\ $$$$ \\ $$$$ \\ $$
Answered by john_santu last updated on 04/Aug/21