Question Number 114543 by bemath last updated on 19/Sep/20
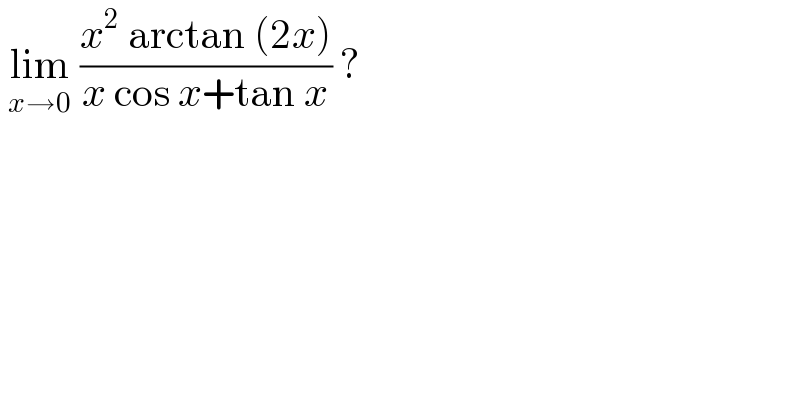
$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{2}} \:\mathrm{arctan}\:\left(\mathrm{2}{x}\right)}{{x}\:\mathrm{cos}\:{x}+\mathrm{tan}\:{x}}\:? \\ $$
Answered by bobhans last updated on 19/Sep/20
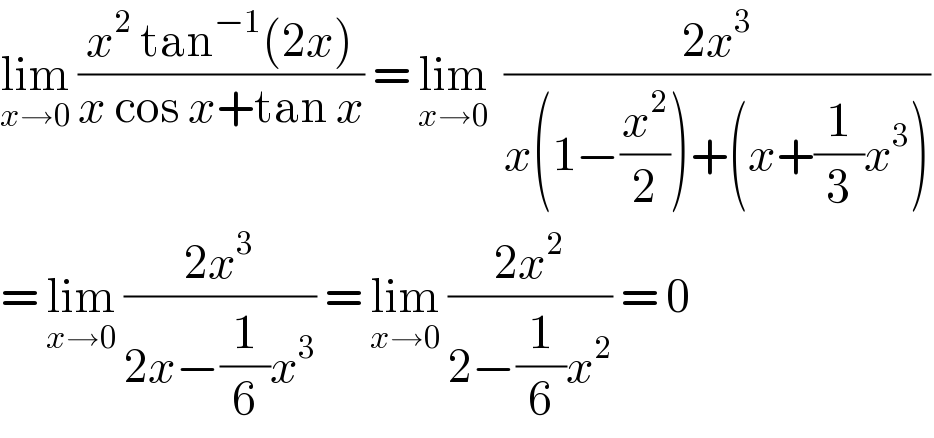
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{2}} \:\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}{x}\right)}{{x}\:\mathrm{cos}\:{x}+\mathrm{tan}\:{x}}\:=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\:\frac{\mathrm{2}{x}^{\mathrm{3}} }{{x}\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\right)+\left({x}+\frac{\mathrm{1}}{\mathrm{3}}{x}^{\mathrm{3}} \right)} \\ $$$$=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{2}{x}^{\mathrm{3}} }{\mathrm{2}{x}−\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{3}} }\:=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{2}{x}^{\mathrm{2}} }{\mathrm{2}−\frac{\mathrm{1}}{\mathrm{6}}{x}^{\mathrm{2}} }\:=\:\mathrm{0} \\ $$
Answered by mathmax by abdo last updated on 19/Sep/20
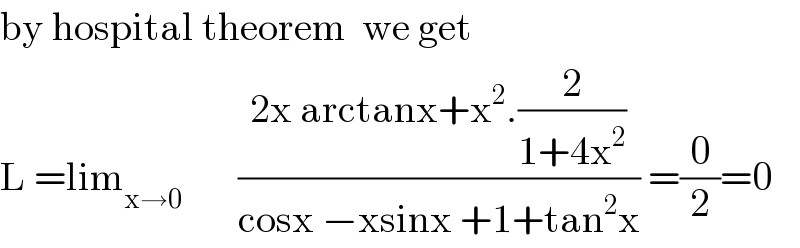
$$\mathrm{by}\:\mathrm{hospital}\:\mathrm{theorem}\:\:\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{L}\:=\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \:\:\:\:\:\:\:\frac{\mathrm{2x}\:\mathrm{arctanx}+\mathrm{x}^{\mathrm{2}} .\frac{\mathrm{2}}{\mathrm{1}+\mathrm{4x}^{\mathrm{2}} }}{\mathrm{cosx}\:−\mathrm{xsinx}\:+\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \mathrm{x}}\:=\frac{\mathrm{0}}{\mathrm{2}}=\mathrm{0} \\ $$
