Question Number 118776 by obaidullah last updated on 19/Oct/20
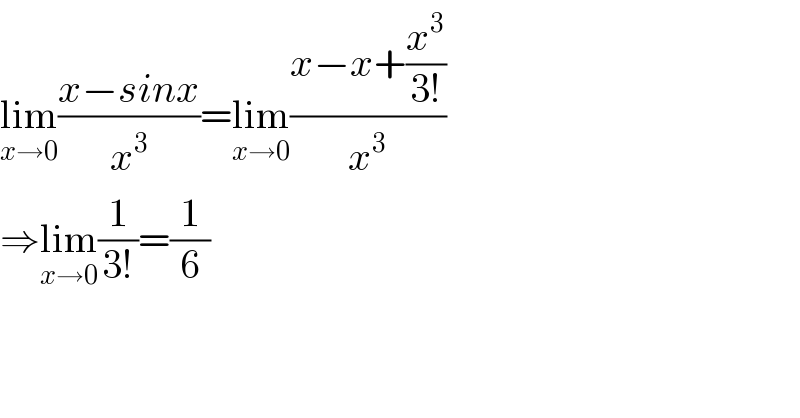
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{x}−{sinx}}{{x}^{\mathrm{3}} }=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{x}−{x}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}}{{x}^{\mathrm{3}} } \\ $$$$\Rightarrow\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{3}!}=\frac{\mathrm{1}}{\mathrm{6}} \\ $$
