Question Number 165063 by MathsFan last updated on 25/Jan/22
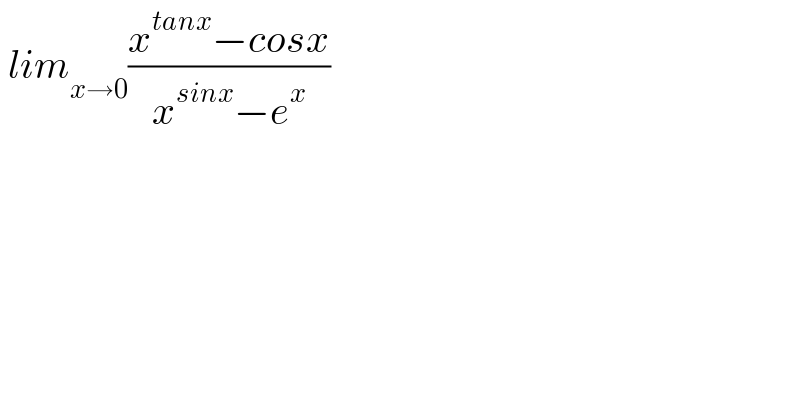
$$\:{lim}_{{x}\rightarrow\mathrm{0}} \frac{{x}^{{tanx}} −{cosx}}{{x}^{{sinx}} −{e}^{{x}} } \\ $$
Commented by MathsFan last updated on 25/Jan/22

$${any}\:{help}? \\ $$
Answered by aleks041103 last updated on 27/Jan/22
![lim_(x→0) ((x^(tanx) −cosx)/(x^(sinx) −e^x ))=L first we substitute (([0^0 ]−1)/([0^0 ]−1))=? lim_(x→0) x^(tanx) =e^(lim_(x→0) tan(x)ln(x)) lim_(x→0) ((lnx)/(ctgx))=^(l′hopital) lim_(x→0) ((1/x)/(−(1/(sin^2 x))))=−lim_(x→0) ((sin^2 x)/x)= =−lim_(x→0) ((2sin(x)cos(x))/1)=0 ⇒lim_(x→0) x^(tan(x)) =e^0 =1 lim_(x→0) x^(sin(x)) =e^(lim_(x→0) ln(x) sin (x)) lim_(x→0) ln(x)sin(x)=lim_(x→0) ((ln(x))/(1/(sin(x))))= =lim_(x→0) ((1/x)/(−((cos(x))/(sin^2 x))))=−lim_(x→0) ((sin^2 x)/(x cos(x)))=−lim_(x→0) ((sin^2 x)/x)=0 ⇒lim_(x→0) x^(sin(x)) =e^0 =1 ⇒lim_(x→0) ((x^(tanx) −cosx)/(x^(sinx) −e^x ))=[((1−1)/(1−1))]=[(0/0)] tanx→x sinx→x l′hopital (x^x )′=x^x (x ln(x))′=x^x (1+ln(x)) ⇒L=lim_(x→0) ((x^x (1+ln(x))+sin(x))/(x^x (1+ln(x))−e^x ))= =lim_(x→0) ((x^x (1+ln(x)))/(x^x (1+ln(x))−e^x ))=1+lim_(x→0) (e^x /(x^x (1+ln(x))−e^x ))= =1+lim_(x→0) (1/(ln(x)))=1 L=1](https://www.tinkutara.com/question/Q165197.png)
$${lim}_{{x}\rightarrow\mathrm{0}} \frac{{x}^{{tanx}} −{cosx}}{{x}^{{sinx}} −{e}^{{x}} }={L} \\ $$$${first}\:{we}\:{substitute} \\ $$$$\frac{\left[\mathrm{0}^{\mathrm{0}} \right]−\mathrm{1}}{\left[\mathrm{0}^{\mathrm{0}} \right]−\mathrm{1}}=? \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\:{x}^{{tanx}} ={e}^{\underset{{x}\rightarrow\mathrm{0}} {{lim}tan}\left({x}\right){ln}\left({x}\right)} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\:\frac{{lnx}}{{ctgx}}\overset{{l}'{hopital}} {=}\:\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{\frac{\mathrm{1}}{{x}}}{−\frac{\mathrm{1}}{{sin}^{\mathrm{2}} {x}}}=−\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{{sin}^{\mathrm{2}} {x}}{{x}}= \\ $$$$=−\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{\mathrm{2}{sin}\left({x}\right){cos}\left({x}\right)}{\mathrm{1}}=\mathrm{0} \\ $$$$\Rightarrow\underset{{x}\rightarrow\mathrm{0}} {{lim}}\:{x}^{{tan}\left({x}\right)} ={e}^{\mathrm{0}} =\mathrm{1} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\:{x}^{{sin}\left({x}\right)} ={e}^{\underset{{x}\rightarrow\mathrm{0}} {{lim}ln}\left({x}\right)\:{sin}\:\left({x}\right)} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {{lim}}\:{ln}\left({x}\right){sin}\left({x}\right)=\underset{{x}\rightarrow\mathrm{0}} {{lim}}\:\frac{{ln}\left({x}\right)}{\frac{\mathrm{1}}{{sin}\left({x}\right)}}= \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{\frac{\mathrm{1}}{{x}}}{−\frac{{cos}\left({x}\right)}{{sin}^{\mathrm{2}} {x}}}=−\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{{sin}^{\mathrm{2}} {x}}{{x}\:{cos}\left({x}\right)}=−\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{{sin}^{\mathrm{2}} {x}}{{x}}=\mathrm{0} \\ $$$$\Rightarrow\underset{{x}\rightarrow\mathrm{0}} {{lim}x}^{{sin}\left({x}\right)} ={e}^{\mathrm{0}} =\mathrm{1} \\ $$$$\Rightarrow{lim}_{{x}\rightarrow\mathrm{0}} \frac{{x}^{{tanx}} −{cosx}}{{x}^{{sinx}} −{e}^{{x}} }=\left[\frac{\mathrm{1}−\mathrm{1}}{\mathrm{1}−\mathrm{1}}\right]=\left[\frac{\mathrm{0}}{\mathrm{0}}\right] \\ $$$${tanx}\rightarrow{x} \\ $$$${sinx}\rightarrow{x} \\ $$$${l}'{hopital} \\ $$$$\left({x}^{{x}} \right)'={x}^{{x}} \left({x}\:{ln}\left({x}\right)\right)'={x}^{{x}} \left(\mathrm{1}+{ln}\left({x}\right)\right) \\ $$$$\Rightarrow{L}=\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{{x}^{{x}} \left(\mathrm{1}+{ln}\left({x}\right)\right)+{sin}\left({x}\right)}{{x}^{{x}} \left(\mathrm{1}+{ln}\left({x}\right)\right)−{e}^{{x}} }= \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{{x}^{{x}} \left(\mathrm{1}+{ln}\left({x}\right)\right)}{{x}^{{x}} \left(\mathrm{1}+{ln}\left({x}\right)\right)−{e}^{{x}} }=\mathrm{1}+\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{{e}^{{x}} }{{x}^{{x}} \left(\mathrm{1}+{ln}\left({x}\right)\right)−{e}^{{x}} }= \\ $$$$=\mathrm{1}+\underset{{x}\rightarrow\mathrm{0}} {{lim}}\frac{\mathrm{1}}{{ln}\left({x}\right)}=\mathrm{1} \\ $$$${L}=\mathrm{1} \\ $$
Commented by aleks041103 last updated on 27/Jan/22

