Question Number 149184 by liberty last updated on 03/Aug/21
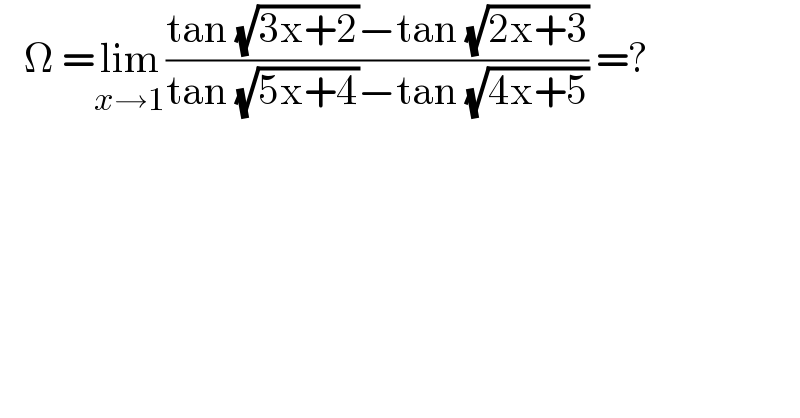
$$\:\:\:\Omega\:=\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\mathrm{tan}\:\sqrt{\mathrm{3x}+\mathrm{2}}−\mathrm{tan}\:\sqrt{\mathrm{2x}+\mathrm{3}}}{\mathrm{tan}\:\sqrt{\mathrm{5x}+\mathrm{4}}−\mathrm{tan}\:\sqrt{\mathrm{4x}+\mathrm{5}}}\:=? \\ $$
Answered by EDWIN88 last updated on 03/Aug/21
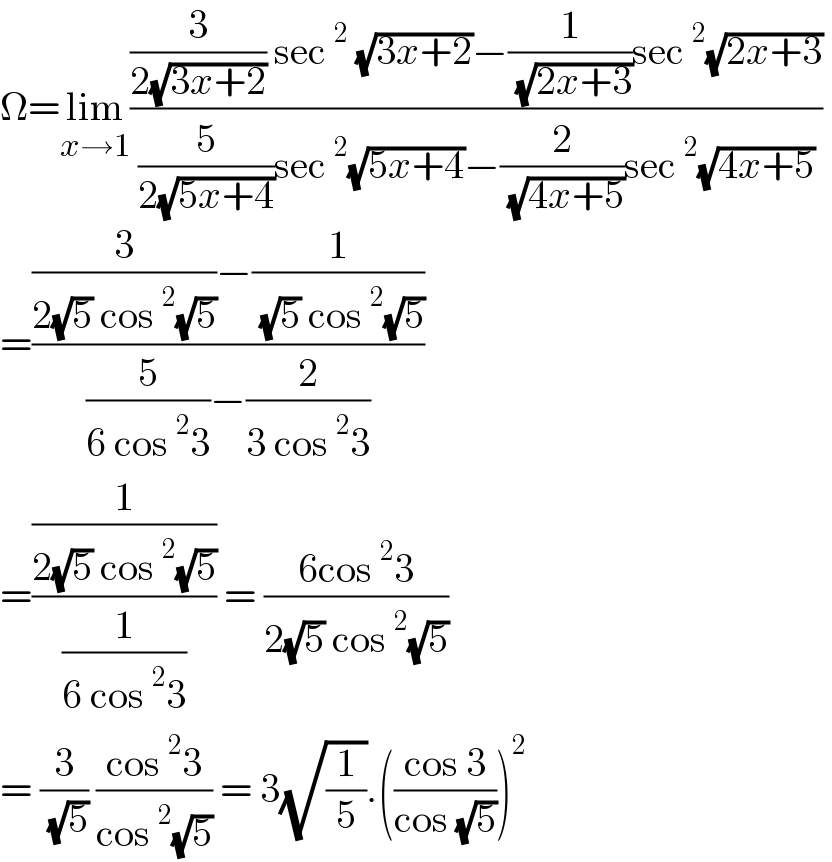
$$\Omega=\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\frac{\mathrm{3}}{\mathrm{2}\sqrt{\mathrm{3}{x}+\mathrm{2}}}\:\mathrm{sec}\:^{\mathrm{2}} \:\sqrt{\mathrm{3}{x}+\mathrm{2}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}{x}+\mathrm{3}}}\mathrm{sec}\:^{\mathrm{2}} \sqrt{\mathrm{2}{x}+\mathrm{3}}}{\frac{\mathrm{5}}{\mathrm{2}\sqrt{\mathrm{5}{x}+\mathrm{4}}}\mathrm{sec}\:^{\mathrm{2}} \sqrt{\mathrm{5}{x}+\mathrm{4}}−\frac{\mathrm{2}}{\:\sqrt{\mathrm{4}{x}+\mathrm{5}}}\mathrm{sec}\:^{\mathrm{2}} \sqrt{\mathrm{4}{x}+\mathrm{5}}}\: \\ $$$$=\frac{\frac{\mathrm{3}}{\mathrm{2}\sqrt{\mathrm{5}}\:\mathrm{cos}\:^{\mathrm{2}} \sqrt{\mathrm{5}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}\:\mathrm{cos}\:^{\mathrm{2}} \sqrt{\mathrm{5}}}}{\frac{\mathrm{5}}{\mathrm{6}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{3}}−\frac{\mathrm{2}}{\mathrm{3}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{3}}} \\ $$$$=\frac{\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{5}}\:\mathrm{cos}\:^{\mathrm{2}} \sqrt{\mathrm{5}}}}{\frac{\mathrm{1}}{\mathrm{6}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{3}}}\:=\:\frac{\mathrm{6cos}\:^{\mathrm{2}} \mathrm{3}}{\mathrm{2}\sqrt{\mathrm{5}}\:\mathrm{cos}\:^{\mathrm{2}} \sqrt{\mathrm{5}}} \\ $$$$=\:\frac{\mathrm{3}}{\:\sqrt{\mathrm{5}}}\:\frac{\mathrm{cos}\:^{\mathrm{2}} \mathrm{3}}{\mathrm{cos}\:^{\mathrm{2}} \sqrt{\mathrm{5}}}\:=\:\mathrm{3}\sqrt{\frac{\mathrm{1}}{\mathrm{5}}}.\left(\frac{\mathrm{cos}\:\mathrm{3}}{\mathrm{cos}\:\sqrt{\mathrm{5}}}\right)^{\mathrm{2}} \\ $$
