Question Number 128604 by john_santu last updated on 08/Jan/21

$$\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{\mathrm{x}^{\mathrm{x}} −\mathrm{x}}{\mathrm{ln}\:\mathrm{x}−\mathrm{x}+\mathrm{1}}\:? \\ $$
Answered by liberty last updated on 09/Jan/21
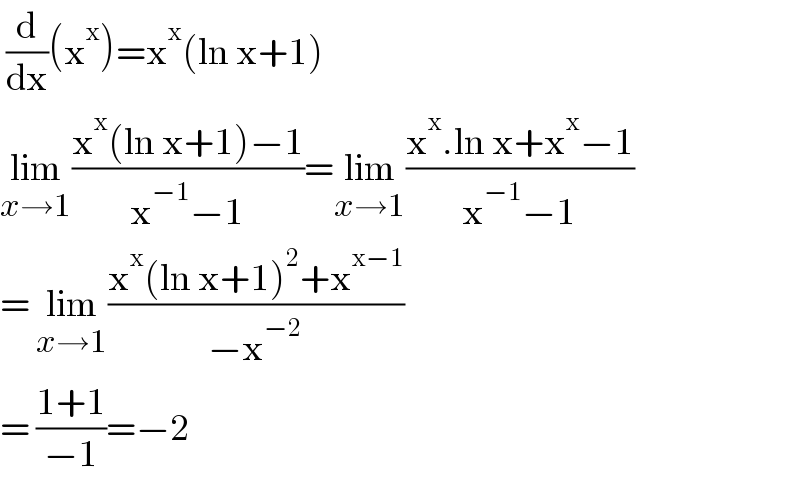
$$\:\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^{\mathrm{x}} \right)=\mathrm{x}^{\mathrm{x}} \left(\mathrm{ln}\:\mathrm{x}+\mathrm{1}\right) \\ $$$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\mathrm{x}^{\mathrm{x}} \left(\mathrm{ln}\:\mathrm{x}+\mathrm{1}\right)−\mathrm{1}}{\mathrm{x}^{−\mathrm{1}} −\mathrm{1}}=\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\mathrm{x}^{\mathrm{x}} .\mathrm{ln}\:\mathrm{x}+\mathrm{x}^{\mathrm{x}} −\mathrm{1}}{\mathrm{x}^{−\mathrm{1}} −\mathrm{1}} \\ $$$$=\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\mathrm{x}^{\mathrm{x}} \left(\mathrm{ln}\:\mathrm{x}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{x}^{\mathrm{x}−\mathrm{1}} }{−\mathrm{x}^{−\mathrm{2}} } \\ $$$$=\:\frac{\mathrm{1}+\mathrm{1}}{−\mathrm{1}}=−\mathrm{2} \\ $$
