Question Number 129723 by Adel last updated on 18/Jan/21
![lim_(x→100) [(x^(100) −100^x )(1/(100−x))]=? DANISH](https://www.tinkutara.com/question/Q129723.png)
$$\underset{{x}\rightarrow\mathrm{100}} {\mathrm{lim}}\left[\left(\mathrm{x}^{\mathrm{100}} −\mathrm{100}^{\mathrm{x}} \right)\frac{\mathrm{1}}{\mathrm{100}−\mathrm{x}}\right]=? \\ $$$$\mathcal{DANISH} \\ $$
Answered by bramlexs22 last updated on 18/Jan/21
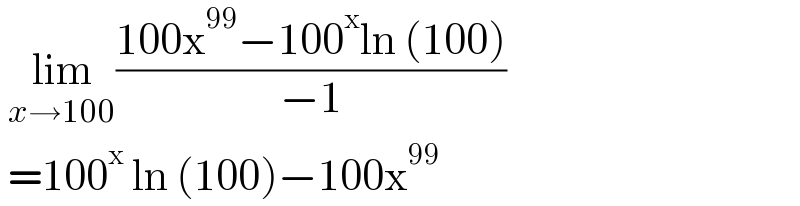
$$\:\underset{{x}\rightarrow\mathrm{100}} {\mathrm{lim}}\frac{\mathrm{100x}^{\mathrm{99}} −\mathrm{100}^{\mathrm{x}} \mathrm{ln}\:\left(\mathrm{100}\right)}{−\mathrm{1}} \\ $$$$\:=\mathrm{100}^{\mathrm{x}} \:\mathrm{ln}\:\left(\mathrm{100}\right)−\mathrm{100x}^{\mathrm{99}} \\ $$
Commented by Adel last updated on 18/Jan/21
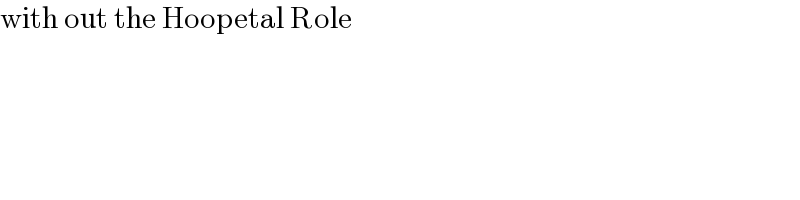
$$\mathrm{with}\:\mathrm{out}\:\mathrm{the}\:\mathrm{Hoopetal}\:\mathrm{Role} \\ $$
