lim-x-2-f-x-2-6-x-2-4-if-lim-x-4-4-f-x-24-x-4-

Question Number 126824 by MathSh last updated on 24/Dec/20

\underset{x \to 2}{l i m} \frac{f (x + 2) - 6}{x - 2} = 4 i f, \underset{x \to 4}{l i m} \frac{4 \cdot f (x) - 24}{x - 4} = ?

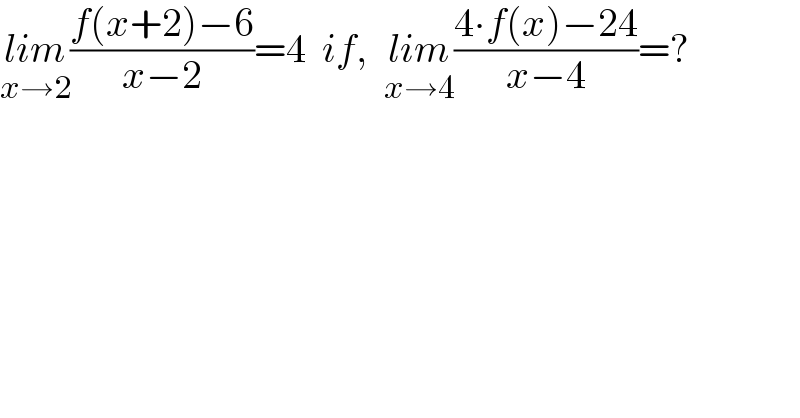
Answered by mahdipoor last updated on 24/Dec/20

\underset{x \to 2}{l i m} \frac{f (x + 2) - 6}{x - 2} = \frac{f (4) - 6}{0} = 4 \Rightarrow f (4) - 6 = 0

\overset{L^{'} h o p i t a l}{\Rightarrow} \underset{x \to 2}{l i m} \frac{f (x + 2) - 6}{x - 2} = \frac{f^{'} (x + 2)}{1} = f^{'} (4) = 4

\underset{x \to 4}{l i m} \frac{4 \cdot f (x) - 24}{x - 4} = \frac{0}{0} \overset{L^{'} h o p i t a l}{\Rightarrow} \frac{4 \times f^{'} (x)}{1} = 4 \times f^{'} (4) = 16

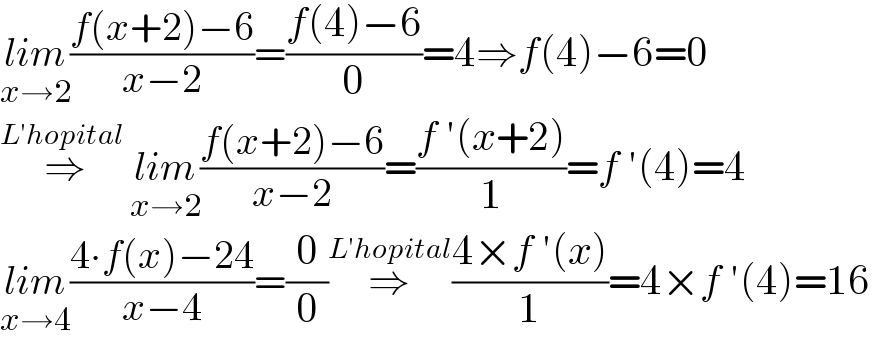
Commented by MathSh last updated on 24/Dec/20

S i r, f^{'} (4) = 4

4 \cdot 4 = 16

Commented by mahdipoor last updated on 24/Dec/20

o h! y o u a r e r i g h t

t h a n k f o r t i p

Commented by MathSh last updated on 24/Dec/20

T h a n k y o u S i r, f o r h e l p i n g t h e

Facebook Tweet Pin