lim-x-a-1-b-1-x-a-x-a-b-gt-0-

Question Number 34554 by rahul 19 last updated on 07/May/18

\lim_{x \to \infty} {(\frac{a - 1 + b^{\frac{1}{x}}}{a})}^{x} = ?

(a, b > 0)

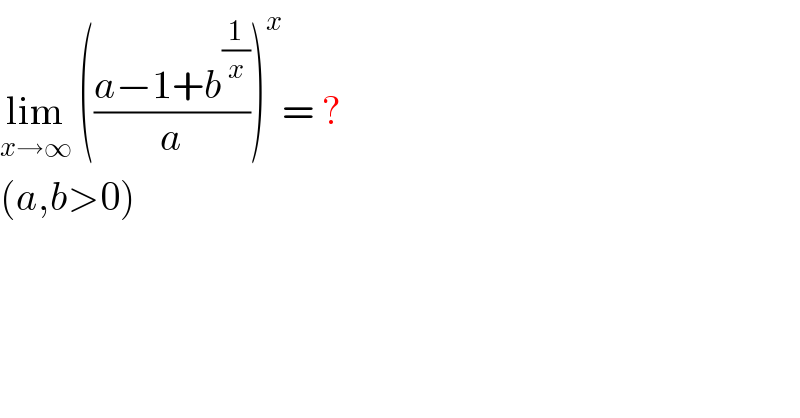
Commented by math khazana by abdo last updated on 09/May/18

l e t p u t A (x) = {(\frac{a - 1 + b^{\frac{1}{x}}}{a})}^{x}

A (x) = {1 + \frac{b^{\frac{1}{x}} - 1}{a}}^{x} \Rightarrow l n (A (x)) = x l n {1 + \frac{b^{\frac{1}{x}} - 1}{a}}

x l n {1 + \frac{b^{\frac{1}{x}} - 1}{a}} \sim x \frac{b^{\frac{1}{x}} - 1}{a} c h . \frac{1}{x} = t g i v e

= \frac{1}{t} \frac{b^{t} - 1}{a} = \frac{1}{t} \frac{e^{t l n b} - 1}{a} \sim \frac{t l n b}{t a} = \frac{l n b}{a} \Rightarrow

{l i m}_{x \to + \infty} l n (A (x)) = \frac{l n b}{a} \Rightarrow {l i m}_{x \to + \infty} A (x) = e^{\frac{l n b}{a}}

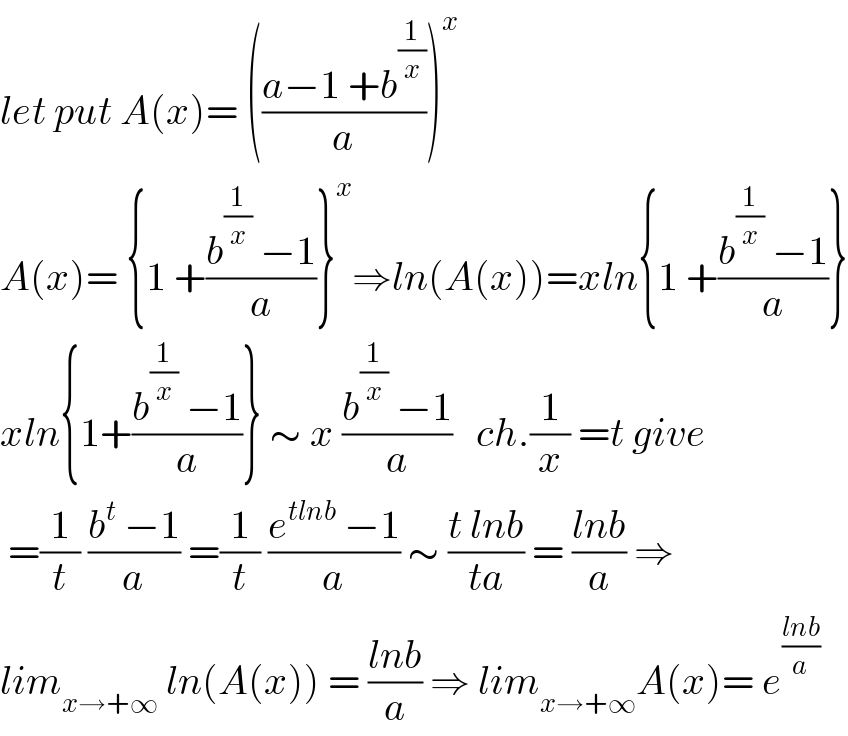
Commented by Joel578 last updated on 10/May/18

Sir, would you mind to explain 3 rd line ?

x \ln {1 + \frac{b^{\frac{1}{x}} - 1}{a}} \sim x (\frac{b^{\frac{1}{x}} - 1}{a})

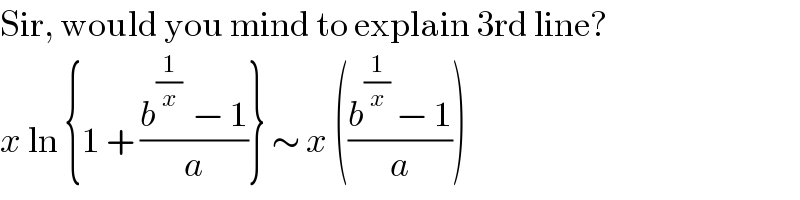
Commented by abdo mathsup 649 cc last updated on 11/May/18

f o r x \in v (0) l n (1 + u) \sim u t a k e u = \frac{b^{\frac{1}{x}} - 1}{a} \Rightarrow

l n {1 + \frac{b^{\frac{1}{x}} - 1}{a}} \sim \frac{b^{\frac{1}{x}} - 1}{a} \dots .

Answered by Joel578 last updated on 08/May/18

L = \lim_{x \to \infty} {(\frac{a + b^{\frac{1}{x}} - 1}{a})}^{x}

\ln L = \lim_{x \to \infty} [x . \ln (\frac{a + b^{\frac{1}{x}} - 1}{a})]

= \lim_{x \to \infty} [\frac{\ln (\frac{a + b^{1 / x} - 1}{a})}{1 / x}]

= \lim_{x \to \infty} [\frac{- \frac{\ln b . b^{1 / x}}{x^{2} (a + b^{1 / x} - 1)}}{- \frac{1}{x^{2}}}]

= \lim_{x \to \infty} [\frac{\ln b . b^{1 / x}}{a + b^{1 / x} - 1}]

= \ln b [\lim_{x \to \infty} (\frac{b^{1 / x}}{a + b^{1 / x} - 1})] = \ln b . (\frac{1}{a + 1 - 1})

\ln L = (\frac{1}{a}) . \ln b = \ln (b^{\frac{1}{a}})

L = b^{\frac{1}{a}}

Commented by rahul 19 last updated on 12/May/18

S i r, p l s e x p l a i n n u m e r a t o r o f l i n e 3 (i k n o w y o u

h a v e u s e l - h o s p i t a l .)

Commented by rahul 19 last updated on 12/May/18

��