Menu Close

lim-x-ln-3e-2x-5e-x-2-ln-27e-3x-1-

Tinku Tara 0 Comments
Pin




Question Number 103463 by bemath last updated on 15/Jul/20
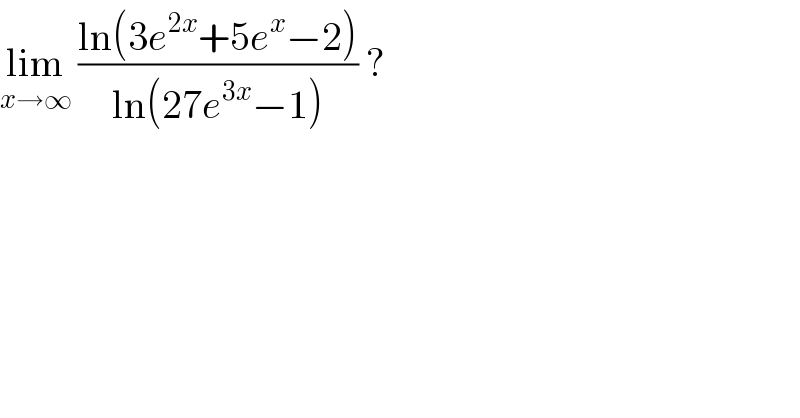
lim_(x→∞) ((ln(3e^(2x) +5e^x −2))/(ln(27e^(3x) −1))) ?
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{ln}\left(\mathrm{3}{e}^{\mathrm{2}{x}} +\mathrm{5}{e}^{{x}} −\mathrm{2}\right)}{\mathrm{ln}\left(\mathrm{27}{e}^{\mathrm{3}{x}} −\mathrm{1}\right)}\:? \\ $$
Answered by Worm_Tail last updated on 15/Jul/20
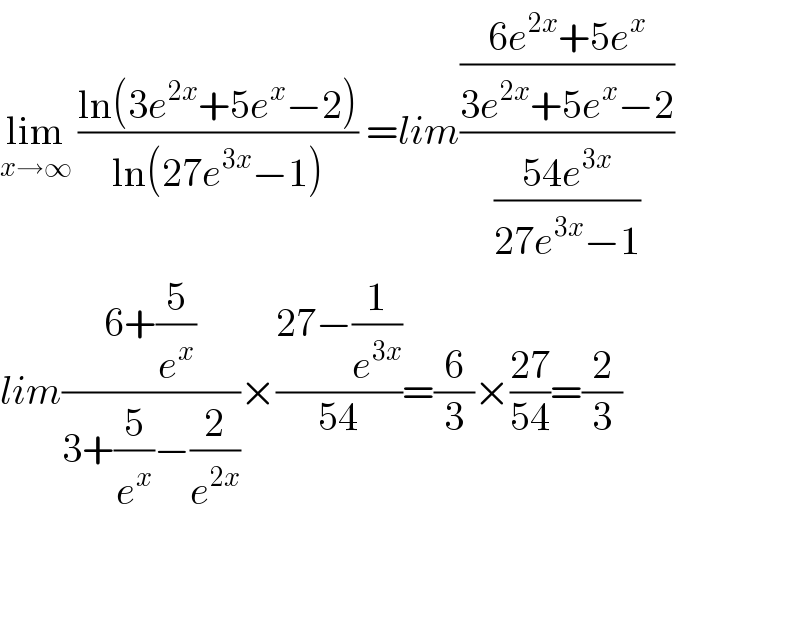
lim_(x→∞) ((ln(3e^(2x) +5e^x −2))/(ln(27e^(3x) −1))) =lim(((6e^(2x) +5e^x )/(3e^(2x) +5e^x −2))/((54e^(3x) )/(27e^(3x) −1))) lim((6+(5/e^x ))/(3+(5/e^x )−(2/e^(2x) )))×((27−(1/e^(3x) ))/(54))=(6/3)×((27)/(54))=(2/3)
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{ln}\left(\mathrm{3}{e}^{\mathrm{2}{x}} +\mathrm{5}{e}^{{x}} −\mathrm{2}\right)}{\mathrm{ln}\left(\mathrm{27}{e}^{\mathrm{3}{x}} −\mathrm{1}\right)}\:={lim}\frac{\frac{\mathrm{6}{e}^{\mathrm{2}{x}} +\mathrm{5}{e}^{{x}} }{\mathrm{3}{e}^{\mathrm{2}{x}} +\mathrm{5}{e}^{{x}} −\mathrm{2}}}{\frac{\mathrm{54}{e}^{\mathrm{3}{x}} }{\mathrm{27}{e}^{\mathrm{3}{x}} −\mathrm{1}}} \\ $$$${lim}\frac{\mathrm{6}+\frac{\mathrm{5}}{{e}^{{x}} }}{\mathrm{3}+\frac{\mathrm{5}}{{e}^{{x}} }−\frac{\mathrm{2}}{{e}^{\mathrm{2}{x}} }}×\frac{\mathrm{27}−\frac{\mathrm{1}}{{e}^{\mathrm{3}{x}} }}{\mathrm{54}}=\frac{\mathrm{6}}{\mathrm{3}}×\frac{\mathrm{27}}{\mathrm{54}}=\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$ \\ $$$$ \\ $$
Answered by bobhans last updated on 15/Jul/20
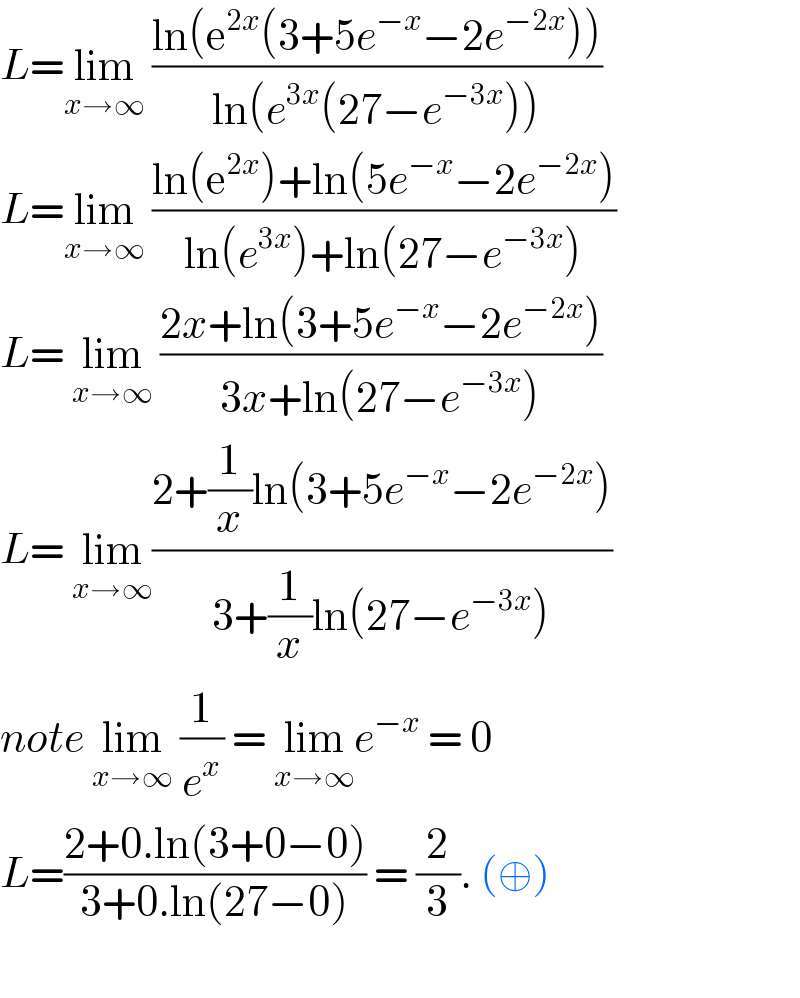
L=lim_(x→∞) ((ln(e^(2x) (3+5e^(−x) −2e^(−2x) )))/(ln(e^(3x) (27−e^(−3x) )))) L=lim_(x→∞) ((ln(e^(2x) )+ln(5e^(−x) −2e^(−2x) ))/(ln(e^(3x) )+ln(27−e^(−3x) ))) L= lim_(x→∞) ((2x+ln(3+5e^(−x) −2e^(−2x) ))/(3x+ln(27−e^(−3x) ))) L= lim_(x→∞) ((2+(1/x)ln(3+5e^(−x) −2e^(−2x) ))/(3+(1/x)ln(27−e^(−3x) ))) note lim_(x→∞) (1/e^x ) = lim_(x→∞) e^(−x) = 0 L=((2+0.ln(3+0−0))/(3+0.ln(27−0))) = (2/3). (⊕)
$${L}=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{ln}\left(\mathrm{e}^{\mathrm{2}{x}} \left(\mathrm{3}+\mathrm{5}{e}^{−{x}} −\mathrm{2}{e}^{−\mathrm{2}{x}} \right)\right)}{\mathrm{ln}\left({e}^{\mathrm{3}{x}} \left(\mathrm{27}−{e}^{−\mathrm{3}{x}} \right)\right)} \\ $$$${L}=\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{ln}\left(\mathrm{e}^{\mathrm{2}{x}} \right)+\mathrm{ln}\left(\mathrm{5}{e}^{−{x}} −\mathrm{2}{e}^{−\mathrm{2}{x}} \right)}{\mathrm{ln}\left({e}^{\mathrm{3}{x}} \right)+\mathrm{ln}\left(\mathrm{27}−{e}^{−\mathrm{3}{x}} \right)} \\ $$$${L}=\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{2}{x}+\mathrm{ln}\left(\mathrm{3}+\mathrm{5}{e}^{−{x}} −\mathrm{2}{e}^{−\mathrm{2}{x}} \right)}{\mathrm{3}{x}+\mathrm{ln}\left(\mathrm{27}−{e}^{−\mathrm{3}{x}} \right)} \\ $$$${L}=\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{2}+\frac{\mathrm{1}}{{x}}\mathrm{ln}\left(\mathrm{3}+\mathrm{5}{e}^{−{x}} −\mathrm{2}{e}^{−\mathrm{2}{x}} \right)}{\mathrm{3}+\frac{\mathrm{1}}{{x}}\mathrm{ln}\left(\mathrm{27}−{e}^{−\mathrm{3}{x}} \right)} \\ $$$${note}\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{1}}{{e}^{{x}} }\:=\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}{e}^{−{x}} \:=\:\mathrm{0} \\ $$$${L}=\frac{\mathrm{2}+\mathrm{0}.\mathrm{ln}\left(\mathrm{3}+\mathrm{0}−\mathrm{0}\right)}{\mathrm{3}+\mathrm{0}.\mathrm{ln}\left(\mathrm{27}−\mathrm{0}\right)}\:=\:\frac{\mathrm{2}}{\mathrm{3}}.\:\left(\oplus\right) \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *