lim-x-ln-e-x-1-x-

Question Number 93821 by john santu last updated on 15/May/20

\lim_{x \to + \infty} \ln (e^{x} + 1) - x =

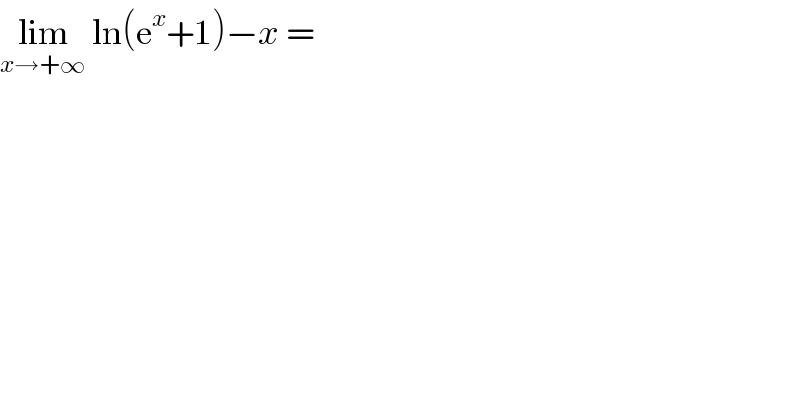
Commented by JDamian last updated on 15/May/20

0

Commented by mathmax by abdo last updated on 15/May/20

f (x) = l n (e^{x} + 1) - x \Rightarrow f (x) = l n (e^{x} (1 + e^{- x})) - x

= x + l n (1 + e^{- x}) - x = l n (1 + e^{- x}) \Rightarrow {l i m}_{x \to + \infty} f (x) = 0

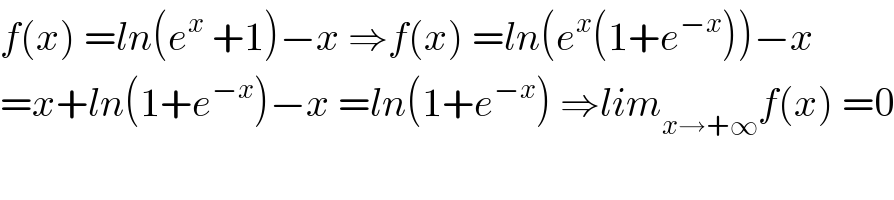
Commented by Kunal12588 last updated on 15/May/20

f (x) = l n (e^{x} + 1) - x = l n (e^{x} + 1) - l n (e^{x})

= l n (1 + e^{- x}) \Rightarrow \underset{x \to \infty^{+}}{l i m f} (x) = 0

Answered by john santu last updated on 15/May/20