Question Number 115122 by bobhans last updated on 23/Sep/20
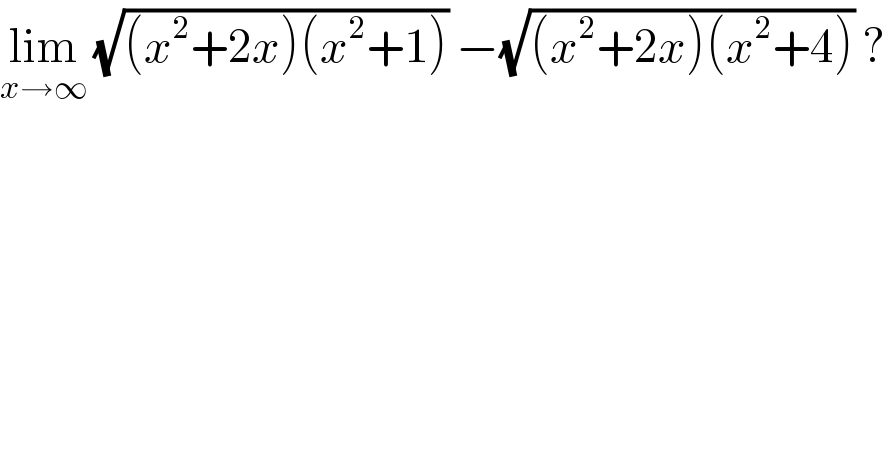
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\sqrt{\left({x}^{\mathrm{2}} +\mathrm{2}{x}\right)\left({x}^{\mathrm{2}} +\mathrm{1}\right)}\:−\sqrt{\left({x}^{\mathrm{2}} +\mathrm{2}{x}\right)\left({x}^{\mathrm{2}} +\mathrm{4}\right)}\:? \\ $$
Commented by malwan last updated on 23/Sep/20
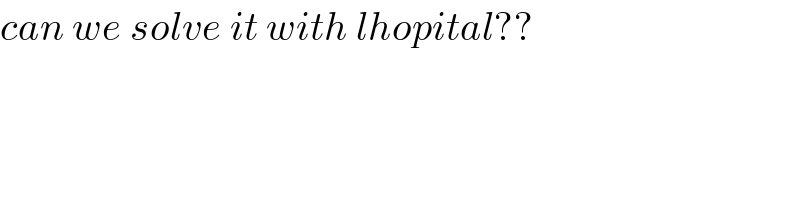
$${can}\:{we}\:{solve}\:{it}\:{with}\:{lhopital}?? \\ $$
Answered by john santu last updated on 23/Sep/20

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\sqrt{{x}^{\mathrm{2}} +\mathrm{2}{x}}\:\left(\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}−\sqrt{{x}^{\mathrm{2}} +\mathrm{4}}\right)= \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\sqrt{{x}^{\mathrm{2}} +\mathrm{2}{x}}\:\left(\frac{−\mathrm{3}}{\:\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}+\sqrt{{x}^{\mathrm{2}} +\mathrm{4}}}\right)\:= \\ $$$$−\mathrm{3}\:×\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{{x}\:\sqrt{\mathrm{1}+\frac{\mathrm{2}}{{x}}}}{{x}\:\left(\sqrt{\mathrm{1}+\frac{\mathrm{1}}{{x}}}+\sqrt{\mathrm{1}+\frac{\mathrm{4}}{{x}}}\right)}\:= \\ $$$$−\frac{\mathrm{3}}{\mathrm{2}}. \\ $$
Answered by bemath last updated on 23/Sep/20

Answered by Dwaipayan Shikari last updated on 23/Sep/20
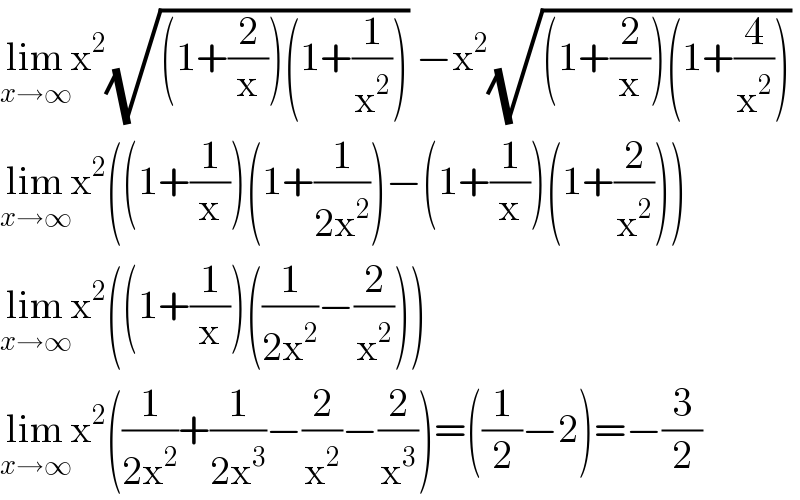
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}x}^{\mathrm{2}} \sqrt{\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{x}}\right)\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }\right)}\:−\mathrm{x}^{\mathrm{2}} \sqrt{\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{x}}\right)\left(\mathrm{1}+\frac{\mathrm{4}}{\mathrm{x}^{\mathrm{2}} }\right)} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}x}^{\mathrm{2}} \left(\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{x}}\right)\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} }\right)−\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{x}}\right)\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }\right)\right) \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}x}^{\mathrm{2}} \left(\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{x}}\right)\left(\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} }−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }\right)\right) \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}x}^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{3}} }−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{3}} }\right)=\left(\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{2}\right)=−\frac{\mathrm{3}}{\mathrm{2}} \\ $$
Answered by Bird last updated on 24/Sep/20

$${let}\:{f}\left({x}\right)\:=\sqrt{\left({x}^{\mathrm{2}} +\mathrm{2}{x}\right)\left({x}^{\mathrm{2}} +\mathrm{1}\right)}−\sqrt{\left({x}^{\mathrm{2}} +\mathrm{2}{x}\right)\left({x}^{\mathrm{2}} +\mathrm{4}\right)} \\ $$$${f}\left({x}\right)={x}^{\mathrm{2}} \sqrt{\left(\mathrm{1}+\frac{\mathrm{2}}{{x}}\right)}.\sqrt{\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}−{x}^{\mathrm{2}} \sqrt{\mathrm{1}+\frac{\mathrm{2}}{{x}}}.\sqrt{\mathrm{1}+\frac{\mathrm{4}}{{x}^{\mathrm{2}} }} \\ $$$${f}\left({x}\right)\sim{x}^{\mathrm{2}} \left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}{x}^{\mathrm{2}} }\right) \\ $$$$−{x}^{\mathrm{2}} \left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)\left(\mathrm{1}+\frac{\mathrm{2}}{{x}^{\mathrm{2}} }\right)\left({x}\rightarrow\infty\right)\:\Rightarrow \\ $$$${f}\left({x}\right)\sim\left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)\left\{{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}−{x}^{\mathrm{2}} −\mathrm{2}\right\} \\ $$$${f}\left({x}\right)\sim\left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)\left(−\frac{\mathrm{3}}{\mathrm{2}}\right)\:\Rightarrow \\ $$$${lim}_{{x}\rightarrow\infty} {f}\left({x}\right)\:=−\frac{\mathrm{3}}{\mathrm{2}} \\ $$
