lim-x-x-2-x-x-

Question Number 180106 by cherokeesay last updated on 07/Nov/22

\lim_{x \to \infty} \sqrt{x^{2} + x} - x

Answered by a.lgnaoui last updated on 07/Nov/22

\sqrt{x^{2} + x} - x = \frac{(\sqrt{x^{2} + x} - x) (\sqrt{x^{2} + x} + x)}{\sqrt{x^{2} + x} + x} (x \in (] - \infty, 0] \cup [- 1, + \infty [)

= \frac{x}{\sqrt{x^{2} + x} + x} = \frac{x}{x (\sqrt{1 + \frac{1}{x}} + 1)} = \frac{1}{\sqrt{1 + \frac{1}{x}} + 1}

\lim_{x \to \infty} \sqrt{x^{2} + x} - x = \lim_{x \to \infty} \frac{1}{\sqrt{1 + \frac{1}{x}} + 1} = \frac{1}{2}

Commented by cherokeesay last updated on 07/Nov/22

v e r y n i c e,

t h a n k y o u s i r .

Answered by CElcedricjunior last updated on 07/Nov/22

\frac{1}{2}

Answered by mr W last updated on 07/Nov/22

= \lim_{x \to \infty} \frac{1}{\frac{1}{x}} (\sqrt{1 + \frac{1}{x}} - 1)

= \lim_{t \to 0} \frac{1}{t} (\sqrt{1 + t} - 1)

= \lim_{t \to 0} \frac{1}{t} (1 + \frac{t}{2} + o (t^{2}) - 1)

= \lim_{t \to 0} (\frac{1}{2} + o (t))

= \frac{1}{2}

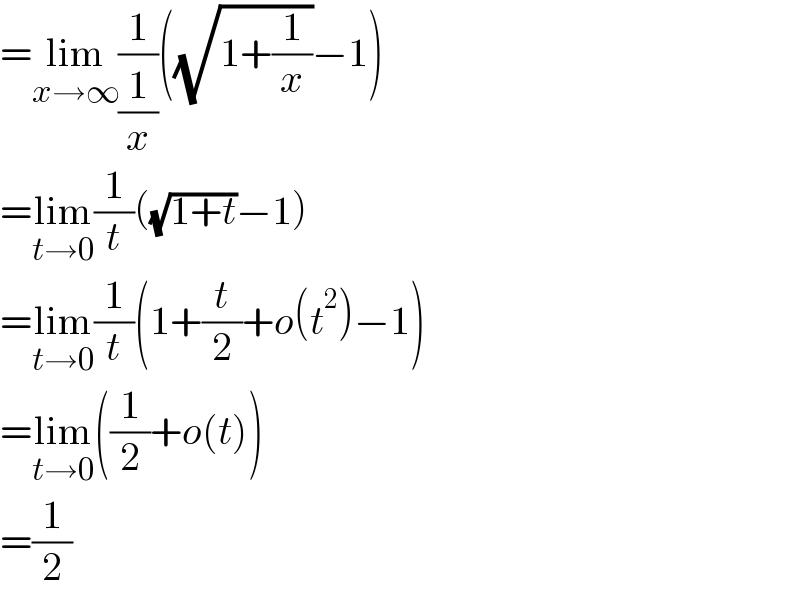
Commented by cherokeesay last updated on 07/Nov/22

N i c e, t h a n k y o u m a s t e r .