Question Number 119000 by bramlexs22 last updated on 21/Oct/20
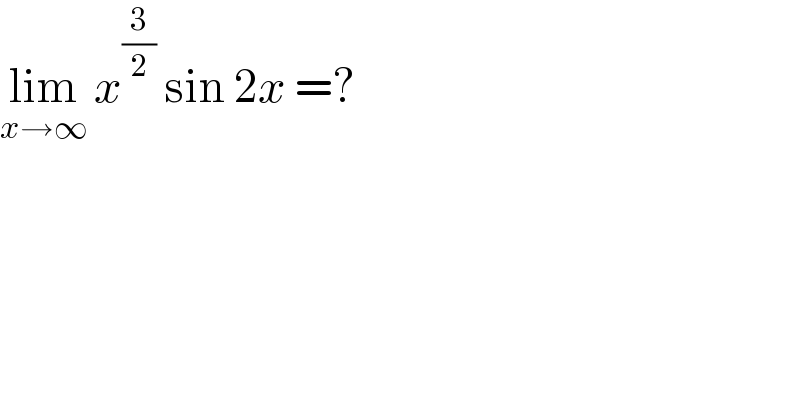
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:{x}^{\frac{\mathrm{3}}{\mathrm{2}}} \:\mathrm{sin}\:\mathrm{2}{x}\:=? \\ $$
Commented by MJS_new last updated on 21/Oct/20
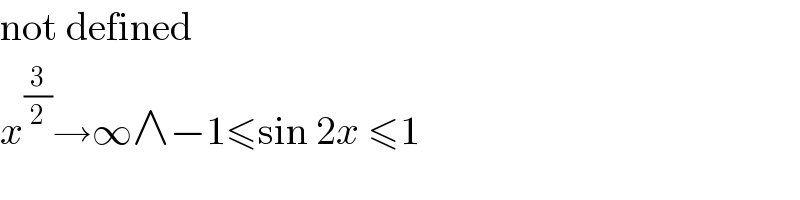
$$\mathrm{not}\:\mathrm{defined} \\ $$$${x}^{\frac{\mathrm{3}}{\mathrm{2}}} \rightarrow\infty\wedge−\mathrm{1}\leqslant\mathrm{sin}\:\mathrm{2}{x}\:\leqslant\mathrm{1} \\ $$
Answered by Bird last updated on 21/Oct/20
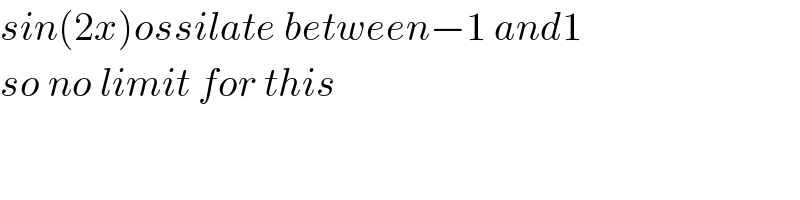
$${sin}\left(\mathrm{2}{x}\right){ossilate}\:{between}−\mathrm{1}\:{and}\mathrm{1} \\ $$$${so}\:{no}\:{limit}\:{for}\:{this} \\ $$
