Question Number 120593 by snipers237 last updated on 01/Nov/20
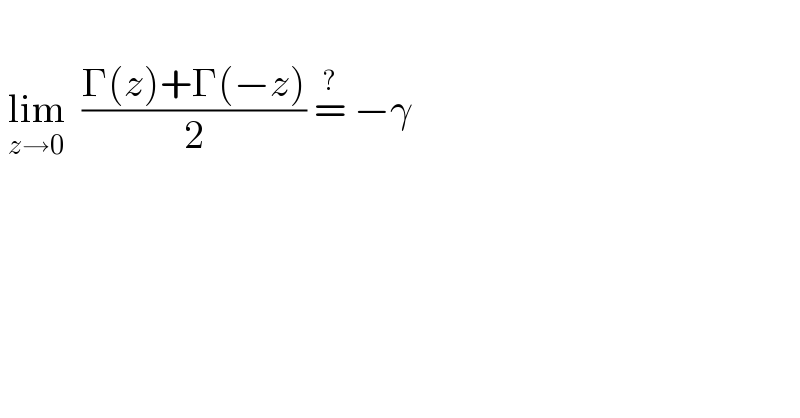
Answered by mnjuly1970 last updated on 02/Nov/20
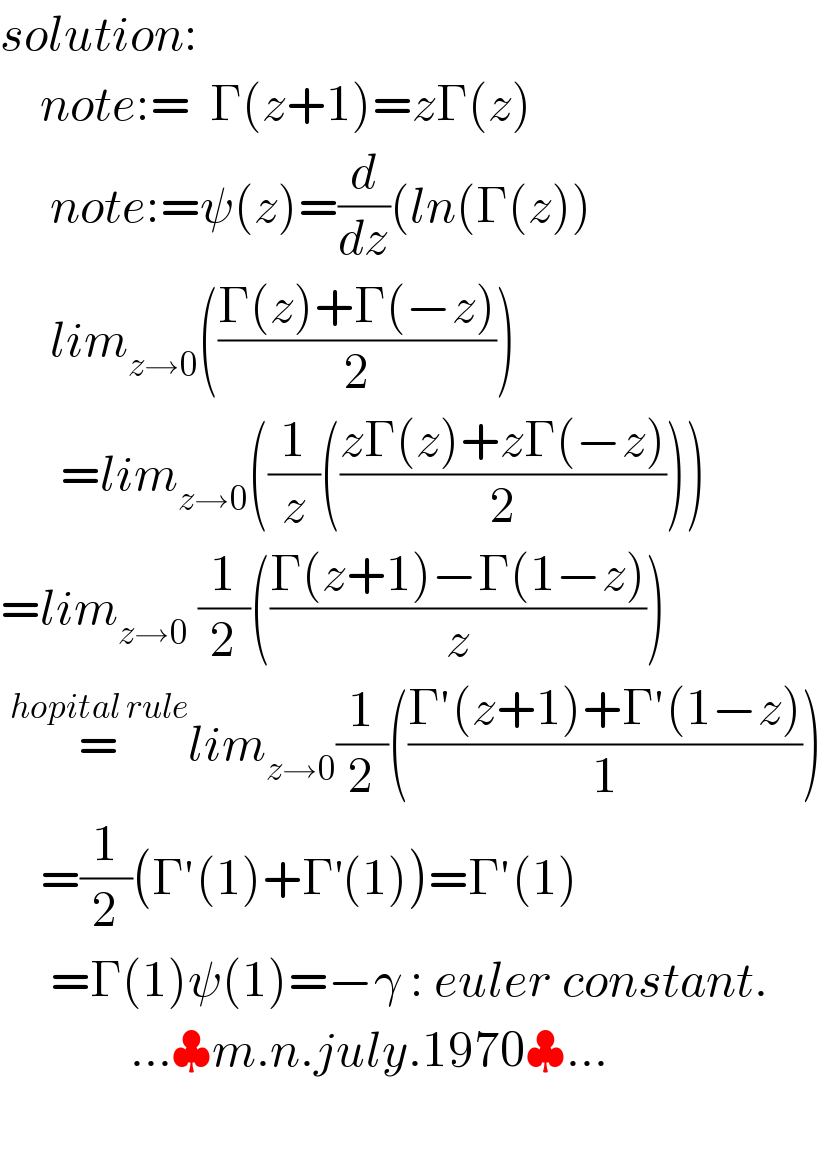
Commented by I want to learn more last updated on 02/Nov/20

Commented by I want to learn more last updated on 02/Nov/20

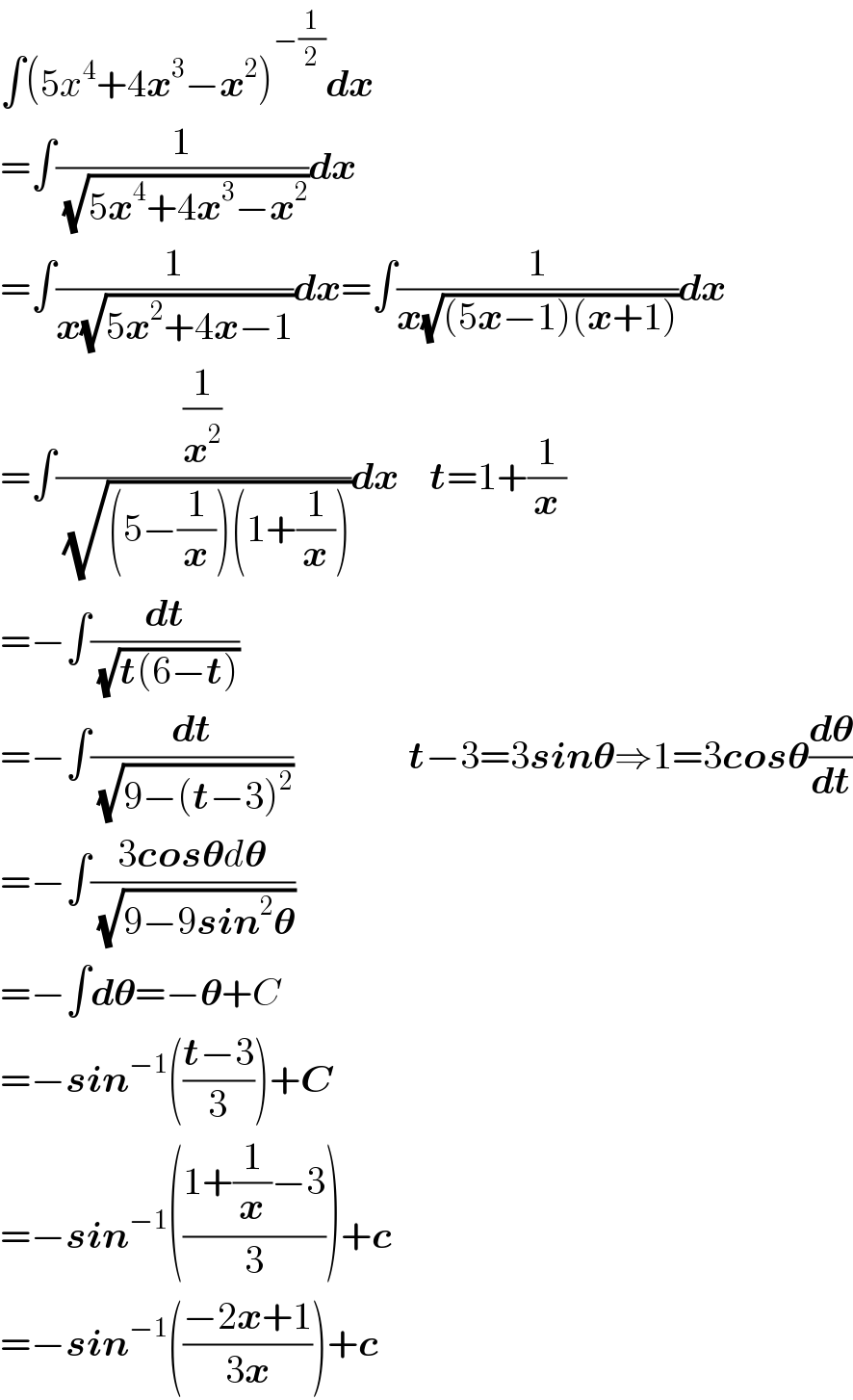
Commented by Dwaipayan Shikari last updated on 03/Nov/20
Commented by I want to learn more last updated on 03/Nov/20
Commented by Dwaipayan Shikari last updated on 03/Nov/20
Commented by I want to learn more last updated on 09/Nov/20

