Question Number 185374 by Mastermind last updated on 20/Jan/23
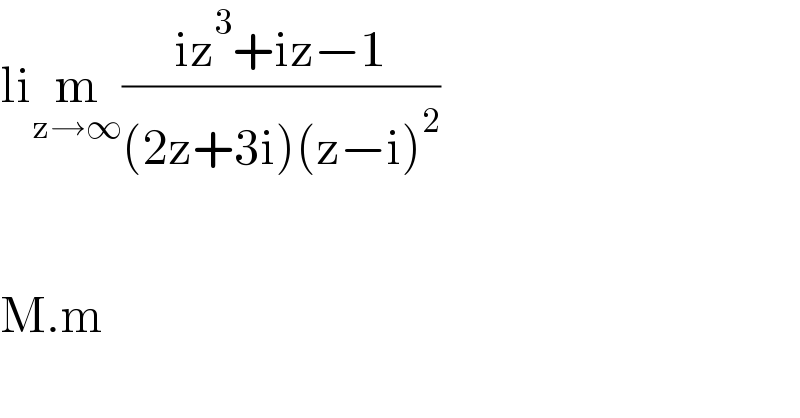
$$\mathrm{li}\underset{\mathrm{z}\rightarrow\infty} {\mathrm{m}}\frac{\mathrm{iz}^{\mathrm{3}} +\mathrm{iz}−\mathrm{1}}{\left(\mathrm{2z}+\mathrm{3i}\right)\left(\mathrm{z}−\mathrm{i}\right)^{\mathrm{2}} } \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$
Answered by MJS_new last updated on 20/Jan/23

$$\mathrm{if}\:{z}\rightarrow\infty\:\mathrm{means}\:{z}\rightarrow\infty+\mathrm{0i}\:\Rightarrow\:\mathrm{we}\:\mathrm{can}\:\mathrm{let}\:{z}\in\mathbb{R} \\ $$$$\mathrm{and}\:\mathrm{i}\:\mathrm{is}\:\mathrm{constant} \\ $$$$\frac{{cz}^{\mathrm{3}} +{cz}−\mathrm{1}}{\left(\mathrm{2}{z}+\mathrm{3}{c}\right)\left({z}−{c}\right)^{\mathrm{2}} }=\frac{{cz}^{\mathrm{3}} +{cz}−\mathrm{1}}{\mathrm{2}{z}^{\mathrm{3}} −{cz}^{\mathrm{2}} −\mathrm{4}{c}^{\mathrm{2}} {z}+\mathrm{3}{c}^{\mathrm{3}} } \\ $$$$\Rightarrow\:\mathrm{the}\:\mathrm{limit}\:\mathrm{is}\:\frac{{c}}{\mathrm{2}}=\frac{\mathrm{i}}{\mathrm{2}} \\ $$
Commented by Mastermind last updated on 21/Jan/23

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{so}\:\mathrm{much}\:\mathrm{boss} \\ $$
