Question Number 156779 by Fresnel last updated on 15/Oct/21

$$\int\frac{{ln}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$
Answered by phanphuoc last updated on 15/Oct/21

$${x}={tant} \\ $$
Answered by puissant last updated on 15/Oct/21
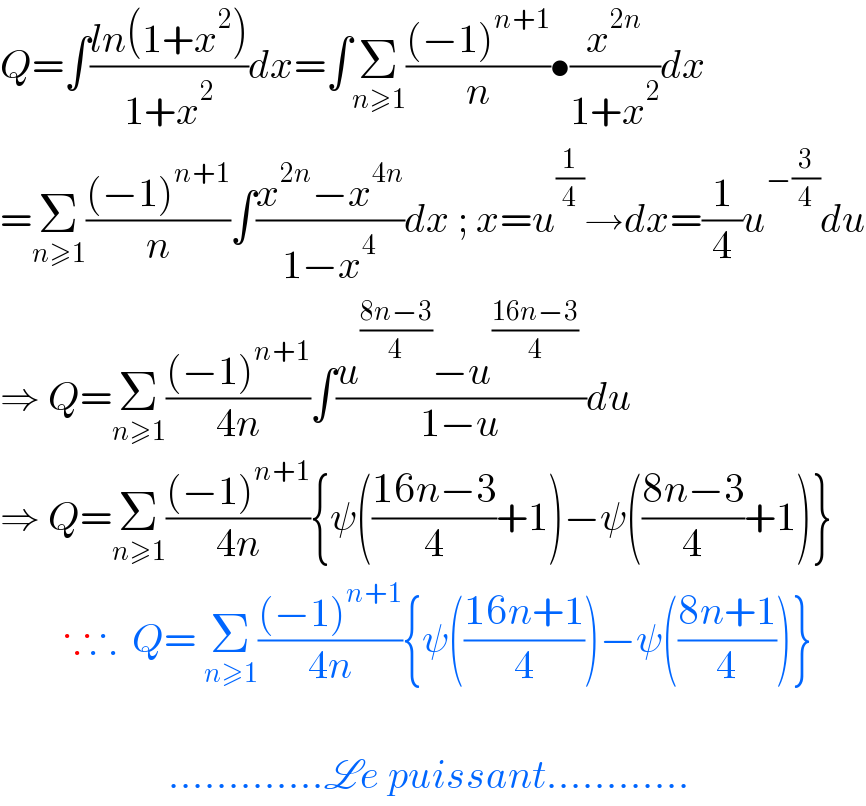
$${Q}=\int\frac{{ln}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\int\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{n}}\bullet\frac{{x}^{\mathrm{2}{n}} }{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$$=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{n}}\int\frac{{x}^{\mathrm{2}{n}} −{x}^{\mathrm{4}{n}} }{\mathrm{1}−{x}^{\mathrm{4}} }{dx}\:;\:{x}={u}^{\frac{\mathrm{1}}{\mathrm{4}}} \rightarrow{dx}=\frac{\mathrm{1}}{\mathrm{4}}{u}^{−\frac{\mathrm{3}}{\mathrm{4}}} {du} \\ $$$$\Rightarrow\:{Q}=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{4}{n}}\int\frac{{u}^{\frac{\mathrm{8}{n}−\mathrm{3}}{\mathrm{4}}} −{u}^{\frac{\mathrm{16}{n}−\mathrm{3}}{\mathrm{4}}} \:}{\mathrm{1}−{u}}{du} \\ $$$$\Rightarrow\:{Q}=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{4}{n}}\left\{\psi\left(\frac{\mathrm{16}{n}−\mathrm{3}}{\mathrm{4}}+\mathrm{1}\right)−\psi\left(\frac{\mathrm{8}{n}−\mathrm{3}}{\mathrm{4}}+\mathrm{1}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\because\therefore\:\:{Q}=\:\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{4}{n}}\left\{\psi\left(\frac{\mathrm{16}{n}+\mathrm{1}}{\mathrm{4}}\right)−\psi\left(\frac{\mathrm{8}{n}+\mathrm{1}}{\mathrm{4}}\right)\right\} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:………….\mathscr{L}{e}\:{puissant}………… \\ $$
Commented by Fresnel last updated on 15/Oct/21

$${Merci} \\ $$
Commented by Ruuudiy last updated on 17/Oct/21

$$\mathfrak{Thanks}\:\mathcal{S} \: \: \: \: \\ $$
