Menu Close

ln-sin-x-dx-

Tinku Tara 0 Comments
Pin




Question Number 114880 by manuel2456 last updated on 21/Sep/20
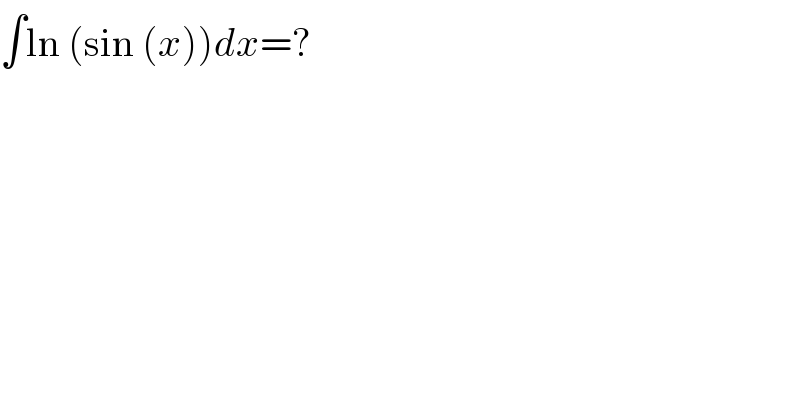
∫ln (sin (x))dx=?
$$\int\mathrm{ln}\:\left(\mathrm{sin}\:\left({x}\right)\right){dx}=? \\ $$
Commented by MJS_new last updated on 21/Sep/20
similar to question 113634
$$\mathrm{similar}\:\mathrm{to}\:\mathrm{question}\:\mathrm{113634} \\ $$
Answered by mathdave last updated on 23/Sep/20
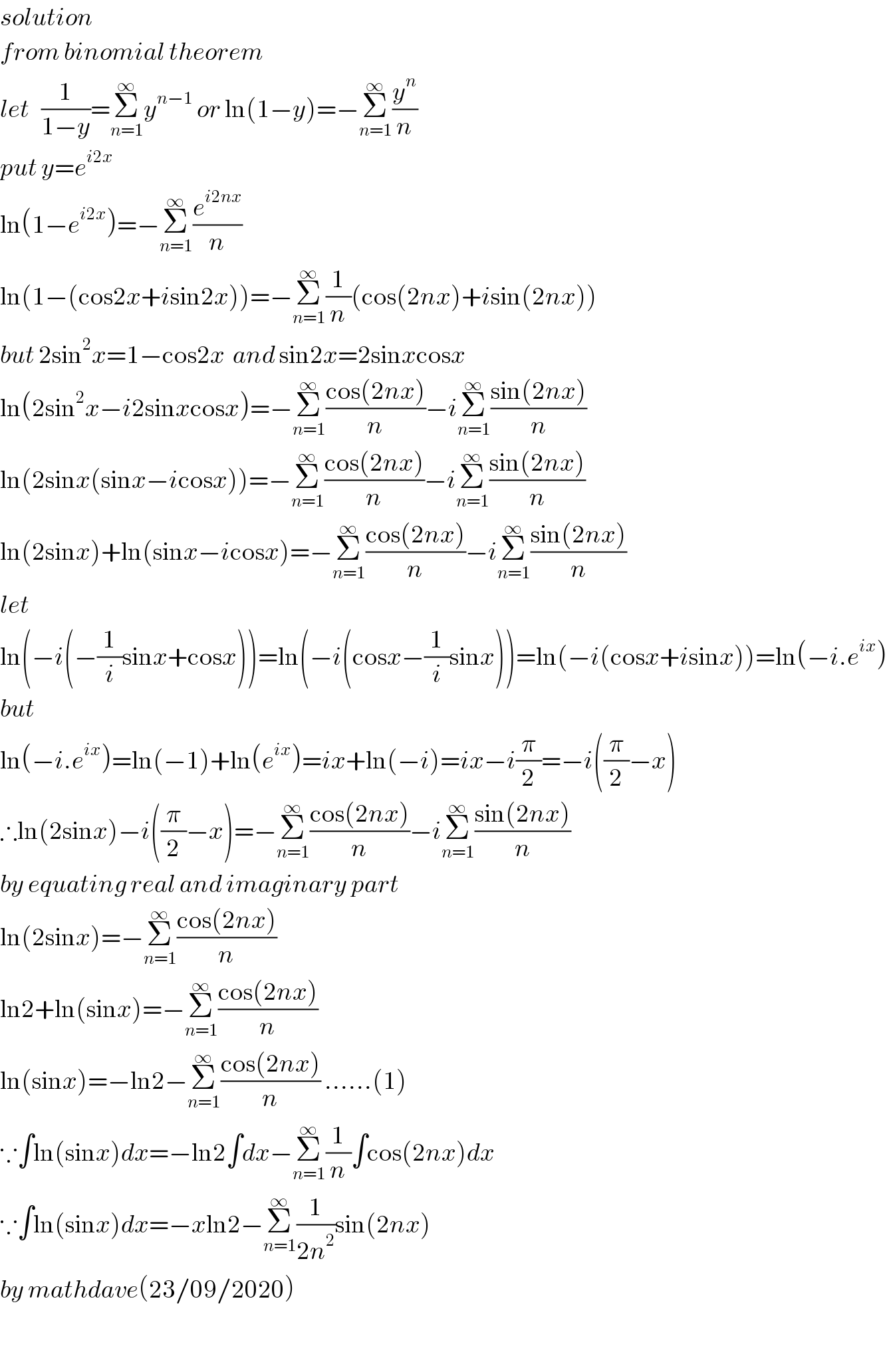
solution from binomial theorem let (1/(1−y))=Σ_(n=1) ^∞ y^(n−1) or ln(1−y)=−Σ_(n=1) ^∞ (y^n /n) put y=e^(i2x) ln(1−e^(i2x) )=−Σ_(n=1) ^∞ (e^(i2nx) /n) ln(1−(cos2x+isin2x))=−Σ_(n=1) ^∞ (1/n)(cos(2nx)+isin(2nx)) but 2sin^2 x=1−cos2x and sin2x=2sinxcosx ln(2sin^2 x−i2sinxcosx)=−Σ_(n=1) ^∞ ((cos(2nx))/n)−iΣ_(n=1) ^∞ ((sin(2nx))/n) ln(2sinx(sinx−icosx))=−Σ_(n=1) ^∞ ((cos(2nx))/n)−iΣ_(n=1) ^∞ ((sin(2nx))/n) ln(2sinx)+ln(sinx−icosx)=−Σ_(n=1) ^∞ ((cos(2nx))/n)−iΣ_(n=1) ^∞ ((sin(2nx))/n) let ln(−i(−(1/i)sinx+cosx))=ln(−i(cosx−(1/i)sinx))=ln(−i(cosx+isinx))=ln(−i.e^(ix) ) but ln(−i.e^(ix) )=ln(−1)+ln(e^(ix) )=ix+ln(−i)=ix−i(π/2)=−i((π/2)−x) ∴ln(2sinx)−i((π/2)−x)=−Σ_(n=1) ^∞ ((cos(2nx))/n)−iΣ_(n=1) ^∞ ((sin(2nx))/n) by equating real and imaginary part ln(2sinx)=−Σ_(n=1) ^∞ ((cos(2nx))/n) ln2+ln(sinx)=−Σ_(n=1) ^∞ ((cos(2nx))/n) ln(sinx)=−ln2−Σ_(n=1) ^∞ ((cos(2nx))/n) ......(1) ∵∫ln(sinx)dx=−ln2∫dx−Σ_(n=1) ^∞ (1/n)∫cos(2nx)dx ∵∫ln(sinx)dx=−xln2−Σ_(n=1) ^∞ (1/(2n^2 ))sin(2nx) by mathdave(23/09/2020)
$${solution}\: \\ $$$${from}\:{binomial}\:{theorem} \\ $$$${let}\:\:\:\frac{\mathrm{1}}{\mathrm{1}−{y}}=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{y}^{{n}−\mathrm{1}} \:{or}\:\mathrm{ln}\left(\mathrm{1}−{y}\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{y}^{{n}} }{{n}} \\ $$$${put}\:{y}={e}^{{i}\mathrm{2}{x}} \\ $$$$\mathrm{ln}\left(\mathrm{1}−{e}^{{i}\mathrm{2}{x}} \right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{e}^{{i}\mathrm{2}{nx}} }{{n}} \\ $$$$\mathrm{ln}\left(\mathrm{1}−\left(\mathrm{cos2}{x}+{i}\mathrm{sin2}{x}\right)\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}}\left(\mathrm{cos}\left(\mathrm{2}{nx}\right)+{i}\mathrm{sin}\left(\mathrm{2}{nx}\right)\right) \\ $$$${but}\:\mathrm{2sin}^{\mathrm{2}} {x}=\mathrm{1}−\mathrm{cos2}{x}\:\:{and}\:\mathrm{sin2}{x}=\mathrm{2sin}{x}\mathrm{cos}{x} \\ $$$$\mathrm{ln}\left(\mathrm{2sin}^{\mathrm{2}} {x}−{i}\mathrm{2sin}{x}\mathrm{cos}{x}\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\left(\mathrm{2}{nx}\right)}{{n}}−{i}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{sin}\left(\mathrm{2}{nx}\right)}{{n}} \\ $$$$\mathrm{ln}\left(\mathrm{2sin}{x}\left(\mathrm{sin}{x}−{i}\mathrm{cos}{x}\right)\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\left(\mathrm{2}{nx}\right)}{{n}}−{i}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{sin}\left(\mathrm{2}{nx}\right)}{{n}} \\ $$$$\mathrm{ln}\left(\mathrm{2sin}{x}\right)+\mathrm{ln}\left(\mathrm{sin}{x}−{i}\mathrm{cos}{x}\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\left(\mathrm{2}{nx}\right)}{{n}}−{i}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{sin}\left(\mathrm{2}{nx}\right)}{{n}} \\ $$$${let} \\ $$$$\mathrm{ln}\left(−{i}\left(−\frac{\mathrm{1}}{{i}}\mathrm{sin}{x}+\mathrm{cos}{x}\right)\right)=\mathrm{ln}\left(−{i}\left(\mathrm{cos}{x}−\frac{\mathrm{1}}{{i}}\mathrm{sin}{x}\right)\right)=\mathrm{ln}\left(−{i}\left(\mathrm{cos}{x}+{i}\mathrm{sin}{x}\right)\right)=\mathrm{ln}\left(−{i}.{e}^{{ix}} \right) \\ $$$${but} \\ $$$$\mathrm{ln}\left(−{i}.{e}^{{ix}} \right)=\mathrm{ln}\left(−\mathrm{1}\right)+\mathrm{ln}\left({e}^{{ix}} \right)={ix}+\mathrm{ln}\left(−{i}\right)={ix}−{i}\frac{\pi}{\mathrm{2}}=−{i}\left(\frac{\pi}{\mathrm{2}}−{x}\right) \\ $$$$\therefore\mathrm{ln}\left(\mathrm{2sin}{x}\right)−{i}\left(\frac{\pi}{\mathrm{2}}−{x}\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\left(\mathrm{2}{nx}\right)}{{n}}−{i}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{sin}\left(\mathrm{2}{nx}\right)}{{n}} \\ $$$${by}\:{equating}\:{real}\:{and}\:{imaginary}\:{part} \\ $$$$\mathrm{ln}\left(\mathrm{2sin}{x}\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\left(\mathrm{2}{nx}\right)}{{n}} \\ $$$$\mathrm{ln2}+\mathrm{ln}\left(\mathrm{sin}{x}\right)=−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\left(\mathrm{2}{nx}\right)}{{n}} \\ $$$$\mathrm{ln}\left(\mathrm{sin}{x}\right)=−\mathrm{ln2}−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\left(\mathrm{2}{nx}\right)}{{n}}\:……\left(\mathrm{1}\right)\:\: \\ $$$$\because\int\mathrm{ln}\left(\mathrm{sin}{x}\right){dx}=−\mathrm{ln2}\int{dx}−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}}\int\mathrm{cos}\left(\mathrm{2}{nx}\right){dx} \\ $$$$\because\int\mathrm{ln}\left(\mathrm{sin}{x}\right){dx}=−{x}\mathrm{ln2}−\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{2}{n}^{\mathrm{2}} }\mathrm{sin}\left(\mathrm{2}{nx}\right) \\ $$$${by}\:{mathdave}\left(\mathrm{23}/\mathrm{09}/\mathrm{2020}\right) \\ $$$$ \\ $$
Commented by Tawa11 last updated on 06/Sep/21
grest sir
$$\mathrm{grest}\:\mathrm{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *