Question Number 19738 by Tinkutara last updated on 15/Aug/17

Commented by math khazana by abdo last updated on 22/Jun/18
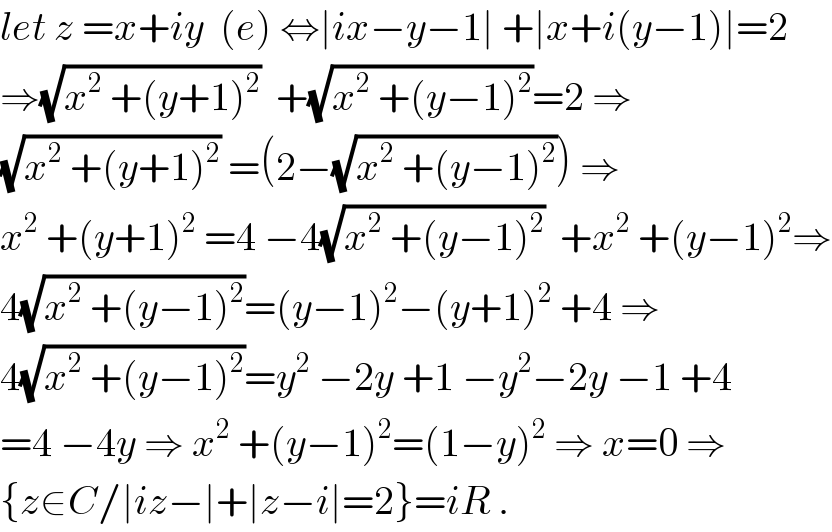
Answered by ajfour last updated on 15/Aug/17

Commented by ajfour last updated on 16/Aug/17

Commented by Tinkutara last updated on 16/Aug/17

Commented by Tinkutara last updated on 16/Aug/17

Commented by ajfour last updated on 16/Aug/17

