Menu Close

log-17-2-15-19-136-x-2-log-19-3-1-22-228-x-3-x-

Tinku Tara 0 Comments
Pin




Question Number 93957 by O Predador last updated on 16/May/20
log_((√(17))−(√2)) (((15)/( (√(19+(√(136)))))))x^2 − log_((√(19))−(√3)) ((1/(22−(√(228)))))x = 3 x = ?
$$\: \\ $$$$\:\mathrm{log}_{\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}} \left(\frac{\mathrm{15}}{\:\sqrt{\mathrm{19}+\sqrt{\mathrm{136}}}}\right)\mathrm{x}^{\mathrm{2}} \:−\:\mathrm{log}_{\sqrt{\mathrm{19}}−\sqrt{\mathrm{3}}} \left(\frac{\mathrm{1}}{\mathrm{22}−\sqrt{\mathrm{228}}}\right)\mathrm{x}\:=\:\mathrm{3} \\ $$$$\: \\ $$$$\:\mathrm{x}\:=\:? \\ $$
Commented by PRITHWISH SEN 2 last updated on 16/May/20
(√(19+(√(136)))) = (((√(34))+2)/( (√2))) ⇒((15(√2))/( (√(34))+2)) = (√(17))−(√2) 22−(√(228))=((√(19))−(√3))^2 log_((√(17))−(√2)) ((√(17))−(√2))x^2 −log_((√(19))−(√3)) x+2=3 2log_((√(17))−(√2)) x=log_((√(19))−(√3)) x and the only possible solution for this is x=1
$$\sqrt{\mathrm{19}+\sqrt{\mathrm{136}}}\:=\:\frac{\sqrt{\mathrm{34}}+\mathrm{2}}{\:\sqrt{\mathrm{2}}}\:\Rightarrow\frac{\mathrm{15}\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{34}}+\mathrm{2}}\:=\:\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}} \\ $$$$\mathrm{22}−\sqrt{\mathrm{228}}=\left(\sqrt{\mathrm{19}}−\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \\ $$$$\mathrm{log}_{\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}} \left(\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}\right)\mathrm{x}^{\mathrm{2}} −\mathrm{log}_{\sqrt{\mathrm{19}}−\sqrt{\mathrm{3}}} \mathrm{x}+\mathrm{2}=\mathrm{3} \\ $$$$\mathrm{2log}_{\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}} \mathrm{x}=\mathrm{log}_{\sqrt{\mathrm{19}}−\sqrt{\mathrm{3}}} \mathrm{x} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{only}\:\mathrm{possible}\:\mathrm{solution}\:\mathrm{for}\:\mathrm{this}\:\mathrm{is} \\ $$$$\boldsymbol{\mathrm{x}}=\mathrm{1} \\ $$
Commented by O Predador last updated on 25/May/20
Simplifying the radicals shouldn′t we consider it a second degree equation?
$$\:\mathrm{Simplifying}\:\:\mathrm{the}\:\:\mathrm{radicals}\:\:\mathrm{shouldn}'\mathrm{t}\:\:\mathrm{we}\:\:\mathrm{consider} \\ $$$$\:\mathrm{it}\:\mathrm{a}\:\:\mathrm{second}\:\:\mathrm{degree}\:\:\mathrm{equation}? \\ $$
Commented by O Predador last updated on 25/May/20
Simplificando os radicais na^� o deve-se considerar uma equac_� a^� o do segundo grau?
$$\:\mathrm{Simplificando}\:\:\mathrm{os}\:\:\mathrm{radicais}\:\:\mathrm{n}\bar {\mathrm{a}o}\:\:\mathrm{deve}-\mathrm{se}\:\:\mathrm{considerar} \\ $$$$\:\mathrm{uma}\:\:\mathrm{equa}\underset{} {\mathrm{c}}\bar {\mathrm{a}o}\:\:\mathrm{do}\:\:\mathrm{segundo}\:\:\mathrm{grau}? \\ $$
Commented by PRITHWISH SEN 2 last updated on 25/May/20
English please
$$\mathrm{English}\:\mathrm{please} \\ $$
Commented by PRITHWISH SEN 2 last updated on 25/May/20
Actually 19+(√(136)) = ((38+2(√(136)))/2) = ((((√(34)))^2 +2^2 +2.(√(34)).2)/2) =((((√(34)))^2 +2^2 +2.(√(34)).(√4))/2) = ((((√(34))+2)^2 )/(((√2))^2 )) I think this will help you.
$$\mathrm{Actually} \\ $$$$\mathrm{19}+\sqrt{\mathrm{136}}\:=\:\frac{\mathrm{38}+\mathrm{2}\sqrt{\mathrm{136}}}{\mathrm{2}}\:=\:\frac{\left(\sqrt{\mathrm{34}}\right)^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +\mathrm{2}.\sqrt{\mathrm{34}}.\mathrm{2}}{\mathrm{2}} \\ $$$$=\frac{\left(\sqrt{\mathrm{34}}\right)^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +\mathrm{2}.\sqrt{\mathrm{34}}.\sqrt{\mathrm{4}}}{\mathrm{2}}\:=\:\frac{\left(\sqrt{\mathrm{34}}+\mathrm{2}\right)^{\mathrm{2}} }{\left(\sqrt{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$$$\mathrm{I}\:\mathrm{think}\:\mathrm{this}\:\mathrm{will}\:\mathrm{help}\:\mathrm{you}. \\ $$
Commented by O Predador last updated on 26/May/20
Grades: { (((√(19+(√(136)))) = (√(17))+(√2))),((22−(√(228)) = ((√(19))−(√3))^2 )) :} log_((√(17))−(√2)) ((√(17))−(√2))x^2 −log_((√(19))−(√3)) ((1/(((√(19))−(√3))^2 )))x = 3 ... ... x^2 +2x = 3 ... ... x^2 +2x+1 = 3+1 ... ... x+1 = ±2 ... { ((x_1 =−3)),((x_2 =1)) :} Solution: {−3, 1} Wouldn′t that be so?
$$\: \\ $$$$\:\mathrm{Grades}:\:\:\begin{cases}{\sqrt{\mathrm{19}+\sqrt{\mathrm{136}}}\:=\:\sqrt{\mathrm{17}}+\sqrt{\mathrm{2}}}\\{\mathrm{22}−\sqrt{\mathrm{228}}\:=\:\left(\sqrt{\mathrm{19}}−\sqrt{\mathrm{3}}\right)^{\mathrm{2}} }\end{cases} \\ $$$$\: \\ $$$$\:\mathrm{log}_{\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}} \left(\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}\right)\mathrm{x}^{\mathrm{2}} −\mathrm{log}_{\sqrt{\mathrm{19}}−\sqrt{\mathrm{3}}} \left(\frac{\mathrm{1}}{\left(\sqrt{\mathrm{19}}−\sqrt{\mathrm{3}}\right)^{\mathrm{2}} }\right)\mathrm{x}\:=\:\mathrm{3}\:… \\ $$$$\: \\ $$$$\:…\:\mathrm{x}^{\mathrm{2}} +\mathrm{2x}\:=\:\mathrm{3}\:… \\ $$$$\:…\:\mathrm{x}^{\mathrm{2}} +\mathrm{2x}+\mathrm{1}\:=\:\mathrm{3}+\mathrm{1}\:… \\ $$$$\:…\:\mathrm{x}+\mathrm{1}\:=\:\pm\mathrm{2}\:… \\ $$$$\: \\ $$$$\:\begin{cases}{\mathrm{x}_{\mathrm{1}} =−\mathrm{3}}\\{\mathrm{x}_{\mathrm{2}} =\mathrm{1}}\end{cases} \\ $$$$\: \\ $$$$\:\mathrm{Solution}:\:\left\{−\mathrm{3},\:\mathrm{1}\right\} \\ $$$$\: \\ $$$$\:\mathrm{Wouldn}'\mathrm{t}\:\:\mathrm{that}\:\:\mathrm{be}\:\:\mathrm{so}? \\ $$
Commented by PRITHWISH SEN 2 last updated on 26/May/20
no. log_((√(17))−(√2)) ((√(17))−(√2))x^2 = log_((√(17))−(√2)) ((√(17))−(√2))+log_((√(17))−(√2)) x^2
$$\mathrm{no}. \\ $$$$\mathrm{log}_{\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}} \left(\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}\right)\mathrm{x}^{\mathrm{2}} =\:\mathrm{log}_{\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}} \left(\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}\right)+\mathrm{log}_{\sqrt{\mathrm{17}}−\sqrt{\mathrm{2}}} \mathrm{x}^{\mathrm{2}} \\ $$
Commented by PRITHWISH SEN 2 last updated on 26/May/20
no. because log_a bc = log_a b + log_a c
$$\mathrm{no}. \\ $$$$\mathrm{because}\:\mathrm{log}_{\mathrm{a}} \mathrm{bc}\:=\:\mathrm{log}_{\mathrm{a}} \mathrm{b}\:+\:\mathrm{log}_{\mathrm{a}} \mathrm{c} \\ $$
Commented by O Predador last updated on 26/May/20
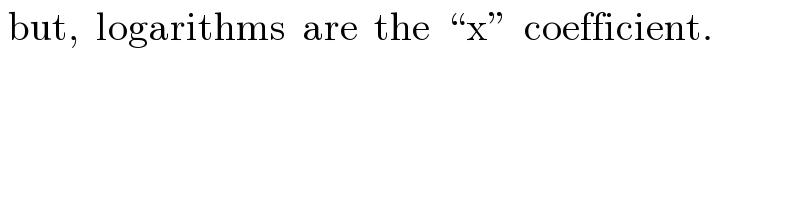
but, logarithms are the “x” coefficient.
$$\:\mathrm{but},\:\:\mathrm{logarithms}\:\:\mathrm{are}\:\:\mathrm{the}\:\:“\mathrm{x}''\:\:\mathrm{coefficient}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *