log-2-x-log-3-x-1-x-

Question Number 91862 by jagoll last updated on 03/May/20

\log_{2} (x) + \log_{3} (x) = 1

x = ?

Commented by Tony Lin last updated on 03/May/20

\frac{l n x}{l n 2} + \frac{l n x}{l n 3} = 1

l n x (\frac{l n 6}{l n 2 \times l n 3}) = 1

l n x = \frac{l n 2 \times l n 3}{l n 6}

x = e^{\frac{l n 2 \times l n 3}{l n 6}} \approx 1.53

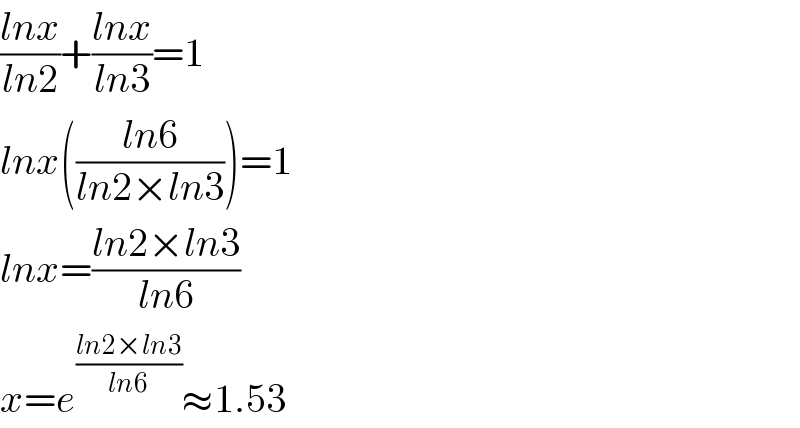
Commented by jagoll last updated on 03/May/20

��

Commented by hmamarques1994@gmail.com last updated on 03/May/20

x = 2^{{l o g}_{6} 3} \approx 1, 52959

Commented by john santu last updated on 03/May/20

2^{\log_{6} (3)} = 3^{\log_{6} (2)}

Answered by john santu last updated on 03/May/20

Commented by john santu last updated on 03/May/20

��

Commented by john santu last updated on 03/May/20

1.52959232849

Commented by hmamarques1994@gmail.com last updated on 03/May/20

G \hat{e n i o}!