Question Number 113800 by Aina Samuel Temidayo last updated on 15/Sep/20
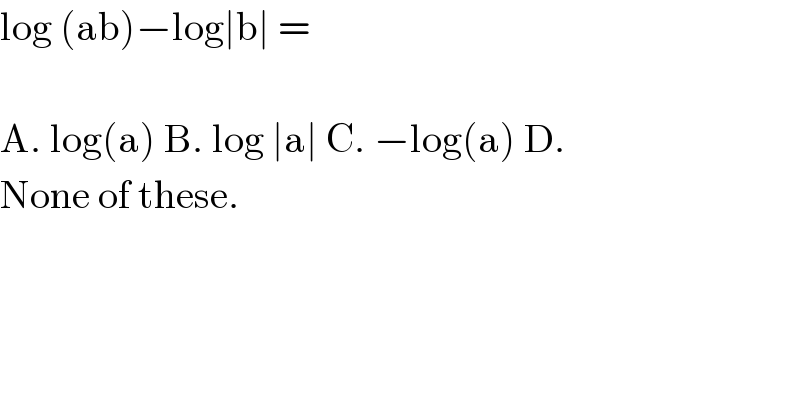
Answered by MJS_new last updated on 15/Sep/20
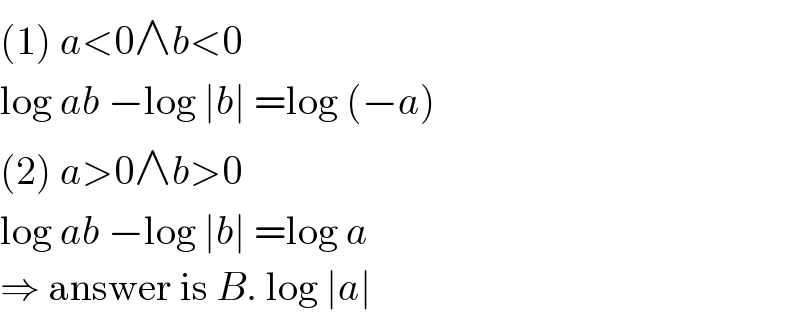
Commented by MJS_new last updated on 15/Sep/20
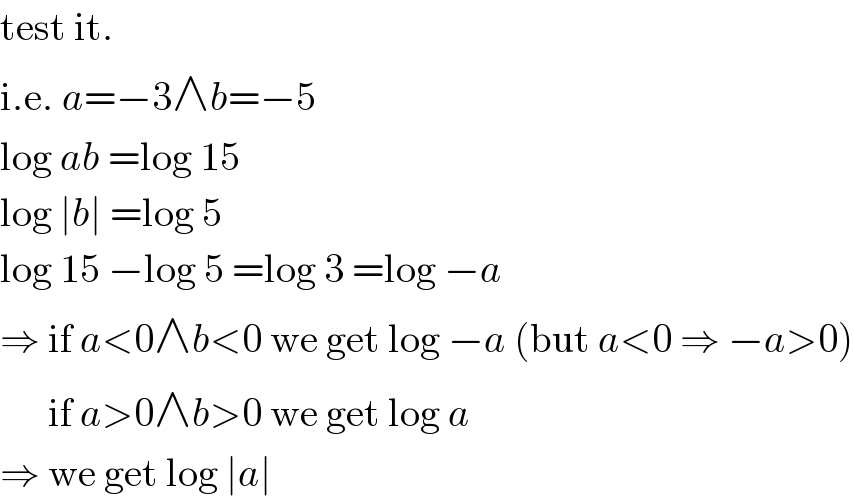
Commented by Aina Samuel Temidayo last updated on 15/Sep/20

Commented by Aina Samuel Temidayo last updated on 15/Sep/20
Commented by Aina Samuel Temidayo last updated on 15/Sep/20
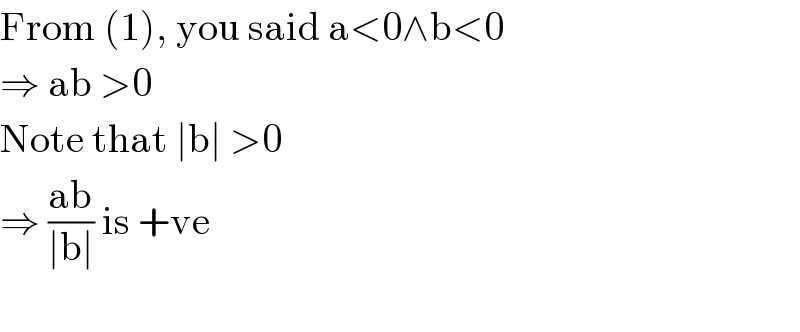
Commented by MJS_new last updated on 15/Sep/20
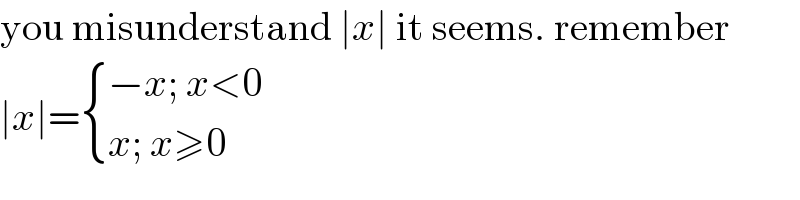
Commented by Aina Samuel Temidayo last updated on 15/Sep/20
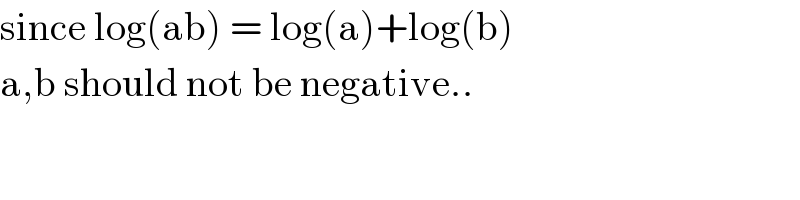
Commented by MJS_new last updated on 15/Sep/20
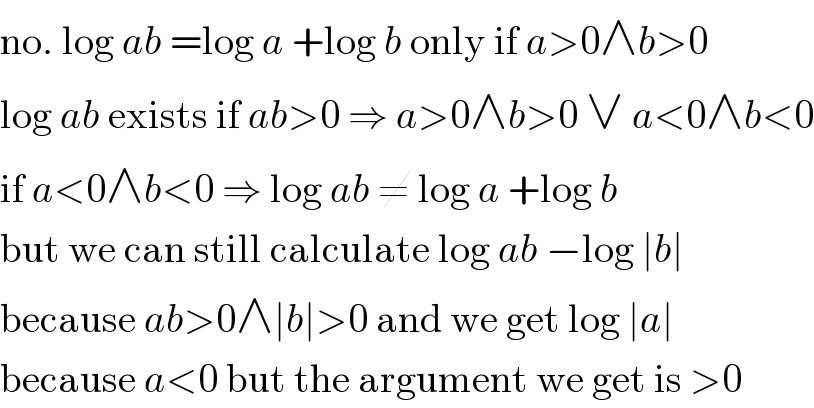
Commented by Aina Samuel Temidayo last updated on 15/Sep/20

