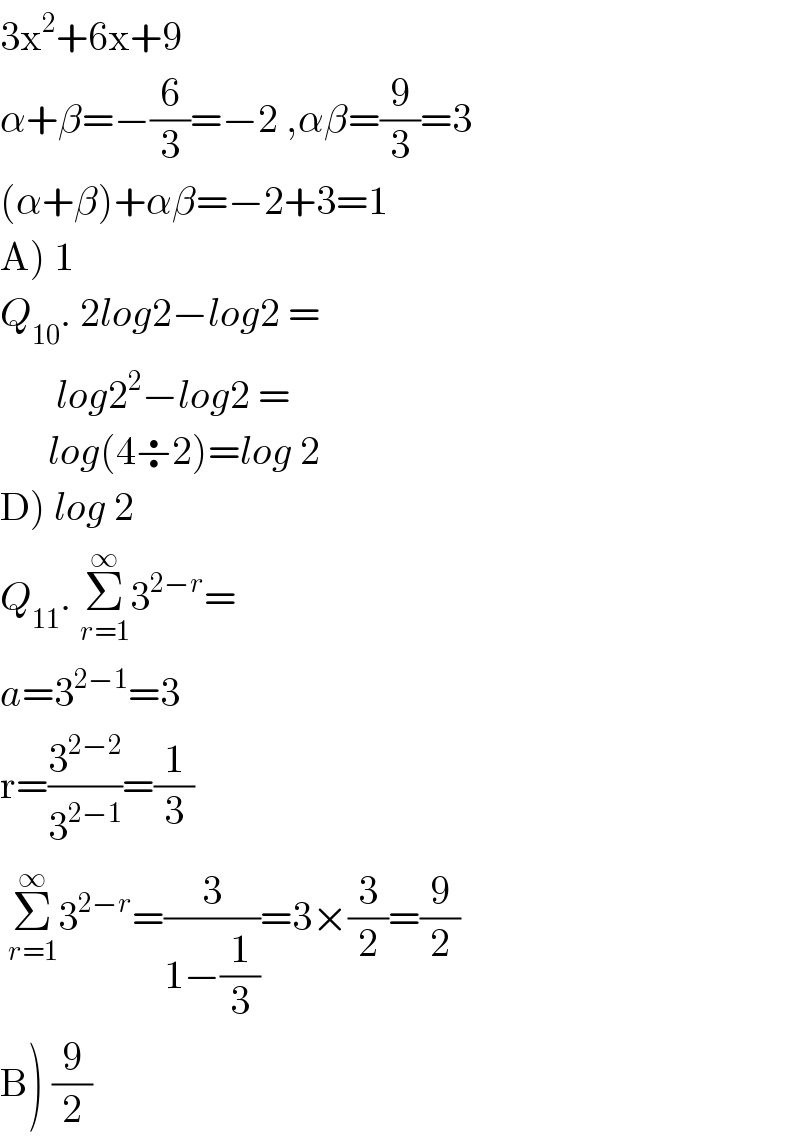Multiple-Choice-Questions-Rio-Mike-Q-1-The-value-of-6-2-4-15-3-is-A-3-B-9-C-4-D-27-Q-2-80-as-a-product-of-its-prime-factor-is-A-2-3-5-B-2-5-3-C-2-2-5-2-D-2-4-

Question Number 35811 by Rio Mike last updated on 23/May/18

M u l t i p l e C h o i c e Q u e s t i o n s

R i o M i k e .

Q_{1} . T h e v a l u e o f 6 + 2 \times 4 - 15 \div 3

i s;

A) 3 B) 9 C) 4 D) 27

Q_{2} . 80 a s a p r o d u c t o f i t s p r i m e

f a c t o r i s .

A) 2^{3} \times 5 B) 2 \times 5^{3} C) 2^{2} \times 5^{2}

D) 2^{4} \times 5

Q_{3} . T h e d e t e m i n a n t o f (\begin{matrix} 6 4 \\ 3 2 \end{matrix}) i s

A) 18 B) 0 C) 24 D) - 24

Q_{4} . t h e v a l u e o f {(\frac{1}{16})}^{- \frac{1}{2}} i s

A) - \frac{1}{4} B) 4 C) - 4 D) \frac{1}{2}

Q_{5} . i f - 8, m, n, 19 a r e i n A P t h e n

(m, n) i s

A) (1, 10) B) (2, 10) C) (3, 13) D) (4, 16) o

Q_{6} . i f c o s θ = \frac{12}{13} t h e n {c o t}^{2} θ i s;

A) \frac{169}{25} B) \frac{25}{169} C) \frac{169}{144} D) \frac{144}{169}

Q_{7} . t h e s o l u t i o n s e t o f 3 ⩽ 2 x - 1 ⩽ 5

i s;

A)] x ⩾ - 2, x ⩽ \frac{8}{3} [B) [x ⩾ 2, x ⩽ - \frac{8}{3} [

C)] x > - 2, x ⩽ \frac{3}{8}] D) [x ⩾ - 2, x ⩽ \frac{8}{3}]

Q_{8} . W h i c h i s a f a c t o r o f f (x) =

x^{2} - 3 x + 2

A) (x - 1) B) (x - 2) C) (x + 1) D) (x + 3)

Q_{9} . T h e s u m o f t h e s u m o f

r o o t s a n d p r o d u c t o f r o o t s o f t h e

q u a d r a t i c e q u a t i o n 3 x^{2} + 6 x + 9 = 0

A) 1 B) - 1 C) 32 D) 5

Q_{10} . 2 l o g 2 - l o g 2 =

A) l o g 8 B) l o g 6 C) l o g 3

D) l o g 2 .

Q_{11} . \underset{r = 1}{\sum^{\infty}} 3^{2 - r} =

A) \frac{9}{4} B) \frac{9}{2} C) \frac{13}{3} D) \frac{1}{2}

Q_{12} . T h e c o n s t a n d t e r m i n t h e

b i n o m i a l e x p a n s i o n o f {(x^{2} + \frac{1}{x^{2}})}^{8} i s

A) 3^{r d} B) 4^{t h} C) 5^{t h} D) 6^{t h}

Q_{13} . c o s (180 - x) =

A) - s i n x B) s i n x C) c o s x

D) - c o s x

Q_{14} . T h e v a l u e o f p f o r w h i c h

(2, 1), (6, 3), a n d (4, p) a r e c o l l i n e a r

i s;

A) 2 B) 1 C) - 1 D) - 2

Q_{15} . \int_{1}^{2} x^{3} d x =

A) - s i n 7 x B) s i n 7 x C) - 7 s i n 7 x

D) 7 s i n 7 x

Q_{16} . G i v e n t h a t g : \to p x - 5, t h e

v a l u e o f p f o r w h i c h g^{- 1} (3) = 4 i s

A) \frac{1}{2} B) - \frac{1}{2} C) - 2 D) 2

Q_{17} . T h e v a l u e o f θ i n t h e r a n g e

0 ° ⩽ θ ⩽ 90 ° f o r w h i c h s i n θ = c o s θ i s

A) 90 ° B) 60 ° C) 30 ° D) 45 °

Q u e s t i o n s

Commented by Rasheed.Sindhi last updated on 24/May/18

How did you upload so large image ?!

Commented by Joel579 last updated on 24/May/18

Actually he typed it, not uploaded it

Commented by Rasheed.Sindhi last updated on 24/May/18

Sir I misunderstood . But his other

uploaded lengthy post is an image .

Commented by Rio Mike last updated on 24/May/18

yeah your right . i typed this but

the other was uploaded a s a n i m a g e

Commented by Rasheed.Sindhi last updated on 25/May/18

Questioners are requested to send at most

(3 short questions) / post

or

(1 long question) / post

{It}^{'} s better practice, I think .

Commented by rahul 19 last updated on 26/May/18

I a g r e e .

Answered by Rasheed.Sindhi last updated on 25/May/18

Q_{1}

6 + 2 \times 4 - 15 \div 3

= 6 + 8 - 5 = 9

B) 9

Q_{2}

D) 2^{4} . 5

Q_{3} T h e d e t e m i n a n t o f (\begin{matrix} 6 4 \\ 3 2 \end{matrix})

6 \times 2 - 3 \times 4 = 0

B) 0

Q_{4} {(\frac{1}{16})}^{- \frac{1}{2}}

= \frac{1}{{(4^{2})}^{- \frac{1}{2}}} = \frac{1}{4^{- 1}} = 4

B) 4

Q_{5} - 8, m, n, 19 are in AP

First term = a = - 8, Common difference = d

n t h term = a_{n} = a + (n - 1) d

a_{4} = - 8 + (4 - 1) d = 19

3 d = 19 + 8 = 27

d = 9

m = - 8 + 9 = 1, n = 1 + 9 = 10

(m, n) = (1, 10)

A) (1, 10)

Answered by Rasheed.Sindhi last updated on 25/May/18

\cos θ = \frac{12}{13}

\cos^{2} θ + \sin^{2} θ = 1

\frac{\cos^{2} θ}{\sin^{2} θ} + 1 = \frac{1}{\sin^{2} θ}

\cot^{2} θ + 1 = \frac{1}{1 - \cos^{2} θ} = \frac{1}{1 - {(\frac{12}{13})}^{2}}

\cot^{2} θ = \frac{1}{\frac{13^{2} - 12^{2}}{13^{2}}} - 1

= \frac{13^{2}}{13^{2} - 12^{2}} - 1 = \frac{144}{25}

D) \frac{144}{25}

Q_{7} 3 ⩽ 2 x - 1 ⩽ 5

4 ⩽ 2 x ⩽ 6

2 ⩽ x ⩽ 3

Neither option is correct!

Q_{8} Factor of x^{2} - 3 x + 2

x^{2} - 2 x - x + 2

x (x - 2) - 1 (x - 2)

(x - 1) (x - 2)

Two options are correct ?!

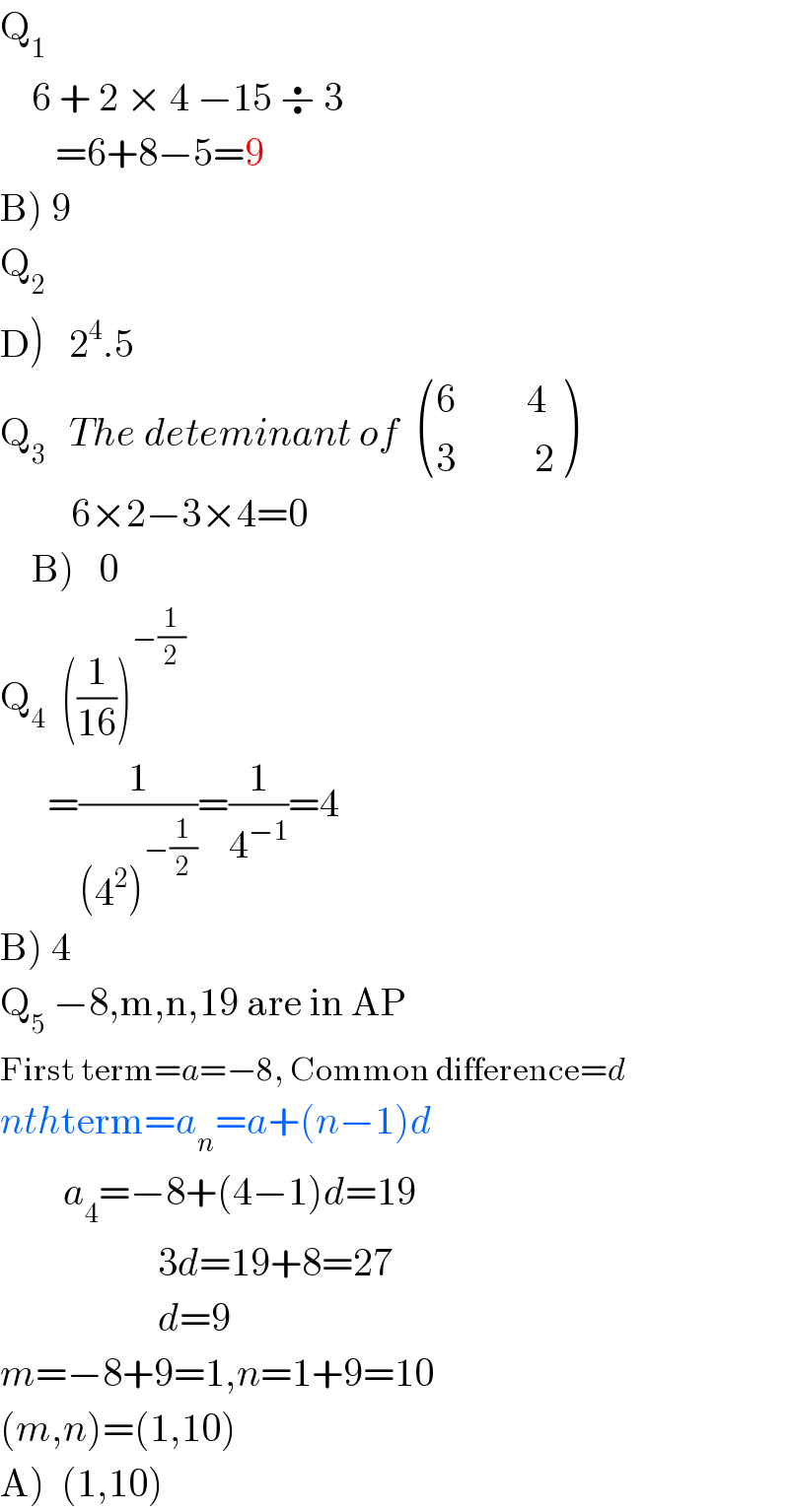
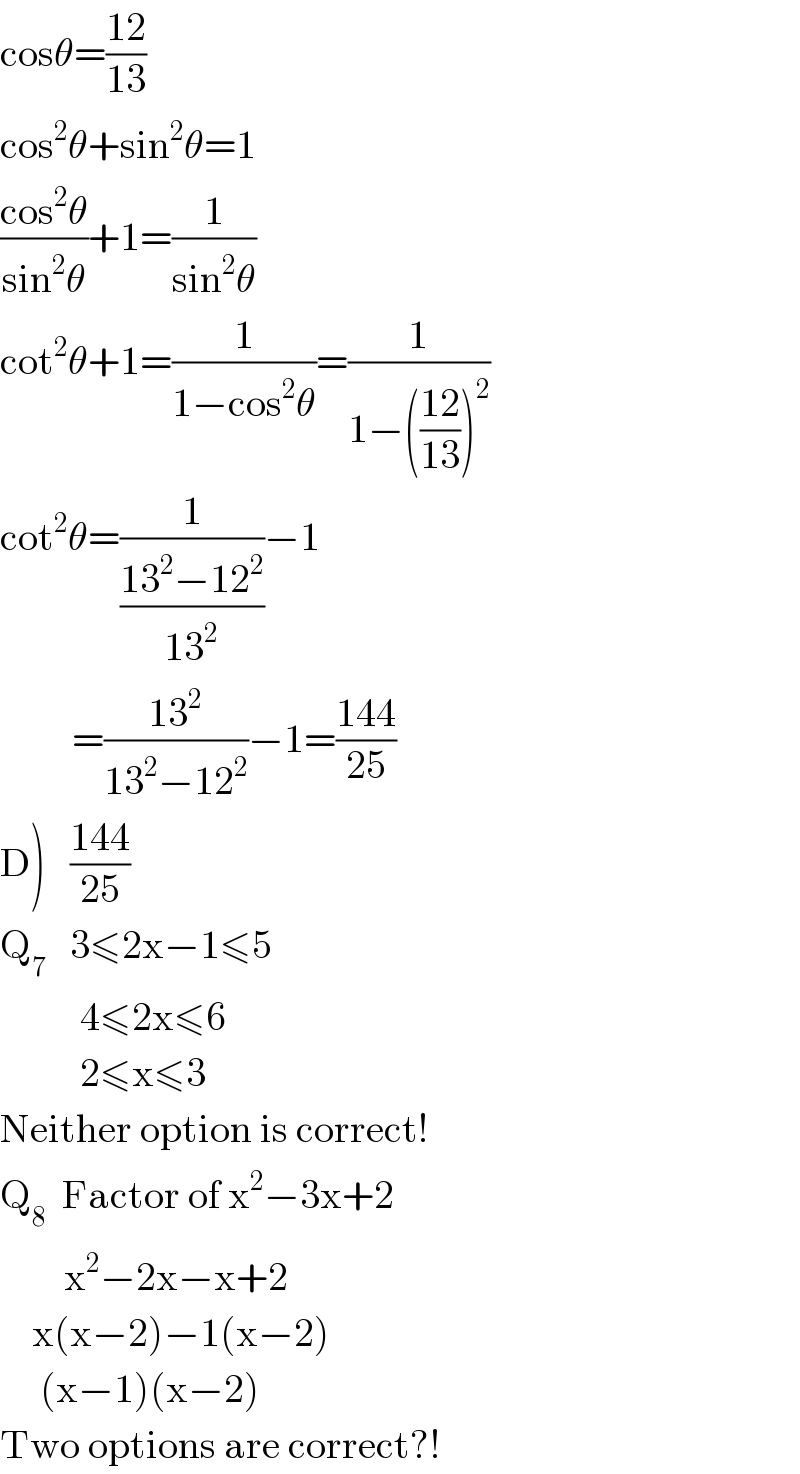
Answered by Rasheed.Sindhi last updated on 26/May/18

{3 x}^{2} + 6 x + 9

α + β = - \frac{6}{3} = - 2, α β = \frac{9}{3} = 3

(α + β) + α β = - 2 + 3 = 1

A) 1

Q_{10} . 2 l o g 2 - l o g 2 =

l o g 2^{2} - l o g 2 =

l o g (4 \div 2) = l o g 2

D) l o g 2

Q_{11} . \underset{r = 1}{\sum^{\infty}} 3^{2 - r} =

a = 3^{2 - 1} = 3

r = \frac{3^{2 - 2}}{3^{2 - 1}} = \frac{1}{3}

\underset{r = 1}{\sum^{\infty}} 3^{2 - r} = \frac{3}{1 - \frac{1}{3}} = 3 \times \frac{3}{2} = \frac{9}{2}

B) \frac{9}{2}