Question Number 32755 by 7991 last updated on 01/Apr/18
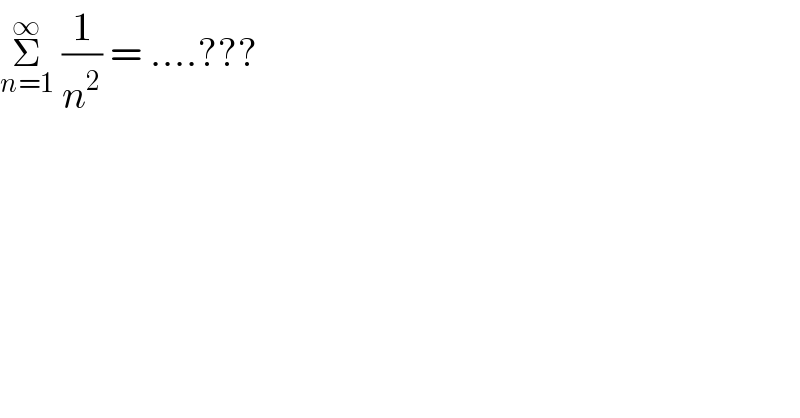
Commented by Rio Mike last updated on 01/Apr/18
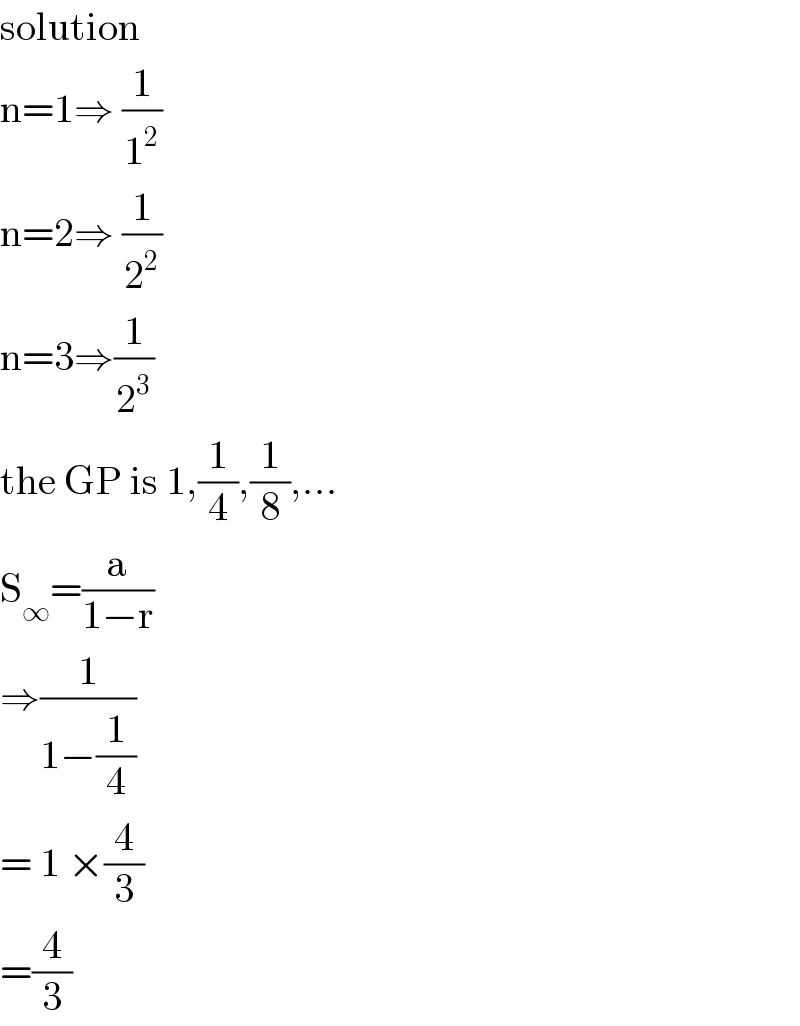
Commented by abdo imad last updated on 01/Apr/18
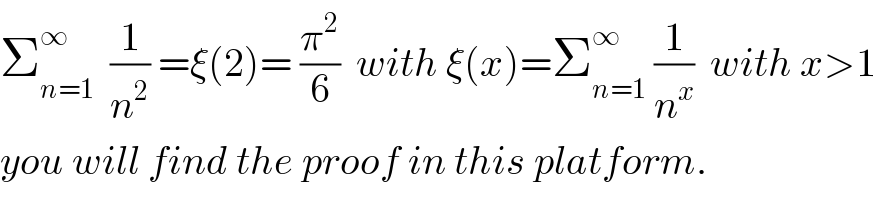
Commented by abdo imad last updated on 01/Apr/18
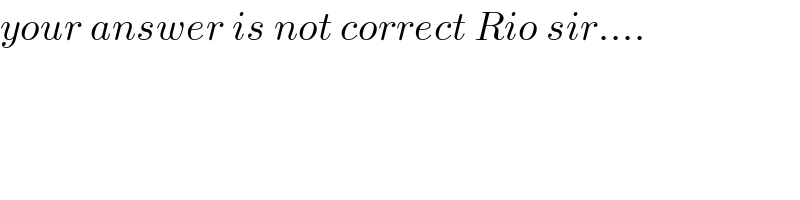
Commented by JDamian last updated on 05/Apr/18
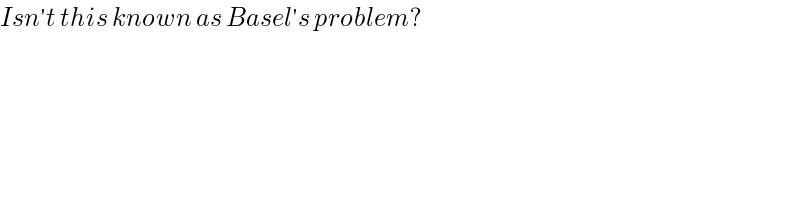
Commented by Rasheed.Sindhi last updated on 05/Apr/18

