n-1-1-n-2n-1-

Question Number 150059 by mathdanisur last updated on 09/Aug/21

$$\underset{\boldsymbol{\mathrm{n}}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\mathrm{1}}{\mathrm{n}\centerdot\left(\mathrm{2n}\:+\:\mathrm{1}\right)}\:=\:? \\ $$

Answered by Kamel last updated on 09/Aug/21

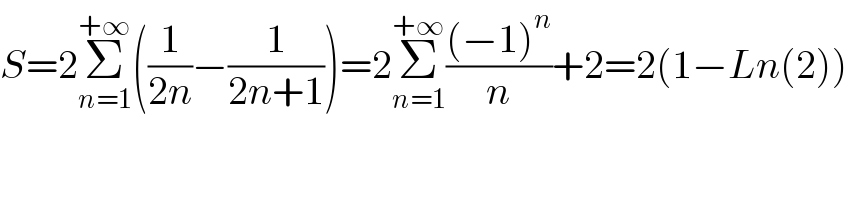
S=2Σ_(n=1) ^(+∞) ((1/(2n))−(1/(2n+1)))=2Σ_(n=1) ^(+∞) (((−1)^n )/n)+2=2(1−Ln(2))

$${S}=\mathrm{2}\underset{{n}=\mathrm{1}} {\overset{+\infty} {\sum}}\left(\frac{\mathrm{1}}{\mathrm{2}{n}}−\frac{\mathrm{1}}{\mathrm{2}{n}+\mathrm{1}}\right)=\mathrm{2}\underset{{n}=\mathrm{1}} {\overset{+\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}}+\mathrm{2}=\mathrm{2}\left(\mathrm{1}−{Ln}\left(\mathrm{2}\right)\right) \\ $$

Commented by mathdanisur last updated on 09/Aug/21

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{Ser} \\ $$

Answered by Ar Brandon last updated on 09/Aug/21

S=Σ_(n=1) ^∞ (1/(n(2n+1)))=Σ_(n=1) ^∞ ((1/n)−(2/(2n+1))) =Σ_(n=1) ^∞ ((1/n)−(1/(n+(1/2))))=ψ((3/2))−ψ(1) =2+ψ((1/2))−ψ(1)=2−γ−2ln2+γ=2−ln4

$${S}=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}\left(\mathrm{2}{n}+\mathrm{1}\right)}=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{{n}}−\frac{\mathrm{2}}{\mathrm{2}{n}+\mathrm{1}}\right) \\ $$$$\:\:\:=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{{n}}−\frac{\mathrm{1}}{{n}+\frac{\mathrm{1}}{\mathrm{2}}}\right)=\psi\left(\frac{\mathrm{3}}{\mathrm{2}}\right)−\psi\left(\mathrm{1}\right) \\ $$$$\:\:\:=\mathrm{2}+\psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\psi\left(\mathrm{1}\right)=\mathrm{2}−\gamma−\mathrm{2ln2}+\gamma=\mathrm{2}−\mathrm{ln4} \\ $$

Commented by Ar Brandon last updated on 09/Aug/21

ψ(x)=−γ+Σ_(n=0) ^∞ ((1/(n+1))−(1/(n+x))) = ((Γ′(x))/(Γ(x))) ψ(x+1)=(1/x)+ψ(x) , ψ((1/2))=−γ−2ln2 ψ((r/m))=−γ−ln(2m)−(π/2)cot(((rπ)/m))+2Σ_(n=1) ^(∣((m−1)/2)∣) cos(((2πnr)/m))ln sin(((πn)/m)) ψ(z+1)=−γ+Σ_(n=2) ^∞ ζ(n)z^(n−1) ψ(x)=−γ+∫_0 ^1 ((1−t^(x−1) )/(1−t))dt ψ′(x)=−∫_0 ^1 ((t^(x−1) ln(t))/(1−t))dt ψ(x)−ψ(1−x)=−πcot(πx) ψ^((1)) (x)+ψ^((1)) (1−x)=π^2 (1+cot^2 (πx)) ψ^((2)) (x)−ψ^((2)) (1−x)=−2π^3 (1+cot^2 (πx))cot(πx)

$$\psi\left(\mathrm{x}\right)=−\gamma+\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{\mathrm{n}+\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{n}+\mathrm{x}}\right)\:=\:\frac{\Gamma'\left(\mathrm{x}\right)}{\Gamma\left(\mathrm{x}\right)} \\ $$$$\psi\left(\mathrm{x}+\mathrm{1}\right)=\frac{\mathrm{1}}{\mathrm{x}}+\psi\left(\mathrm{x}\right)\:,\:\psi\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=−\gamma−\mathrm{2ln2} \\ $$$$\psi\left(\frac{\mathrm{r}}{\mathrm{m}}\right)=−\gamma−\mathrm{ln}\left(\mathrm{2m}\right)−\frac{\pi}{\mathrm{2}}\mathrm{cot}\left(\frac{\mathrm{r}\pi}{\mathrm{m}}\right)+\mathrm{2}\underset{\mathrm{n}=\mathrm{1}} {\overset{\mid\frac{\mathrm{m}−\mathrm{1}}{\mathrm{2}}\mid} {\sum}}\mathrm{cos}\left(\frac{\mathrm{2}\pi\mathrm{nr}}{\mathrm{m}}\right)\mathrm{ln}\:\mathrm{sin}\left(\frac{\pi\mathrm{n}}{\mathrm{m}}\right) \\ $$$$\psi\left({z}+\mathrm{1}\right)=−\gamma+\underset{{n}=\mathrm{2}} {\overset{\infty} {\sum}}\zeta\left({n}\right){z}^{{n}−\mathrm{1}} \\ $$$$\psi\left(\mathrm{x}\right)=−\gamma+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−\mathrm{t}^{\mathrm{x}−\mathrm{1}} }{\mathrm{1}−\mathrm{t}}\mathrm{dt} \\ $$$$\psi'\left(\mathrm{x}\right)=−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{t}^{\mathrm{x}−\mathrm{1}} \mathrm{ln}\left(\mathrm{t}\right)}{\mathrm{1}−\mathrm{t}}\mathrm{dt} \\ $$$$\psi\left(\mathrm{x}\right)−\psi\left(\mathrm{1}−\mathrm{x}\right)=−\pi\mathrm{cot}\left(\pi\mathrm{x}\right) \\ $$$$\psi^{\left(\mathrm{1}\right)} \left(\mathrm{x}\right)+\psi^{\left(\mathrm{1}\right)} \left(\mathrm{1}−\mathrm{x}\right)=\pi^{\mathrm{2}} \left(\mathrm{1}+\mathrm{cot}^{\mathrm{2}} \left(\pi\mathrm{x}\right)\right) \\ $$$$\psi^{\left(\mathrm{2}\right)} \left(\mathrm{x}\right)−\psi^{\left(\mathrm{2}\right)} \left(\mathrm{1}−\mathrm{x}\right)=−\mathrm{2}\pi^{\mathrm{3}} \left(\mathrm{1}+\mathrm{cot}^{\mathrm{2}} \left(\pi\mathrm{x}\right)\right)\mathrm{cot}\left(\pi\mathrm{x}\right) \\ $$

Commented by mathdanisur last updated on 09/Aug/21

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{Ser} \\ $$

Commented by Tawa11 last updated on 09/Aug/21

$$\mathrm{weldone}\:\mathrm{sir} \\ $$

Commented by Tawa11 last updated on 09/Aug/21

sir Ar brandon, please give me the definition of ψ that you used. or books sir. please ...

$$\mathrm{sir}\:\mathrm{Ar}\:\mathrm{brandon},\:\mathrm{please}\:\mathrm{give}\:\mathrm{me}\:\mathrm{the}\:\mathrm{definition}\:\mathrm{of}\:\:\:\psi\:\:\mathrm{that}\:\mathrm{you}\:\mathrm{used}. \\ $$$$\mathrm{or}\:\mathrm{books}\:\mathrm{sir}.\:\mathrm{please}\:… \\ $$

Commented by mnjuly1970 last updated on 09/Aug/21