Question Number 126952 by Study last updated on 25/Dec/20
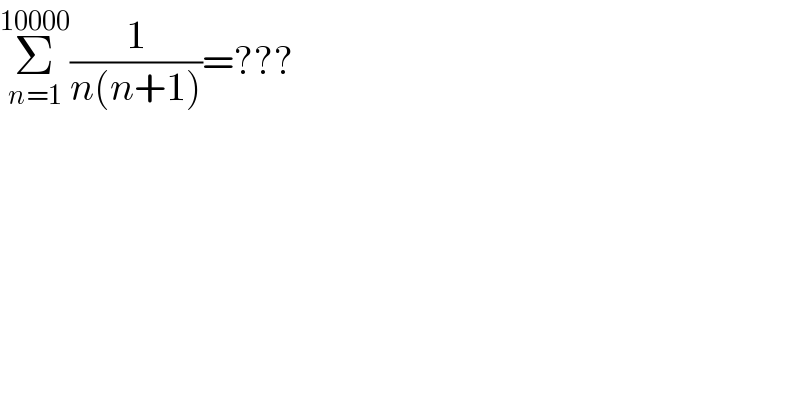
$$\underset{{n}=\mathrm{1}} {\overset{\mathrm{10000}} {\sum}}\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)}=??? \\ $$
Commented by Study last updated on 25/Dec/20

$${help}\:{me} \\ $$
Answered by Dwaipayan Shikari last updated on 25/Dec/20
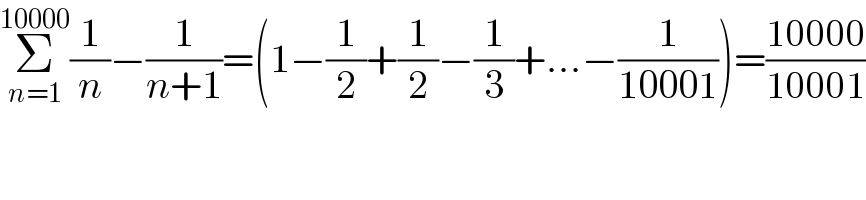
$$\underset{{n}=\mathrm{1}} {\overset{\mathrm{10000}} {\sum}}\frac{\mathrm{1}}{{n}}−\frac{\mathrm{1}}{{n}+\mathrm{1}}=\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{3}}+…−\frac{\mathrm{1}}{\mathrm{10001}}\right)=\frac{\mathrm{10000}}{\mathrm{10001}} \\ $$
Answered by Olaf last updated on 25/Dec/20
![S = Σ_(n=1) ^(10000) (1/(n(n+1))) S = Σ_(n=1) ^(10000) [(1/n)−(1/(n+1))] S = (1/1)−(1/(10001)) = ((10000)/(10001))](https://www.tinkutara.com/question/Q126956.png)
$$\mathrm{S}\:=\:\underset{{n}=\mathrm{1}} {\overset{\mathrm{10000}} {\sum}}\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)} \\ $$$$\mathrm{S}\:=\:\underset{{n}=\mathrm{1}} {\overset{\mathrm{10000}} {\sum}}\left[\frac{\mathrm{1}}{{n}}−\frac{\mathrm{1}}{{n}+\mathrm{1}}\right] \\ $$$$\mathrm{S}\:=\:\frac{\mathrm{1}}{\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{10001}}\:=\:\frac{\mathrm{10000}}{\mathrm{10001}} \\ $$
Answered by bramlexs22 last updated on 25/Dec/20
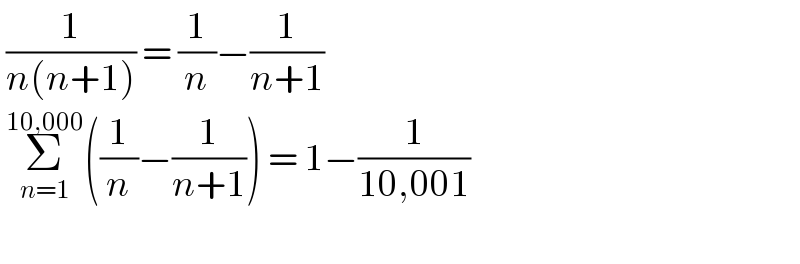
$$\:\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)}\:=\:\frac{\mathrm{1}}{{n}}−\frac{\mathrm{1}}{{n}+\mathrm{1}} \\ $$$$\:\underset{{n}=\mathrm{1}} {\overset{\mathrm{10},\mathrm{000}} {\sum}}\left(\frac{\mathrm{1}}{{n}}−\frac{\mathrm{1}}{{n}+\mathrm{1}}\right)\:=\:\mathrm{1}−\frac{\mathrm{1}}{\mathrm{10},\mathrm{001}} \\ $$$$ \\ $$
