n-1-2n-1-2n-1-4n-2-

Question Number 103881 by bemath last updated on 18/Jul/20

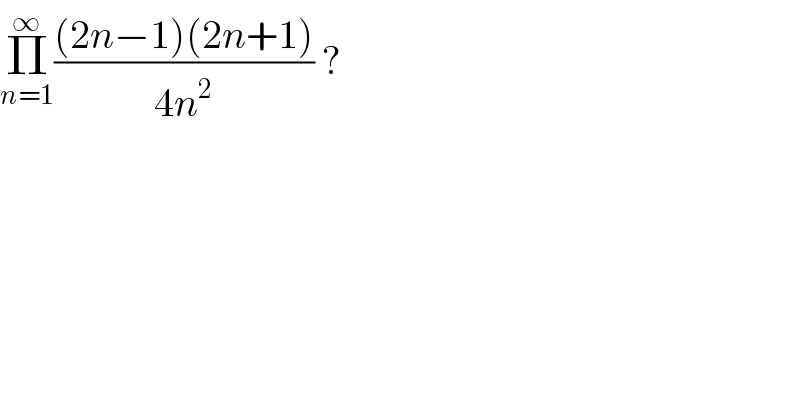
$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\frac{\left(\mathrm{2}{n}−\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{4}{n}^{\mathrm{2}} }\:? \\ $$

Answered by bramlex last updated on 18/Jul/20

use Euler infinity product ((sin x)/x) = Π_(n=1) ^∞ (1−(x^2 /(n^2 π^2 ))) put x = (π/2) ⇒((sin ((π/2)))/(π/2)) = Π_(n=1) ^∞ (1−((π^2 /4)/(n^2 π^2 ))) (2/π) = Π_(n=1) ^∞ (1−(1/(4n^2 ))) (2/π) = Π_(n=1) ^∞ (((4n^2 −1)/(4n^2 )))= Π_(n=1) ^∞ (((2n−1)(2n+1))/(4n^2 )).

$${use}\:{Euler}\:{infinity}\:{product}\: \\ $$$$\frac{\mathrm{sin}\:{x}}{{x}}\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{{n}^{\mathrm{2}} \pi^{\mathrm{2}} }\right) \\ $$$${put}\:{x}\:=\:\frac{\pi}{\mathrm{2}}\:\Rightarrow\frac{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{2}}\right)}{\frac{\pi}{\mathrm{2}}}\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\left(\mathrm{1}−\frac{\frac{\pi^{\mathrm{2}} }{\mathrm{4}}}{{n}^{\mathrm{2}} \pi^{\mathrm{2}} }\right) \\ $$$$\frac{\mathrm{2}}{\pi}\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4}{n}^{\mathrm{2}} }\right)\: \\ $$$$\frac{\mathrm{2}}{\pi}\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\left(\frac{\mathrm{4}{n}^{\mathrm{2}} −\mathrm{1}}{\mathrm{4}{n}^{\mathrm{2}} }\right)=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\frac{\left(\mathrm{2}{n}−\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{4}{n}^{\mathrm{2}} }. \\ $$

Commented by bemath last updated on 18/Jul/20

$${cooll} \\ $$

Answered by mathmax by abdo last updated on 18/Jul/20

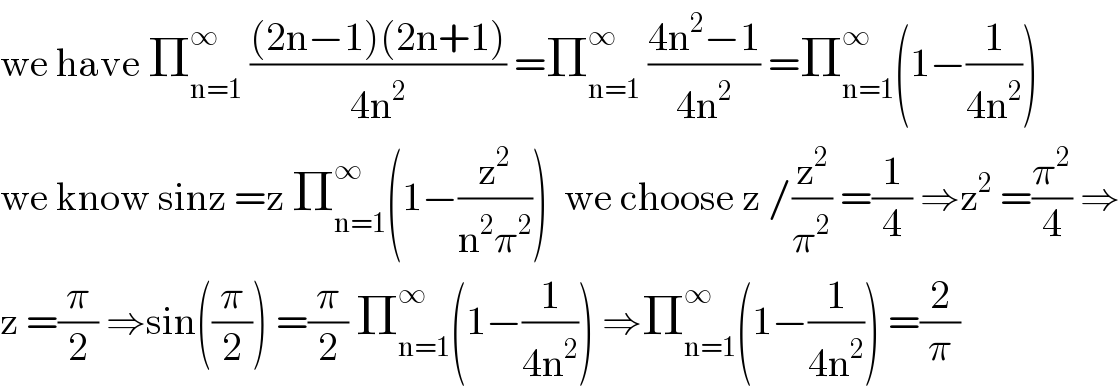
we have Π_(n=1) ^∞ (((2n−1)(2n+1))/(4n^2 )) =Π_(n=1) ^∞ ((4n^2 −1)/(4n^2 )) =Π_(n=1) ^∞ (1−(1/(4n^2 ))) we know sinz =z Π_(n=1) ^∞ (1−(z^2 /(n^2 π^2 ))) we choose z /(z^2 /π^2 ) =(1/4) ⇒z^2 =(π^2 /4) ⇒ z =(π/2) ⇒sin((π/2)) =(π/2) Π_(n=1) ^∞ (1−(1/(4n^2 ))) ⇒Π_(n=1) ^∞ (1−(1/(4n^2 ))) =(2/π)

$$\mathrm{we}\:\mathrm{have}\:\prod_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\left(\mathrm{2n}−\mathrm{1}\right)\left(\mathrm{2n}+\mathrm{1}\right)}{\mathrm{4n}^{\mathrm{2}} }\:=\prod_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{4n}^{\mathrm{2}} −\mathrm{1}}{\mathrm{4n}^{\mathrm{2}} }\:=\prod_{\mathrm{n}=\mathrm{1}} ^{\infty} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4n}^{\mathrm{2}} }\right) \\ $$$$\mathrm{we}\:\mathrm{know}\:\mathrm{sinz}\:=\mathrm{z}\:\prod_{\mathrm{n}=\mathrm{1}} ^{\infty} \left(\mathrm{1}−\frac{\mathrm{z}^{\mathrm{2}} }{\mathrm{n}^{\mathrm{2}} \pi^{\mathrm{2}} }\right)\:\:\mathrm{we}\:\mathrm{choose}\:\mathrm{z}\:/\frac{\mathrm{z}^{\mathrm{2}} }{\pi^{\mathrm{2}} }\:=\frac{\mathrm{1}}{\mathrm{4}}\:\Rightarrow\mathrm{z}^{\mathrm{2}} \:=\frac{\pi^{\mathrm{2}} }{\mathrm{4}}\:\Rightarrow \\ $$$$\mathrm{z}\:=\frac{\pi}{\mathrm{2}}\:\Rightarrow\mathrm{sin}\left(\frac{\pi}{\mathrm{2}}\right)\:=\frac{\pi}{\mathrm{2}}\:\prod_{\mathrm{n}=\mathrm{1}} ^{\infty} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4n}^{\mathrm{2}} }\right)\:\Rightarrow\prod_{\mathrm{n}=\mathrm{1}} ^{\infty} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4n}^{\mathrm{2}} }\right)\:=\frac{\mathrm{2}}{\pi} \\ $$

Commented by bemath last updated on 18/Jul/20

$${thank}\:{you}\:{sir} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply