Question Number 167517 by Gbenga last updated on 18/Mar/22

$$\underset{\boldsymbol{\mathrm{n}}=\mathrm{1}} {\overset{\infty} {\boldsymbol{\sum}}}\frac{\boldsymbol{\mathrm{cos}}\left(\boldsymbol{\mathrm{n}}\right)\boldsymbol{\mathrm{sin}}\left(\boldsymbol{\mathrm{n}}\right)}{\boldsymbol{\mathrm{tan}}\left(\boldsymbol{\mathrm{n}}\right)} \\ $$
Answered by alephzero last updated on 18/Mar/22
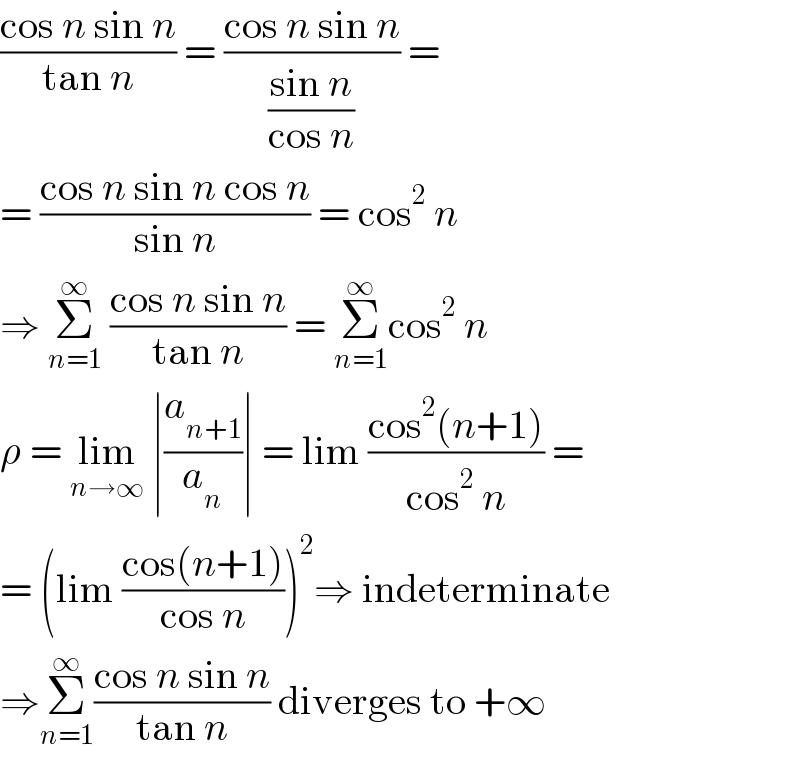
$$\frac{\mathrm{cos}\:{n}\:\mathrm{sin}\:{n}}{\mathrm{tan}\:{n}}\:=\:\frac{\mathrm{cos}\:{n}\:\mathrm{sin}\:{n}}{\frac{\mathrm{sin}\:{n}}{\mathrm{cos}\:{n}}}\:= \\ $$$$=\:\frac{\mathrm{cos}\:{n}\:\mathrm{sin}\:{n}\:\mathrm{cos}\:{n}}{\mathrm{sin}\:{n}}\:=\:\mathrm{cos}^{\mathrm{2}} \:{n} \\ $$$$\Rightarrow\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\mathrm{cos}\:{n}\:\mathrm{sin}\:{n}}{\mathrm{tan}\:{n}}\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\mathrm{cos}^{\mathrm{2}} \:{n} \\ $$$$\rho\:=\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:\mid\frac{{a}_{{n}+\mathrm{1}} }{{a}_{{n}} }\mid\:=\:\mathrm{lim}\:\frac{\mathrm{cos}^{\mathrm{2}} \left({n}+\mathrm{1}\right)}{\mathrm{cos}^{\mathrm{2}} \:{n}}\:= \\ $$$$=\:\left(\mathrm{lim}\:\frac{\mathrm{cos}\left({n}+\mathrm{1}\right)}{\mathrm{cos}\:{n}}\right)^{\mathrm{2}} \Rightarrow\:\mathrm{indeterminate} \\ $$$$\Rightarrow\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\:{n}\:\mathrm{sin}\:{n}}{\mathrm{tan}\:{n}}\:\mathrm{diverges}\:\mathrm{to}\:+\infty \\ $$
Commented by Gbenga last updated on 18/Mar/22

$$\boldsymbol{\mathrm{thanks}}\:\boldsymbol{\mathrm{sir}} \\ $$
