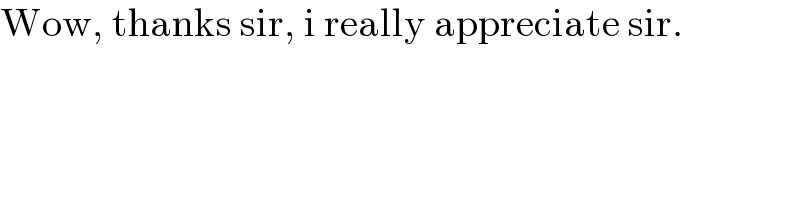n-1-n-1-3-3-

Question Number 125918 by I want to learn more last updated on 15/Dec/20

\underset{n = - \infty}{\sum^{\infty}} \frac{1}{{(n + \frac{1}{3})}^{3}}

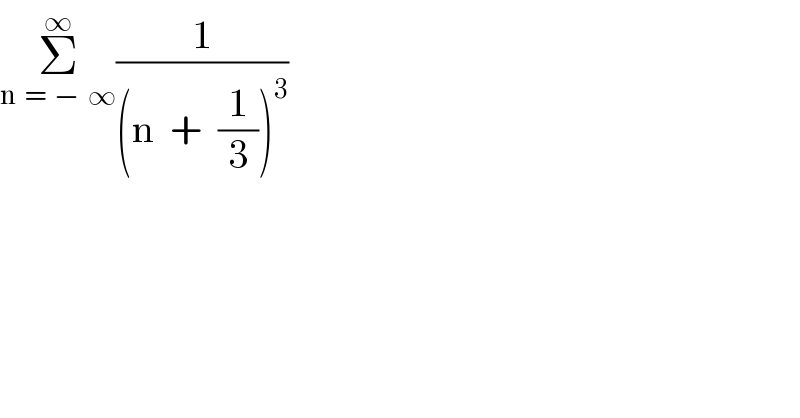
Answered by mindispower last updated on 15/Dec/20

f (z) = \frac{π c o t (π z)}{{(z + \frac{1}{3})}^{3}}, o v e r {R e}^{i θ}, θ \in [0, 2 π]; R \to \infty

\lim_{R \to \infty} \int_{C} f (z) d z = 2 i π R e s (f, C) = 0

∣ c o t (π z) ∣< M, ∣ z ∣\to 0

\frac{1}{{(z + \frac{1}{3})}^{3}} \to 0 w h e n ∣ z ∣\to \infty

p o l s o f f a r e - \frac{1}{3}, o r d e r 3

k \in Z o r d e r 1

R e s (f, k) = \frac{1}{{(k + \frac{1}{3})}^{3}}

R e s (f, - \frac{1}{3}) = \frac{1}{2} \frac{\partial}{\partial z^{2}} . π c o t (π z)

= \frac{1}{2} [2 π^{3} (1 + {c o t}^{2} (π z)) c o t (π z)) ∣ z = - \frac{1}{3}

= π^{3} (\frac{4}{3}) (- \frac{1}{\sqrt{3}})

= \frac{- 4 π^{3}}{3 \sqrt{3}}

\underset{k \in Z}{\sum^{}} R e s (f, k) + R e s (f, - \frac{1}{3}) = 0

\Rightarrow Σ r e s (f, k) = - R e s (f, - \frac{1}{3}) = \frac{4 π^{3}}{3 \sqrt{3}}

Commented by I want to learn more last updated on 15/Dec/20

Thanks sir . I appreciate .

Answered by mnjuly1970 last updated on 16/Dec/20

Commented by I want to learn more last updated on 16/Dec/20

Thanks sir, i appreciate . But sir, where can i learn summation like this .

please . Tell me a book to download . please .

Commented by mnjuly1970 last updated on 16/Dec/20

t h a n k y o u s o m u c h m a s t e r . .

1 . a d v a n c e d c a l c u l u s \dots {_{2 . R u e l - c h u c h i l l}^{1 . M u r r a y - s p i e g e l}

2 . c o m p l e x a n a l y s i s . . (R u e l - c h u r c h i l l)

Commented by I want to learn more last updated on 20/Dec/20

Wow, thanks sir, i really appreciate sir .