Menu Close

nice-calculus-evaluate-0-x-tan-1-x-1-x-2-dx-

Tinku Tara 0 Comments
Pin




Question Number 125390 by mnjuly1970 last updated on 10/Dec/20
nice calculus... evaluate ::::↷ Ω=∫_0 ^( ∞) (((√x) tan^(−1) (x))/(1+x^2 ))dx=???
$$\:\:\:\:\:\:\:\:{nice}\:\:{calculus}… \\ $$$$\:\:\:\:\:{evaluate}\:::::\curvearrowright \\ $$$$\:\:\:\:\Omega=\int_{\mathrm{0}} ^{\:\infty} \frac{\sqrt{{x}}\:{tan}^{−\mathrm{1}} \left({x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=??? \\ $$
Answered by mathmax by abdo last updated on 11/Dec/20
I =∫_0 ^∞ (((√x)arctan(x))/(1+x^2 ))dx changement (√x)=t give x=t^2 ⇒ I=∫_0 ^∞ ((t arctan(t^2 ))/(1+t^4 ))(2t)dt =2 ∫_0 ^∞ (t^2 /(1+t^4 ))arctan(t^2 )dt =∫_(−∞) ^(+∞) ((t^2 arctan(t^2 ))/(t^4 +1))dt its clear that I is convergent let ϕ(z)=((z^2 arctan(z^2 ))/(z^4 +1)) we have ϕ(z)=((z^2 arctan(z^2 ))/((z^2 −i)(z^2 +i))) =((z^2 arctan(z^2 ))/((z−e^((iπ)/4) )(z+e^((iπ)/4) )(z−e^(−((iπ)/4)) )(z+e^(−((iπ)/4)) ))) ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ{Res(ϕ,e^((iπ)/4) )+Res(ϕ,−e^(−((iπ)/4)) )} Res(ϕ,e^((iπ)/4) ) =((iarctan(i))/(2e^((iπ)/4) (2i))) =(1/4)e^(−((iπ)/4)) arctan(i) Res(ϕ,−e^(−((iπ)/4)) ) =((−iarctan(−i))/((−2i)(−2e^(−((iπ)/4)) ))) =(1/4)e^((iπ)/4) arctan(i) ⇒ ∫_(−∞) ^(+∞) ϕ(z)dz =((2iπ)/4) arctan(i){e^((iπ)/4) +e^(−((iπ)/4)) } =((iπ)/2)arctan(i)×2cos((π/4)) =iπarctan(i)×((√2)/2) ⇒I=((iπ)/( (√2))) arctan(i) rest to determine the value of arctan(i)... be continued...
$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\sqrt{\mathrm{x}}\mathrm{arctan}\left(\mathrm{x}\right)}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:\:\mathrm{changement}\:\sqrt{\mathrm{x}}=\mathrm{t}\:\mathrm{give}\:\mathrm{x}=\mathrm{t}^{\mathrm{2}} \:\Rightarrow \\ $$$$\mathrm{I}=\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{t}\:\mathrm{arctan}\left(\mathrm{t}^{\mathrm{2}} \right)}{\mathrm{1}+\mathrm{t}^{\mathrm{4}} }\left(\mathrm{2t}\right)\mathrm{dt}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{4}} }\mathrm{arctan}\left(\mathrm{t}^{\mathrm{2}} \right)\mathrm{dt} \\ $$$$=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{t}^{\mathrm{2}} \:\mathrm{arctan}\left(\mathrm{t}^{\mathrm{2}} \right)}{\mathrm{t}^{\mathrm{4}} \:+\mathrm{1}}\mathrm{dt}\:\:\mathrm{its}\:\mathrm{clear}\:\mathrm{that}\:\mathrm{I}\:\mathrm{is}\:\mathrm{convergent}\:\mathrm{let} \\ $$$$\varphi\left(\mathrm{z}\right)=\frac{\mathrm{z}^{\mathrm{2}} \:\mathrm{arctan}\left(\mathrm{z}^{\mathrm{2}} \right)}{\mathrm{z}^{\mathrm{4}} \:+\mathrm{1}}\:\:\mathrm{we}\:\mathrm{have}\:\varphi\left(\mathrm{z}\right)=\frac{\mathrm{z}^{\mathrm{2}} \mathrm{arctan}\left(\mathrm{z}^{\mathrm{2}} \right)}{\left(\mathrm{z}^{\mathrm{2}} −\mathrm{i}\right)\left(\mathrm{z}^{\mathrm{2}} \:+\mathrm{i}\right)} \\ $$$$=\frac{\mathrm{z}^{\mathrm{2}} \:\mathrm{arctan}\left(\mathrm{z}^{\mathrm{2}} \right)}{\left(\mathrm{z}−\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(\mathrm{z}+\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(\mathrm{z}+\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)} \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\left\{\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)+\mathrm{Res}\left(\varphi,−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\right\} \\ $$$$\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\:=\frac{\mathrm{iarctan}\left(\mathrm{i}\right)}{\mathrm{2e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \left(\mathrm{2i}\right)}\:=\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \:\mathrm{arctan}\left(\mathrm{i}\right) \\ $$$$\mathrm{Res}\left(\varphi,−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\:=\frac{−\mathrm{iarctan}\left(−\mathrm{i}\right)}{\left(−\mathrm{2i}\right)\left(−\mathrm{2e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)}\:=\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \:\mathrm{arctan}\left(\mathrm{i}\right)\:\Rightarrow \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\frac{\mathrm{2i}\pi}{\mathrm{4}}\:\mathrm{arctan}\left(\mathrm{i}\right)\left\{\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \:+\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right\} \\ $$$$=\frac{\mathrm{i}\pi}{\mathrm{2}}\mathrm{arctan}\left(\mathrm{i}\right)×\mathrm{2cos}\left(\frac{\pi}{\mathrm{4}}\right)\:=\mathrm{i}\pi\mathrm{arctan}\left(\mathrm{i}\right)×\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{I}=\frac{\mathrm{i}\pi}{\:\sqrt{\mathrm{2}}}\:\mathrm{arctan}\left(\mathrm{i}\right)\:\:\mathrm{rest}\:\mathrm{to}\:\mathrm{determine}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{arctan}\left(\mathrm{i}\right)… \\ $$$$\mathrm{be}\:\mathrm{continued}… \\ $$
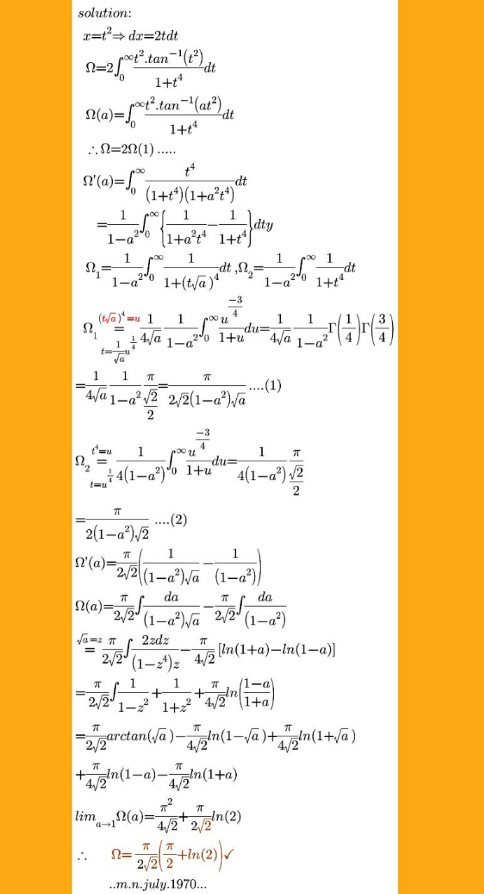
Answered by mnjuly1970 last updated on 11/Dec/20

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *