nice-calculus-find-0-4-ln-x-4x-x-2-1-2-dx-

Question Number 124432 by mnjuly1970 last updated on 03/Dec/20

\dots n i c e c a l c u l u s \dots

f i n d ::

ϕ = \int_{0}^{4} \frac{l n (x)}{{(4 x - x^{2})}^{\frac{1}{2}}} d x = ?

Answered by mindispower last updated on 03/Dec/20

x = 4 t

q = \int_{0}^{1} \frac{l n (4) + l n (t)}{{(t - t^{2})}^{\frac{1}{2}}} d t

w = l n (4) \int_{0}^{1} \frac{1}{t^{\frac{1}{2}} {(1 - t)}^{\frac{1}{2}}} + \int_{0}^{1} \frac{l n (t)}{\sqrt{t} . \sqrt{1 - t}} d t

= l n (4) . \int_{0}^{1} t^{\frac{1}{2} -} {(1 - t)}^{\frac{1}{2} - 1} d t + \partial_{c} \int_{0}^{1} t^{\frac{1}{2} + c - 1} {(1 - t)}^{\frac{1}{2} - 1} d t ∣_{c = 0}

= l n (4) β (\frac{1}{2}, \frac{1}{2}) + \partial_{C} β (\frac{1}{2} + c, \frac{1}{2}) ∣_{c = 0}

= \frac{l n (4) π}{s i n (\frac{π}{2})} + β (\frac{1}{2} + c, \frac{1}{2}) (Ψ (\frac{1}{2} + c) - Ψ (1 + c)) ∣_{c = 0}

= π l n (4) + π (Ψ (\frac{1}{2}) - Ψ (1))

= π (l n (4) + π (- 2 l n (2) - γ + γ)

= 0

Commented by mnjuly1970 last updated on 03/Dec/20

m e r c e y m r m i n d s p o w e r . .

t h a n k s a l o t \dots

Commented by mindispower last updated on 05/Dec/20

w i t h e p l e a s u r s i r

Answered by mnjuly1970 last updated on 03/Dec/20

a n o t h e r w a y

w e k n o w :

\int_{0}^{\frac{π}{2}} l n (s i n (x)) d x = \frac{- π}{2} l n (2) ✓

x = 4 y

ϕ = \int_{0}^{1} \frac{l n (4) + l n (y)}{\sqrt{y} \sqrt{1 - y}} d y

\overset{y = {s i n}^{2} (t)}{=} \int_{0}^{\frac{π}{2}} {\frac{2 l n (2) + 2 l n (s i n (t)}{s i n (t) c o s (t)}} (2 s i n (t) c o s (t)) d t

= 2 π l n (2) + 4 \int_{0}^{\frac{π}{2}} l n (s i n (t)) d t

= 2 π l n (2) - 2 π l n (2) = 0 ✓

Answered by mathmax by abdo last updated on 03/Dec/20

A = \int_{0}^{4} \frac{lnx}{\sqrt{4 x - x^{2}}} dx \Rightarrow A = \int_{0}^{4} \frac{lnx}{\sqrt{- (x^{2} - 4 x + 4 - 4)}} dx

= \int_{0}^{4} \frac{lnx}{\sqrt{4 - {(x - 2)}^{2}}} dx =_{x - 2 = 2 cost} \int_{π}^{0} \frac{\ln (2 + 2 cost)}{2 sint} (- 2 sint) dt

= \int_{0}^{π} \ln (2 (1 + cost)) dt = \int_{0}^{π} \ln ({4 \cos}^{2} (\frac{t}{2})) dt

= 2 π \ln (2) + 2 \int_{0}^{π} \ln (\cos (\frac{t}{2})) dt (\to \frac{t}{2} = x)

= 2 π \ln (2) + 2 \int_{0}^{\frac{π}{2}} \ln (cosx) (2 dx) = 2 π \ln (2) + 4 (- \frac{π}{2} \ln 2)

= 2 π \ln 2 - 2 π \ln (2) = 0 \Rightarrow A = 0

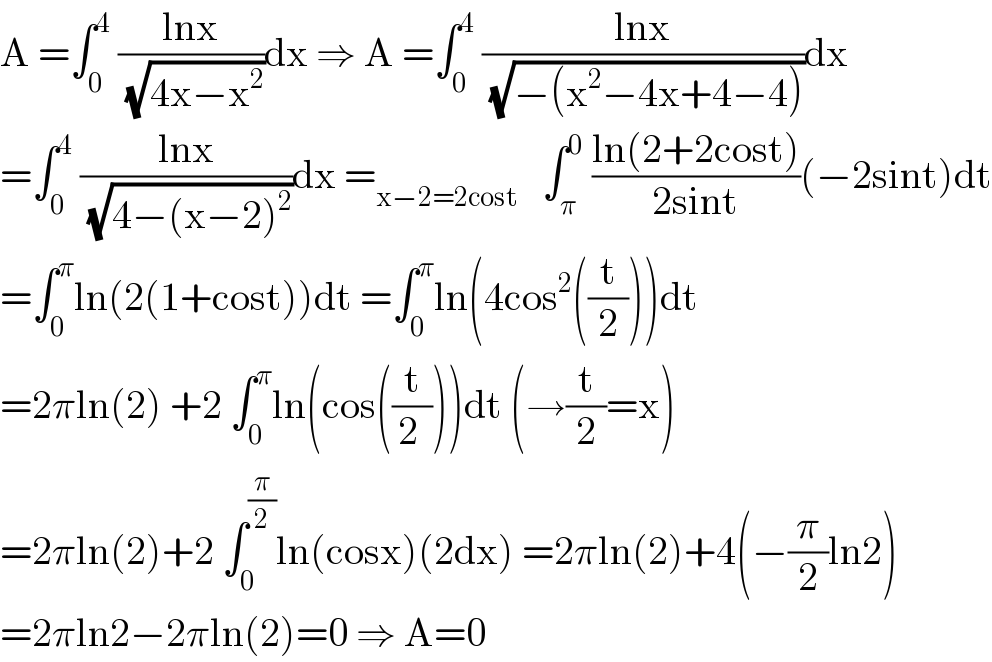
Commented by mnjuly1970 last updated on 03/Dec/20

t h a n k s a l o t s i r m a x

e x c e l l e n t