nice-calculus-if-cos-1-x-cos-1-y-cos-1-z-pi-show-that-x-2-y-2-z-2-2xyz-1-M-N-july-1970-

Question Number 120156 by mnjuly1970 last updated on 29/Oct/20

\dots ♠ n i c e c a l c u l u s ♠ \dots

i f {c o s}^{- 1} (x) + {c o s}^{- 1} (y) + {c o s}^{- 1} (z) = π ✓

s h o w t h a t ::

x^{2} + y^{2} + z^{2} + 2 x y z = 1 ✓

\dots ♣ M . N . j u l y . 1970 ♣ \dots

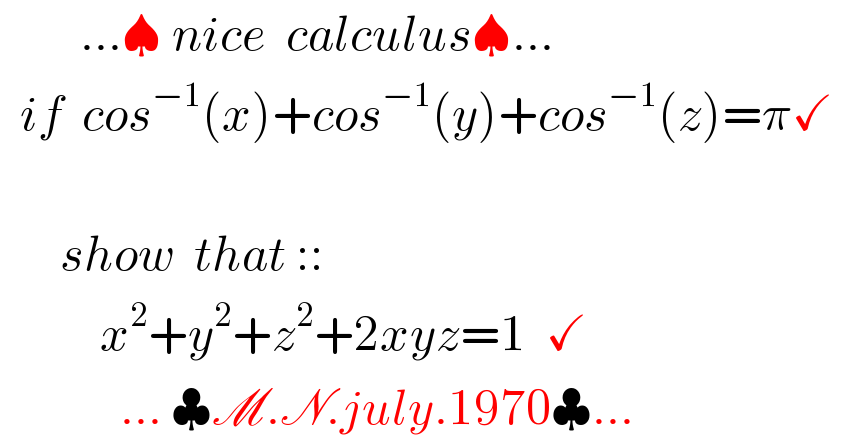
Answered by TANMAY PANACEA last updated on 29/Oct/20

c o s ({c o s}^{- 1} x + {c o s}^{- 1} y) = c o s (π - {c o s}^{- 1} z)

x y - \sqrt{1 - x^{2}} \sqrt{1 - y^{2}} = - z

{(x y + z)}^{2} = (1 - x^{2}) (1 - y^{2})

x^{2} y^{2} + 2 x y z + z^{2} = 1 - x^{2} - y^{2} + x^{2} y^{2}

x^{2} + y^{2} + z^{2} + 2 x y z = 1

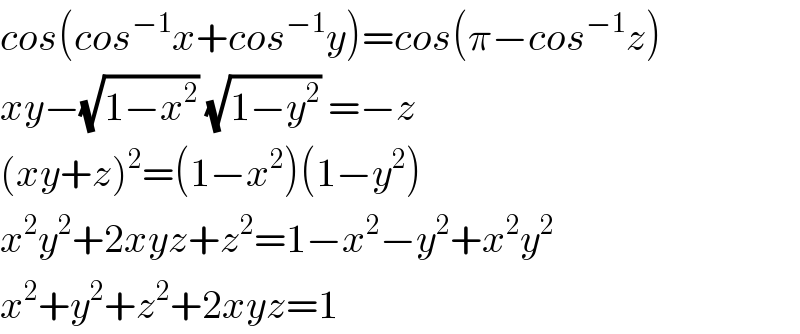
Commented by mnjuly1970 last updated on 29/Oct/20

t h a n k y o u s o m u c h m a s t e r

t a n m a y \dots g r a t e f u l \dots

Commented by TANMAY PANACEA last updated on 29/Oct/20

m o s t w e l c o m e s i r

Commented by Dwaipayan Shikari last updated on 29/Oct/20

��

Commented by Ar Brandon last updated on 30/Oct/20

মহান কাজ��

Commented by Ar Brandon last updated on 30/Oct/20

ওহে, বন্ধু আমার. আশা করি ভালই আছ.��

Commented by som(math1967) last updated on 30/Oct/20

এসব কি?

Commented by Ar Brandon last updated on 30/Oct/20

কোথায় ? আপনি স্যার সম্পর্কে কি কথা বলছেন?��

Commented by som(math1967) last updated on 30/Oct/20

? ?

Commented by Dwaipayan Shikari last updated on 30/Oct/20

বাংলা শিখলে কখন?

Commented by Ar Brandon last updated on 30/Oct/20

কখনই না��