nice-calculus-prove-that-lim-n-n-1-n-2-n-e-3-4-where-n-superfactorial-n-n-1-n-2-3-2-1-m-

Question Number 120312 by mnjuly1970 last updated on 30/Oct/20

\dots ♣ n i c e c a l c u l u s ♣ \dots

p r o v e t h a t ::

{l i m}_{n \to \infty} \frac{\sqrt[n^{2}]{n $}}{\sqrt{n}} ? \overset{? ? ?}{=} e^{\frac{- 3}{4}}

w h e r e :: n $ \overset{s u p e r f a c t o r i a l}{=} n! . (n - 1)! . (n - 2)! \dots 3! . 2! . 1!

\dots ♠ m . n . 1970 ♠ \dots

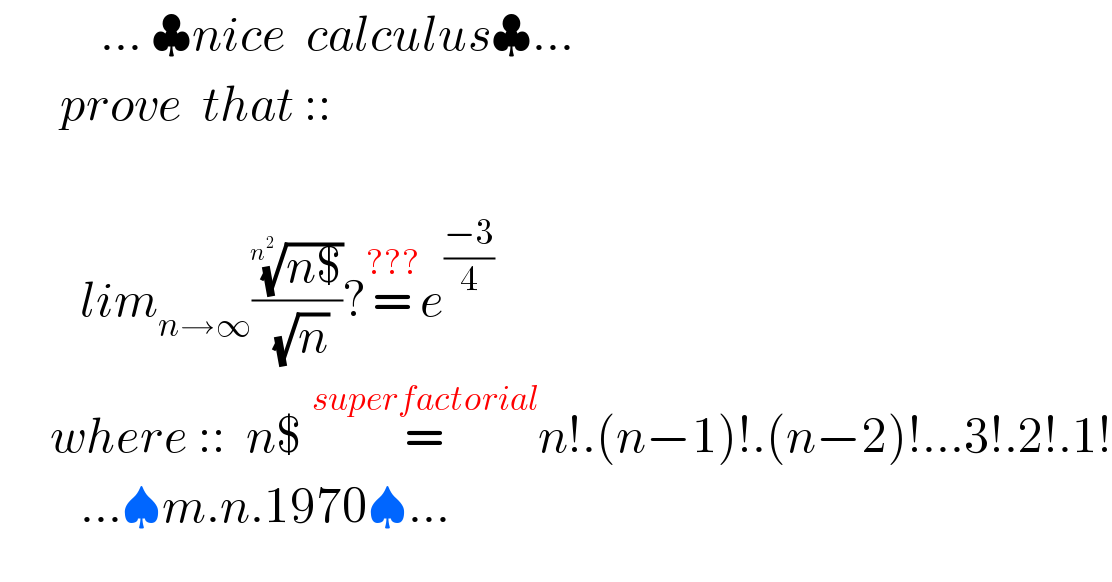
Answered by mathmax by abdo last updated on 30/Oct/20

you are a super man \dots .

Commented by mnjuly1970 last updated on 31/Oct/20

g r a t e f u l s i r m a x

t h a n k y o u f o r y o u r f a v o r

i n f a c t . y o u a r e v e r y v e r y p o w e r f u l a n d e x p e r t i n m a t h e m a t i c s .

i a m y o u r s t u d e n t m r m a x .

Commented by mnjuly1970 last updated on 31/Oct/20

Commented by mathmax by abdo last updated on 01/Nov/20

you are always welcome . . thanks

Commented by mnjuly1970 last updated on 01/Nov/20

s i c e r e l y y o u r s

m . n . j u l y . 1970