nice-calculus-verify-that-A-2-5-1-3-1-5-is-a-rational-number-

Question Number 126109 by mnjuly1970 last updated on 17/Dec/20

\dots . n i c e c a l c u l u s \dots

v e r i f y t h a t :: A = \frac{\sqrt[3]{2 + \sqrt{5}}}{1 + \sqrt{5}} i s

a r a t i o n a l n u m b e r \dots

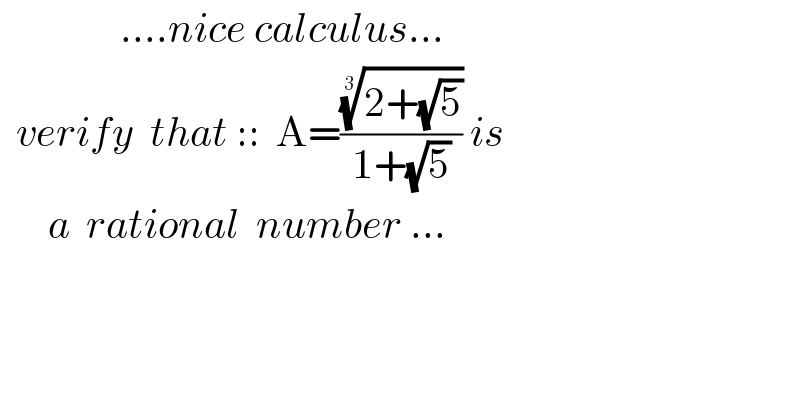
Answered by som(math1967) last updated on 17/Dec/20

\frac{\sqrt[3]{8 (2 + \sqrt{5)}}}{(1 + \sqrt{5}) \times 2}

= \frac{\sqrt[3]{16 + 8 \sqrt{5}}}{2 (1 + \sqrt{5})}

= \frac{\sqrt[3]{1^{3} + 3 \times 1^{2} \times \sqrt{5} + 3 \times 1 \times {(\sqrt{5})}^{2} + {(\sqrt{5})}^{3}}}{2 (1 + \sqrt{5})}

= \frac{\sqrt[3]{{(1 + \sqrt{5})}^{3}}}{2 (1 + \sqrt{5})} = \frac{1}{2} i s a r a t i o n a l

n u m b e r .

Commented by mnjuly1970 last updated on 17/Dec/20

t h a n k s a l o t

Answered by Dwaipayan Shikari last updated on 17/Dec/20

A = \frac{\sqrt[3]{1 + t}}{t} = \sqrt{\frac{1}{t^{3}} + \frac{1}{t^{2}}} = \sqrt[3]{\frac{1}{{(1 + \sqrt{5})}^{3}} + \frac{1}{{(1 + \sqrt{5})}^{2}}}

= \sqrt[3]{{(\frac{\sqrt{5} - 1}{4})}^{2} + {(\frac{\sqrt{5} - 1}{4})}^{3}} = \sqrt[3]{\frac{5 \sqrt{5} - 1 - 3 \sqrt{5} (\sqrt{5} - 1)}{64} + \frac{24 - 8 \sqrt{5}}{64}}

= \sqrt[3]{\frac{5 \sqrt{5} - 1 - 15 + 3 \sqrt{5} + 24 - 8 \sqrt{5}}{64}} = \frac{1}{2}

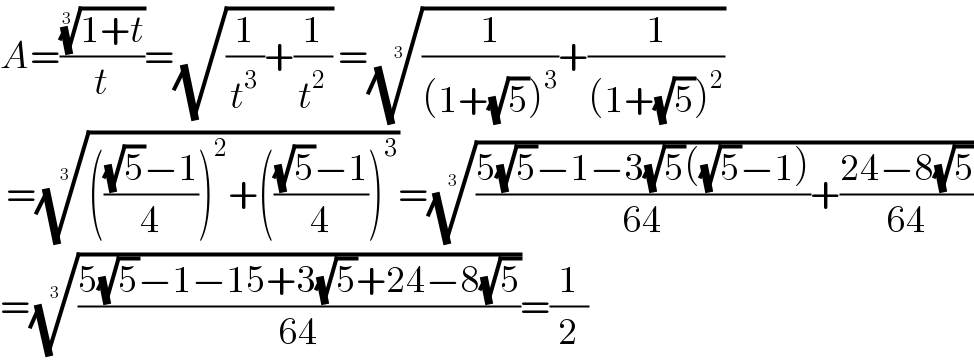
Commented by mnjuly1970 last updated on 17/Dec/20

g r a t e f u l \dots