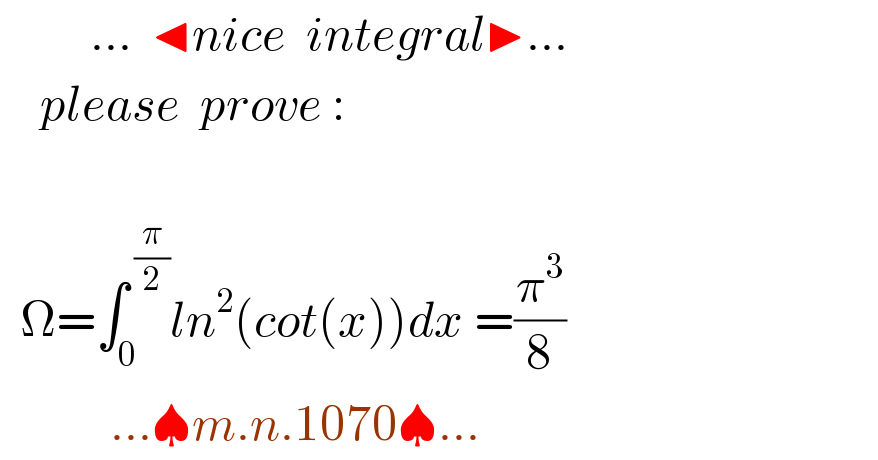
Question Number 117953 by mnjuly1970 last updated on 14/Oct/20
$$\:\:\:\:\:\:\:\:\:…\:\:\blacktriangleleft{nice}\:\:{integral}\blacktriangleright… \\ $$$$\:\:\:\:{please}\:\:{prove}\:: \\ $$$$ \\ $$$$\:\:\Omega=\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} {ln}^{\mathrm{2}} \left({cot}\left({x}\right)\right){dx}\:=\frac{\pi^{\mathrm{3}} }{\mathrm{8}}\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:…\spadesuit{m}.{n}.\mathrm{1070}\spadesuit… \\ $$
Answered by mindispower last updated on 14/Oct/20
![Ω=∫_0 ^(π/2) ln^2 (cot(x))dx;cot(x)=s⇒ Ω=∫_0 ^∞ ((ln^2 (s))/(1+s^2 ))ds=2∫_0 ^1 ((ln^2 (s))/(1+s^2 ))ds=2∫_0 ^1 Σ(−s^2 )^k ln^2 (s)ds =2Σ_(n≥0) (−1)^n ∫_0 ^1 s^(2k) ln^2 (s)ds ∫_0 ^1 s^n ln^2 (s)ds=−(2/(n+1))∫_0 ^1 s^n ln(s)ds =(2/((n+1)^2 )) ∫_0 ^1 s^n ds=(2/((n+1)^3 )) we get Σ_(n≥0) ((4(−1)^n )/((2n+1)^3 ))=Σ_(n≥0) (4/((4n+1)^3 ))−Σ_(n≥0) (4/((4n+3)^3 )) =(1/(16))[Σ_(n≥0) (1/((n+(1/4))^3 ))−Σ_(n≥0) (1/((n+(3/4))^3 ))] =(1/(16))[Ψ^3 ((1/4))−Ψ^3 ((3/4))] Ψ(1−x)−Ψ(x)=πcot(πx) ⇒Ψ^3 (1−x)−Ψ^3 (x)=(d^2 /dx^2 )(πcot(πx)) =(d/dx)(−π^2 (1+cot^2 (πx))=2π^3 (1+cot^2 (πx))cot(πx)) (1/(16))[Ψ^3 ((1/4))−Ψ^3 ((3/4))]=(1/(16))(2π^3 (1+cot^2 ((π/4)))cot((π/4)) =(π^3 /4) may bee forget somthing](https://www.tinkutara.com/question/Q117996.png)
$$\Omega=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}^{\mathrm{2}} \left({cot}\left({x}\right)\right){dx};{cot}\left({x}\right)={s}\Rightarrow \\ $$$$\Omega=\int_{\mathrm{0}} ^{\infty} \frac{{ln}^{\mathrm{2}} \left({s}\right)}{\mathrm{1}+{s}^{\mathrm{2}} }{ds}=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}^{\mathrm{2}} \left({s}\right)}{\mathrm{1}+{s}^{\mathrm{2}} }{ds}=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \Sigma\left(−{s}^{\mathrm{2}} \right)^{{k}} {ln}^{\mathrm{2}} \left({s}\right){ds} \\ $$$$=\mathrm{2}\underset{{n}\geqslant\mathrm{0}} {\sum}\left(−\mathrm{1}\right)^{{n}} \int_{\mathrm{0}} ^{\mathrm{1}} {s}^{\mathrm{2}{k}} {ln}^{\mathrm{2}} \left({s}\right){ds} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {s}^{{n}} {ln}^{\mathrm{2}} \left({s}\right){ds}=−\frac{\mathrm{2}}{{n}+\mathrm{1}}\int_{\mathrm{0}} ^{\mathrm{1}} {s}^{{n}} {ln}\left({s}\right){ds} \\ $$$$=\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }\:\:\int_{\mathrm{0}} ^{\mathrm{1}} {s}^{{n}} {ds}=\frac{\mathrm{2}}{\left({n}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$${we}\:{get}\:\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{4}\left(−\mathrm{1}\right)^{{n}} }{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{3}} }=\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{4}}{\left(\mathrm{4}{n}+\mathrm{1}\right)^{\mathrm{3}} }−\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{4}}{\left(\mathrm{4}{n}+\mathrm{3}\right)^{\mathrm{3}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{16}}\left[\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{1}}{\left({n}+\frac{\mathrm{1}}{\mathrm{4}}\right)^{\mathrm{3}} }−\underset{{n}\geqslant\mathrm{0}} {\sum}\frac{\mathrm{1}}{\left({n}+\frac{\mathrm{3}}{\mathrm{4}}\right)^{\mathrm{3}} }\right] \\ $$$$=\frac{\mathrm{1}}{\mathrm{16}}\left[\Psi^{\mathrm{3}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)−\Psi^{\mathrm{3}} \left(\frac{\mathrm{3}}{\mathrm{4}}\right)\right] \\ $$$$\Psi\left(\mathrm{1}−{x}\right)−\Psi\left({x}\right)=\pi{cot}\left(\pi{x}\right) \\ $$$$\Rightarrow\Psi^{\mathrm{3}} \left(\mathrm{1}−{x}\right)−\Psi^{\mathrm{3}} \left({x}\right)=\frac{{d}^{\mathrm{2}} }{{dx}^{\mathrm{2}} }\left(\pi{cot}\left(\pi{x}\right)\right) \\ $$$$=\frac{{d}}{{dx}}\left(−\pi^{\mathrm{2}} \left(\mathrm{1}+{cot}^{\mathrm{2}} \left(\pi{x}\right)\right)=\mathrm{2}\pi^{\mathrm{3}} \left(\mathrm{1}+{cot}^{\mathrm{2}} \left(\pi{x}\right)\right){cot}\left(\pi{x}\right)\right) \\ $$$$\frac{\mathrm{1}}{\mathrm{16}}\left[\Psi^{\mathrm{3}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)−\Psi^{\mathrm{3}} \left(\frac{\mathrm{3}}{\mathrm{4}}\right)\right]=\frac{\mathrm{1}}{\mathrm{16}}\left(\mathrm{2}\pi^{\mathrm{3}} \left(\mathrm{1}+{cot}^{\mathrm{2}} \left(\frac{\pi}{\mathrm{4}}\right)\right){cot}\left(\frac{\pi}{\mathrm{4}}\right)\right. \\ $$$$=\frac{\pi^{\mathrm{3}} }{\mathrm{4}}\:\:{may}\:{bee}\:{forget}\:{somthing} \\ $$$$ \\ $$
Commented by mnjuly1970 last updated on 14/Oct/20
$${thank}\:{you}\:{master}\: \\ $$$${your}\:{work}\:{is}\:{very}\:{nice}\:{and} \\ $$$${admirable} \\ $$$${final}\:{answer}\:{is}\:{not}\:{very} \\ $$$${important}\:.{your}\:{solution} \\ $$$${is}\:{fabulous}.{grateful}. \\ $$
Commented by mindispower last updated on 19/Oct/20
$${thank}\:{you}\:{withe}\:{plesur} \\ $$$${sir}\:{do}\:{you}\:{have}\:{somme}\:{lectur}\:{about}\:{polygarithms}\: \\ $$$${function}\:{identities} \\ $$$${thank}\:{you}\:{for}\:{you}\:\:{answer} \\ $$
Answered by mathmax by abdo last updated on 14/Oct/20
![A =∫_0 ^(π/2) ln^2 (cotanx)dx ⇒ A =∫_0 ^(π/2) ln^2 ((1/(tanx)))dx =∫_0 ^(π/2) ln^2 (tanx)dx =_(tanx=t) ∫_0 ^∞ ((ln^2 (t))/(1+t^2 ))dt =∫_0 ^1 ((ln^2 t)/(1+t^2 ))dt +∫_1 ^∞ ((ln^2 t)/(1+t^2 ))dt(→t=(1/u)) =∫_0 ^1 ((ln^2 t)/(1+t^2 ))dt +∫_0 ^1 ((ln^2 u)/((1+(1/u^2 ))))(du/u^2 ) =2∫_0 ^1 ((ln^2 x)/(1+x^2 ))dx =2 ∫_0 ^1 ln^2 x(Σ_(n=0) ^∞ (−1)^n x^(2n) )dx =2 Σ_(n=0) ^∞ (−1)^n ∫_0 ^1 x^(2n) ln^2 x dx =2Σ_(n=0) ^∞ (−1)^n A_n by parts A_n =∫_0 ^1 x^(2n) ln^2 xdx =[(x^(2n+1) /(2n+1))ln^2 x]_0 ^1 −∫_0 ^1 (x^(2n+1) /(2n+1))((2lnx)/x)dx =−(2/((2n+1)))∫_0 ^1 x^(2n) lnx dx =−(2/((2n+1))){ [(x^(2n+1) /(2n+1))lnx]_0 ^1 −∫_0 ^1 (x^(2n) /(2n+1))} =(2/((2n+1)^3 )) ⇒ A =4 Σ_(n=0) ^∞ (((−1)^n )/((2n+1)^3 )) rest to find the value of this serie by sir fourier...be continued....](https://www.tinkutara.com/question/Q118046.png)
$$\mathrm{A}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{ln}^{\mathrm{2}} \left(\mathrm{cotanx}\right)\mathrm{dx}\:\Rightarrow\:\mathrm{A}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{ln}^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{tanx}}\right)\mathrm{dx} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{ln}^{\mathrm{2}} \left(\mathrm{tanx}\right)\mathrm{dx}\:=_{\mathrm{tanx}=\mathrm{t}} \:\:\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{ln}^{\mathrm{2}} \left(\mathrm{t}\right)}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{ln}^{\mathrm{2}} \mathrm{t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt}\:+\int_{\mathrm{1}} ^{\infty} \:\frac{\mathrm{ln}^{\mathrm{2}} \mathrm{t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt}\left(\rightarrow\mathrm{t}=\frac{\mathrm{1}}{\mathrm{u}}\right) \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{ln}^{\mathrm{2}} \mathrm{t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt}\:+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}^{\mathrm{2}} \mathrm{u}}{\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{u}^{\mathrm{2}} }\right)}\frac{\mathrm{du}}{\mathrm{u}^{\mathrm{2}} }\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{ln}^{\mathrm{2}} \mathrm{x}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}^{\mathrm{2}} \mathrm{x}\left(\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{x}^{\mathrm{2n}} \right)\mathrm{dx} \\ $$$$=\mathrm{2}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{\mathrm{n}} \:\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{2n}} \:\mathrm{ln}^{\mathrm{2}} \mathrm{x}\:\mathrm{dx}\:=\mathrm{2}\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{A}_{\mathrm{n}} \:\:\mathrm{by}\:\mathrm{parts} \\ $$$$\mathrm{A}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{2n}} \:\mathrm{ln}^{\mathrm{2}} \mathrm{xdx}\:=\left[\frac{\mathrm{x}^{\mathrm{2n}+\mathrm{1}} }{\mathrm{2n}+\mathrm{1}}\mathrm{ln}^{\mathrm{2}} \mathrm{x}\right]_{\mathrm{0}} ^{\mathrm{1}} −\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{x}^{\mathrm{2n}+\mathrm{1}} }{\mathrm{2n}+\mathrm{1}}\frac{\mathrm{2lnx}}{\mathrm{x}}\mathrm{dx} \\ $$$$=−\frac{\mathrm{2}}{\left(\mathrm{2n}+\mathrm{1}\right)}\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{2n}} \mathrm{lnx}\:\mathrm{dx}\:=−\frac{\mathrm{2}}{\left(\mathrm{2n}+\mathrm{1}\right)}\left\{\:\left[\frac{\mathrm{x}^{\mathrm{2n}+\mathrm{1}} }{\mathrm{2n}+\mathrm{1}}\mathrm{lnx}\right]_{\mathrm{0}} ^{\mathrm{1}} −\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{x}^{\mathrm{2n}} }{\mathrm{2n}+\mathrm{1}}\right\} \\ $$$$=\frac{\mathrm{2}}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{3}} }\:\Rightarrow\:\mathrm{A}\:=\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{3}} }\:\mathrm{rest}\:\mathrm{to}\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of} \\ $$$$\mathrm{this}\:\mathrm{serie}\:\mathrm{by}\:\mathrm{sir}\:\mathrm{fourier}…\mathrm{be}\:\mathrm{continued}…. \\ $$
Commented by mnjuly1970 last updated on 15/Oct/20
$${thank}\:{you}\:{so}\:{much}\:{sir}. \\ $$$${grateful}… \\ $$