Question Number 114950 by joki last updated on 22/Sep/20
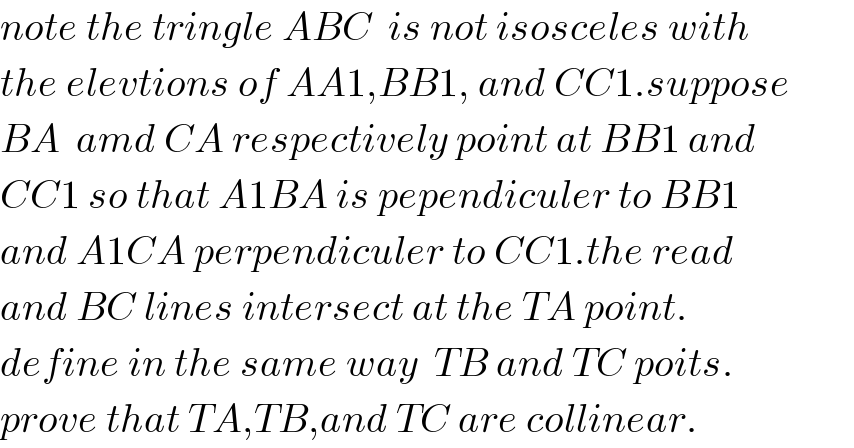
$${note}\:{the}\:{tringle}\:{ABC}\:\:{is}\:{not}\:{isosceles}\:{with}\: \\ $$$${the}\:{elevtions}\:{of}\:{AA}\mathrm{1},{BB}\mathrm{1},\:{and}\:{CC}\mathrm{1}.{suppose} \\ $$$${BA}\:\:{amd}\:{CA}\:{respectively}\:{point}\:{at}\:{BB}\mathrm{1}\:{and} \\ $$$${CC}\mathrm{1}\:{so}\:{that}\:{A}\mathrm{1}{BA}\:{is}\:{pependiculer}\:{to}\:{BB}\mathrm{1} \\ $$$${and}\:{A}\mathrm{1}{CA}\:{perpendiculer}\:{to}\:{CC}\mathrm{1}.{the}\:{read}\: \\ $$$${and}\:{BC}\:{lines}\:{intersect}\:{at}\:{the}\:{TA}\:{point}. \\ $$$${define}\:{in}\:{the}\:{same}\:{way}\:\:{TB}\:{and}\:{TC}\:{poits}. \\ $$$${prove}\:{that}\:{TA},{TB},{and}\:{TC}\:{are}\:{collinear}. \\ $$
