one-cube-of-side-length-3cm-is-painted-by-three-colour-say-red-blue-and-black-opposte-face-same-colour-Now-cube-is-cut-into-27-small-cube-i-How-many-cube-has-three-face-coloured-ii-How-many-cube-two

FacebookTweetPin

Question Number 119463 by TANMAY PANACEA last updated on 24/Oct/20

$$onecubeofsidelength3cmispaintedbythreecolour$$$$say\left(redblueandblack\right)oppostefacesamecolour$$$$Nowcubeiscutinto27smallcube$$$$i)Howmanycubehasthreefacecoloured$$$$ii)Howmanycubetwofacecoloured$$$$iii)Howmanycubehasnocolourinitsface$$

Commented by TANMAY PANACEA last updated on 25/Oct/20

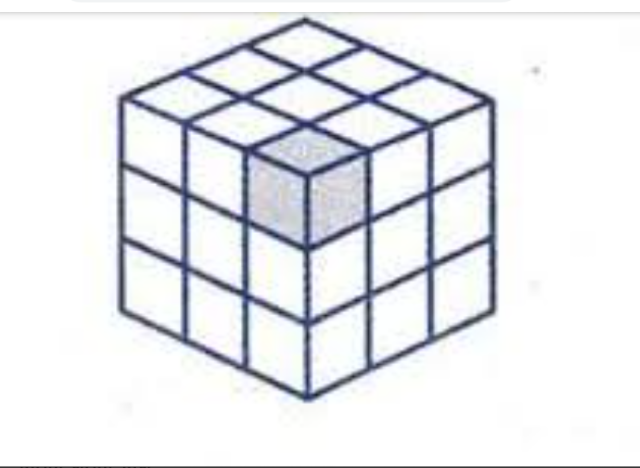
Commented by TANMAY PANACEA last updated on 25/Oct/20

$$1)threefacecoloured=8\left(cubeatcorner\right)$$$$2)seetopmostlayerandbottommostlayer$$$$4\times 2=8cubetwofacecoloured.$$$$middlelayer4cubeofcorner$$$$sototal=8+4=12$$$$3)seetopmostandbottommostfacecentredcube$$$$onefacecolouredandmiddlelayer4cube$$$$=2+4=6cubeonefacecoloured$$$$4)nofacecoloured=1cube$$$$\mathit{s}\mathit{o}$$$$\mathit{t}\mathit{h}\mathit{r}\mathit{e}\mathit{e}\left(\mathit{o}3\right)\mathit{f}\mathit{a}\mathit{c}\mathit{e}\mathit{c}\mathit{o}\mathit{l}\mathit{o}\mathit{u}\mathit{r}\mathit{e}\mathit{d}=8$$$$\mathit{t}\mathit{w}\mathit{o}\mathit{f}\mathit{a}\mathit{c}\mathit{e}\mathit{c}\mathit{o}\mathit{l}\mathit{o}\mathit{u}\mathit{r}\mathit{e}\mathit{d}=12$$$$\mathit{o}\mathit{n}\mathit{e}\mathit{f}\mathit{a}\mathit{c}\mathit{e}\mathit{c}\mathit{o}\mathit{l}\mathit{o}\mathit{u}\mathit{r}\mathit{e}\mathit{d}=6$$$$\mathit{n}\mathit{o}\mathit{f}\mathit{a}\mathit{c}\mathit{e}\mathit{c}\mathit{o}\mathit{l}\mathit{o}\mathit{u}\mathit{r}\mathit{e}\mathit{d}=1$$$$8+12+6+1=27$$

Answered by som(math1967) last updated on 25/Oct/20

$$\mathrm{i})8\mathrm{cubes}$$$$\mathrm{ii})8\mathrm{cubes}$$$$\mathrm{iii})1\mathrm{cube}$$$$$$

Commented by som(math1967) last updated on 25/Oct/20

$$\mathrm{Thik}\mathrm{bollam}?$$$$\mathrm{Thik}\mathrm{bollam}?$$

Terms of Service
Privacy Policy
Contact: info@tinkutara.com

FacebookTweetPin