Question Number 19795 by Tinkutara last updated on 15/Aug/17
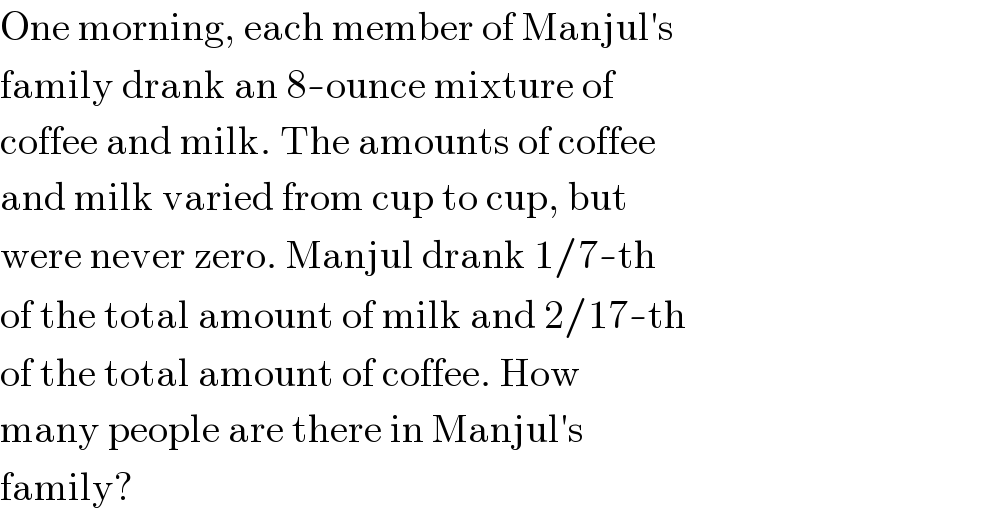
$$\mathrm{One}\:\mathrm{morning},\:\mathrm{each}\:\mathrm{member}\:\mathrm{of}\:\mathrm{Manjul}'\mathrm{s} \\ $$$$\mathrm{family}\:\mathrm{drank}\:\mathrm{an}\:\mathrm{8}-\mathrm{ounce}\:\mathrm{mixture}\:\mathrm{of} \\ $$$$\mathrm{coffee}\:\mathrm{and}\:\mathrm{milk}.\:\mathrm{The}\:\mathrm{amounts}\:\mathrm{of}\:\mathrm{coffee} \\ $$$$\mathrm{and}\:\mathrm{milk}\:\mathrm{varied}\:\mathrm{from}\:\mathrm{cup}\:\mathrm{to}\:\mathrm{cup},\:\mathrm{but} \\ $$$$\mathrm{were}\:\mathrm{never}\:\mathrm{zero}.\:\mathrm{Manjul}\:\mathrm{drank}\:\mathrm{1}/\mathrm{7}-\mathrm{th} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{total}\:\mathrm{amount}\:\mathrm{of}\:\mathrm{milk}\:\mathrm{and}\:\mathrm{2}/\mathrm{17}-\mathrm{th} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{total}\:\mathrm{amount}\:\mathrm{of}\:\mathrm{coffee}.\:\mathrm{How} \\ $$$$\mathrm{many}\:\mathrm{people}\:\mathrm{are}\:\mathrm{there}\:\mathrm{in}\:\mathrm{Manjul}'\mathrm{s} \\ $$$$\mathrm{family}? \\ $$
Answered by Tinkutara last updated on 19/Aug/17
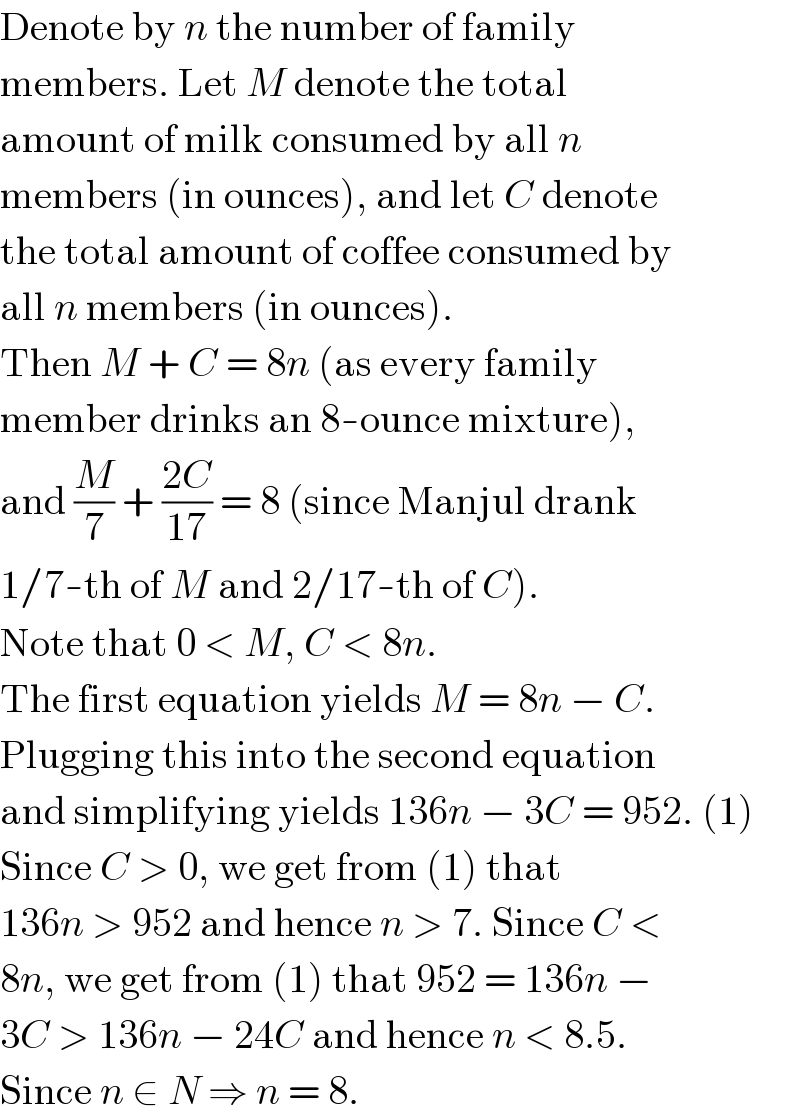
$$\mathrm{Denote}\:\mathrm{by}\:{n}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{family} \\ $$$$\mathrm{members}.\:\mathrm{Let}\:{M}\:\mathrm{denote}\:\mathrm{the}\:\mathrm{total} \\ $$$$\mathrm{amount}\:\mathrm{of}\:\mathrm{milk}\:\mathrm{consumed}\:\mathrm{by}\:\mathrm{all}\:{n} \\ $$$$\mathrm{members}\:\left(\mathrm{in}\:\mathrm{ounces}\right),\:\mathrm{and}\:\mathrm{let}\:{C}\:\mathrm{denote} \\ $$$$\mathrm{the}\:\mathrm{total}\:\mathrm{amount}\:\mathrm{of}\:\mathrm{coffee}\:\mathrm{consumed}\:\mathrm{by} \\ $$$$\mathrm{all}\:{n}\:\mathrm{members}\:\left(\mathrm{in}\:\mathrm{ounces}\right). \\ $$$$\mathrm{Then}\:{M}\:+\:{C}\:=\:\mathrm{8}{n}\:\left(\mathrm{as}\:\mathrm{every}\:\mathrm{family}\right. \\ $$$$\left.\mathrm{member}\:\mathrm{drinks}\:\mathrm{an}\:\mathrm{8}-\mathrm{ounce}\:\mathrm{mixture}\right), \\ $$$$\mathrm{and}\:\frac{{M}}{\mathrm{7}}\:+\:\frac{\mathrm{2}{C}}{\mathrm{17}}\:=\:\mathrm{8}\:\left(\mathrm{since}\:\mathrm{Manjul}\:\mathrm{drank}\right. \\ $$$$\left.\mathrm{1}/\mathrm{7}-\mathrm{th}\:\mathrm{of}\:{M}\:\mathrm{and}\:\mathrm{2}/\mathrm{17}-\mathrm{th}\:\mathrm{of}\:{C}\right). \\ $$$$\mathrm{Note}\:\mathrm{that}\:\mathrm{0}\:<\:{M},\:{C}\:<\:\mathrm{8}{n}. \\ $$$$\mathrm{The}\:\mathrm{first}\:\mathrm{equation}\:\mathrm{yields}\:{M}\:=\:\mathrm{8}{n}\:−\:{C}. \\ $$$$\mathrm{Plugging}\:\mathrm{this}\:\mathrm{into}\:\mathrm{the}\:\mathrm{second}\:\mathrm{equation} \\ $$$$\mathrm{and}\:\mathrm{simplifying}\:\mathrm{yields}\:\mathrm{136}{n}\:−\:\mathrm{3}{C}\:=\:\mathrm{952}.\:\left(\mathrm{1}\right) \\ $$$$\mathrm{Since}\:{C}\:>\:\mathrm{0},\:\mathrm{we}\:\mathrm{get}\:\mathrm{from}\:\left(\mathrm{1}\right)\:\mathrm{that} \\ $$$$\mathrm{136}{n}\:>\:\mathrm{952}\:\mathrm{and}\:\mathrm{hence}\:{n}\:>\:\mathrm{7}.\:\mathrm{Since}\:{C}\:< \\ $$$$\mathrm{8}{n},\:\mathrm{we}\:\mathrm{get}\:\mathrm{from}\:\left(\mathrm{1}\right)\:\mathrm{that}\:\mathrm{952}\:=\:\mathrm{136}{n}\:− \\ $$$$\mathrm{3}{C}\:>\:\mathrm{136}{n}\:−\:\mathrm{24}{C}\:\mathrm{and}\:\mathrm{hence}\:{n}\:<\:\mathrm{8}.\mathrm{5}. \\ $$$$\mathrm{Since}\:{n}\:\in\:{N}\:\Rightarrow\:{n}\:=\:\mathrm{8}. \\ $$
