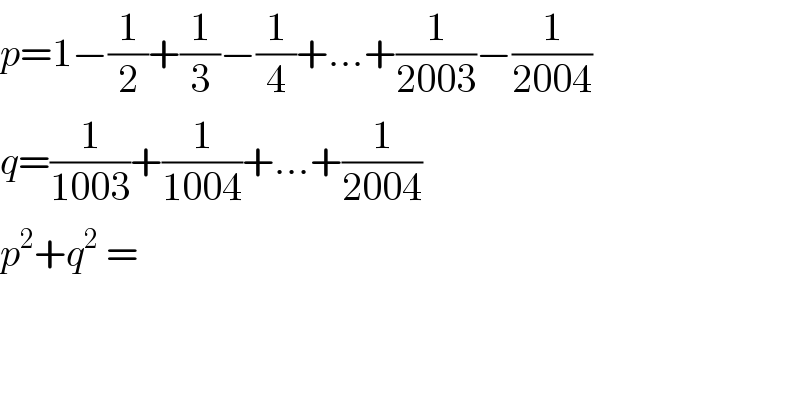
p-1-1-2-1-3-1-4-1-2003-1-2004-q-1-1003-1-1004-1-2004-p-2-q-2-

Question Number 91277 by john santu last updated on 29/Apr/20

p = 1 - \frac{1}{2} + \frac{1}{3} - \frac{1}{4} + \dots + \frac{1}{2003} - \frac{1}{2004}

q = \frac{1}{1003} + \frac{1}{1004} + \dots + \frac{1}{2004}

p^{2} + q^{2} =

Answered by naka3546 last updated on 30/Apr/20

Commented by jagoll last updated on 30/Apr/20

i t h i n k i t w r o n g a n s w e r

Commented by naka3546 last updated on 30/Apr/20

S h o w y o u r w o r k i n g s, p l e a s e

Answered by naka3546 last updated on 30/Apr/20

Facebook Tweet Pin