p-3-q-3-p-2-q-2-c-2-0-find-p-q-in-terms-of-c-if-c-2-lt-4-9-

Question Number 175462 by ajfour last updated on 30/Aug/22

p^{3} + q^{3} + p^{2} + q^{2} + c^{2} = 0

f i n d p + q i n t e r m s o f c . i f c^{2} < \frac{4}{9} .

Commented by mr W last updated on 01/Sep/22

i t h i n k t h e r e i s n o u n i q u e v a l u e p + q,

b u t t h e r e a r e {(p + q)}_{m i n} a n d {(p + q)}_{m a x} .

Commented by ajfour last updated on 01/Sep/22

v a l u e s m a y b e m u l t i p l e b u t a r e

c o n s t a n t s, i t h i n k .

Commented by mr W last updated on 01/Sep/22

f o r p, q \in R

- \frac{2}{\sqrt[3]{\frac{1}{c^{2}} (\sqrt{1 + \frac{8}{27 c^{2}}} + 1)} - \sqrt[3]{\frac{1}{c^{2}} (\sqrt{1 + \frac{8}{27 c^{2}}} - 1)}} ⩽ p + q < - \frac{2}{3}

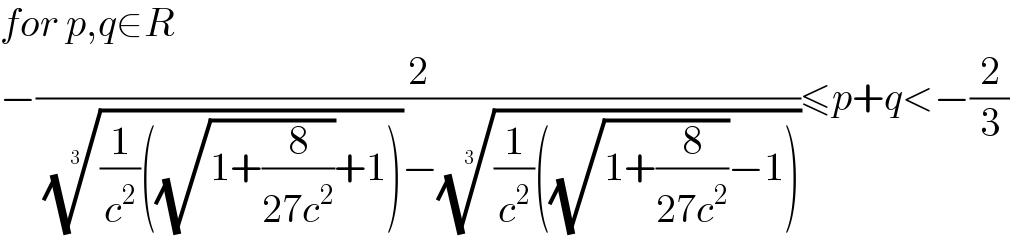
Answered by mr W last updated on 04/Sep/22

l e t s = p + q

s^{3} - 3 s p q + s^{2} - 2 p q + c^{2} = 0

s^{3} - 3 s p (s - p) + s^{2} - 2 p (s - p) + c^{2} = 0

(3 s + 2) p^{2} - s (3 s + 2) p + s^{3} + s^{2} + c^{2} = 0

Δ = s^{2} {(3 s + 2)}^{2} - 4 (3 s + 2) (s^{3} + s^{2} + c^{2}) ⩾ 0

(3 s + 2) (s^{3} + 2 s^{2} + 4 c^{2}) ⩽ 0

\Rightarrow 3 s + 2 < 0 \Rightarrow s < - \frac{2}{3}

\Rightarrow s^{3} + 2 s^{2} + 4 c^{2} ⩾ 0 \Rightarrow s ⩾ - \frac{2}{\sqrt[3]{\frac{1}{c^{2}} (\sqrt{1 + \frac{8}{27 c^{2}}} + 1)} - \sqrt[3]{\frac{1}{c^{2}} (\sqrt{1 + \frac{8}{27 c^{2}}} - 1)}}

i . e . - \frac{2}{\sqrt[3]{\frac{1}{c^{2}} (\sqrt{1 + \frac{8}{27 c^{2}}} + 1)} - \sqrt[3]{\frac{1}{c^{2}} (\sqrt{1 + \frac{8}{27 c^{2}}} - 1)}} ⩽ p + q < - \frac{2}{3}

Commented by Tawa11 last updated on 15/Sep/22

Great sir .

Facebook Tweet Pin