Question Number 177820 by cortano1 last updated on 09/Oct/22
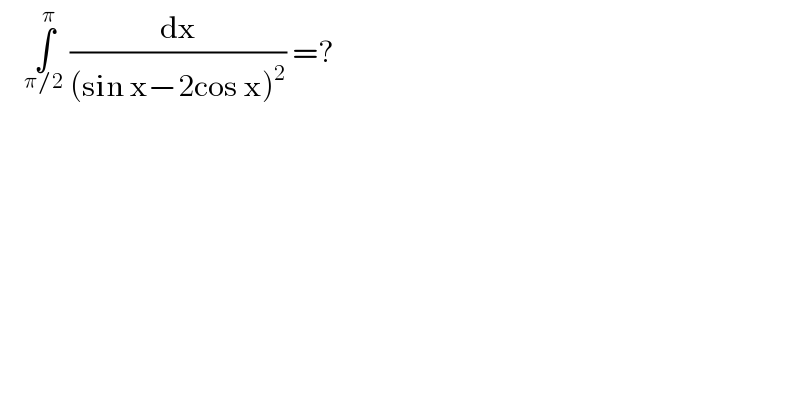
$$\:\:\:\:\underset{\pi/\mathrm{2}} {\overset{\pi} {\int}}\:\frac{\mathrm{dx}}{\left(\mathrm{sin}\:\mathrm{x}−\mathrm{2cos}\:\mathrm{x}\right)^{\mathrm{2}} }\:=? \\ $$
Answered by qaz last updated on 09/Oct/22
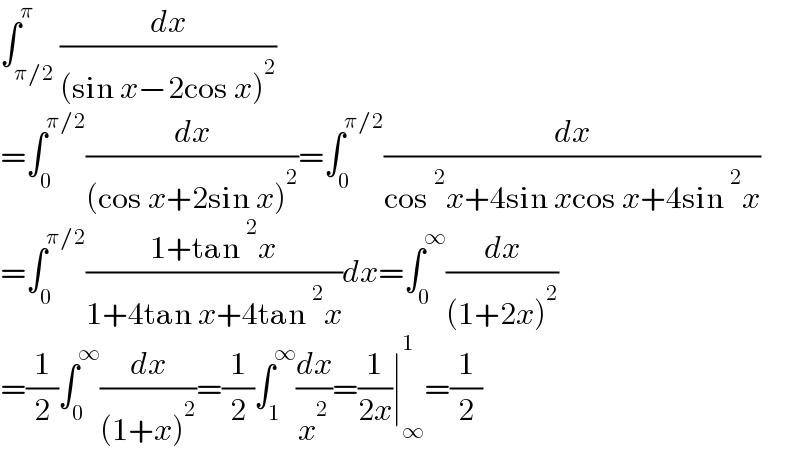
$$\int_{\pi/\mathrm{2}} ^{\pi} \frac{{dx}}{\left(\mathrm{sin}\:{x}−\mathrm{2cos}\:{x}\right)^{\mathrm{2}} } \\ $$$$=\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \frac{{dx}}{\left(\mathrm{cos}\:{x}+\mathrm{2sin}\:{x}\right)^{\mathrm{2}} }=\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \frac{{dx}}{\mathrm{cos}\:^{\mathrm{2}} {x}+\mathrm{4sin}\:{x}\mathrm{cos}\:{x}+\mathrm{4sin}\:^{\mathrm{2}} {x}} \\ $$$$=\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \frac{\mathrm{1}+\mathrm{tan}\:^{\mathrm{2}} {x}}{\mathrm{1}+\mathrm{4tan}\:{x}+\mathrm{4tan}\:^{\mathrm{2}} {x}}{dx}=\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{\left(\mathrm{1}+\mathrm{2}{x}\right)^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{1}} ^{\infty} \frac{{dx}}{{x}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}{x}}\mid_{\infty} ^{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Answered by Frix last updated on 09/Oct/22
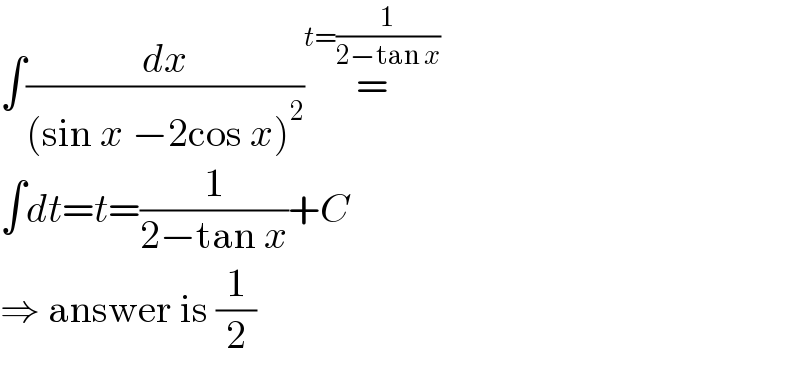
$$\int\frac{{dx}}{\left(\mathrm{sin}\:{x}\:−\mathrm{2cos}\:{x}\right)^{\mathrm{2}} }\overset{{t}=\frac{\mathrm{1}}{\mathrm{2}−\mathrm{tan}\:{x}}} {=} \\ $$$$\int{dt}={t}=\frac{\mathrm{1}}{\mathrm{2}−\mathrm{tan}\:{x}}+{C} \\ $$$$\Rightarrow\:\mathrm{answer}\:\mathrm{is}\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$
