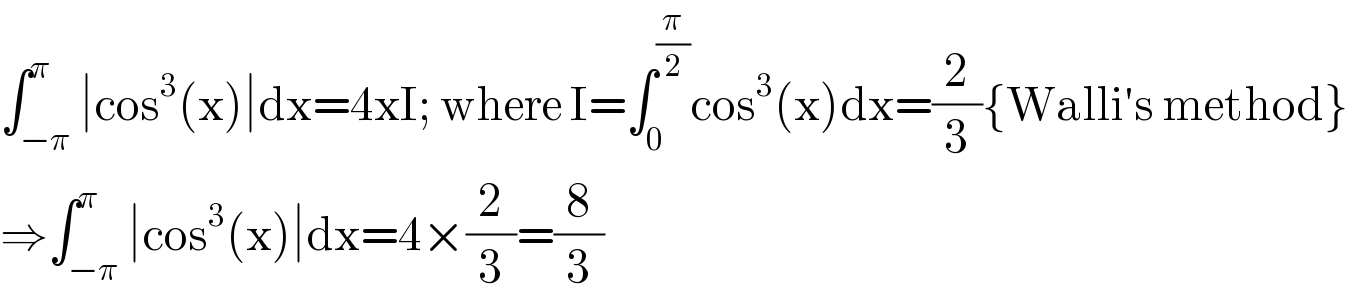Question Number 95549 by Fikret last updated on 25/May/20
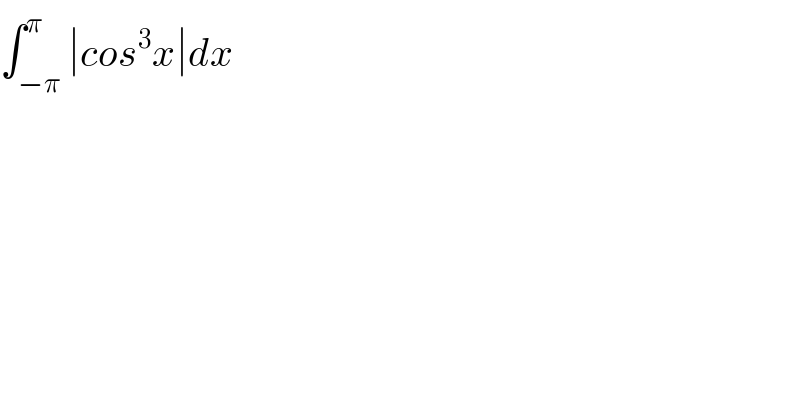
Answered by MJS last updated on 26/May/20
![∫_(−π) ^π ∣cos^3 x∣dx=4∫_0 ^(π/2) cos^3 x dx= =∫_0 ^(π/2) (cos 3x +3cos x)dx= =[(1/3)sin 3x +3sin x]_0 ^(π/2) =(8/3)](https://www.tinkutara.com/question/Q95556.png)
Commented by peter frank last updated on 26/May/20
Commented by MJS last updated on 26/May/20
Commented by peter frank last updated on 26/May/20
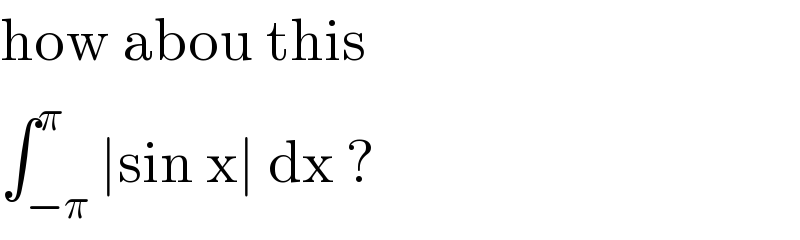
Commented by MJS last updated on 26/May/20
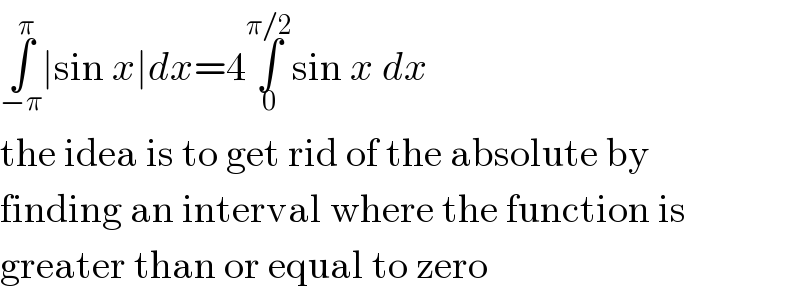
Commented by peter frank last updated on 26/May/20

Answered by 1549442205 last updated on 26/May/20
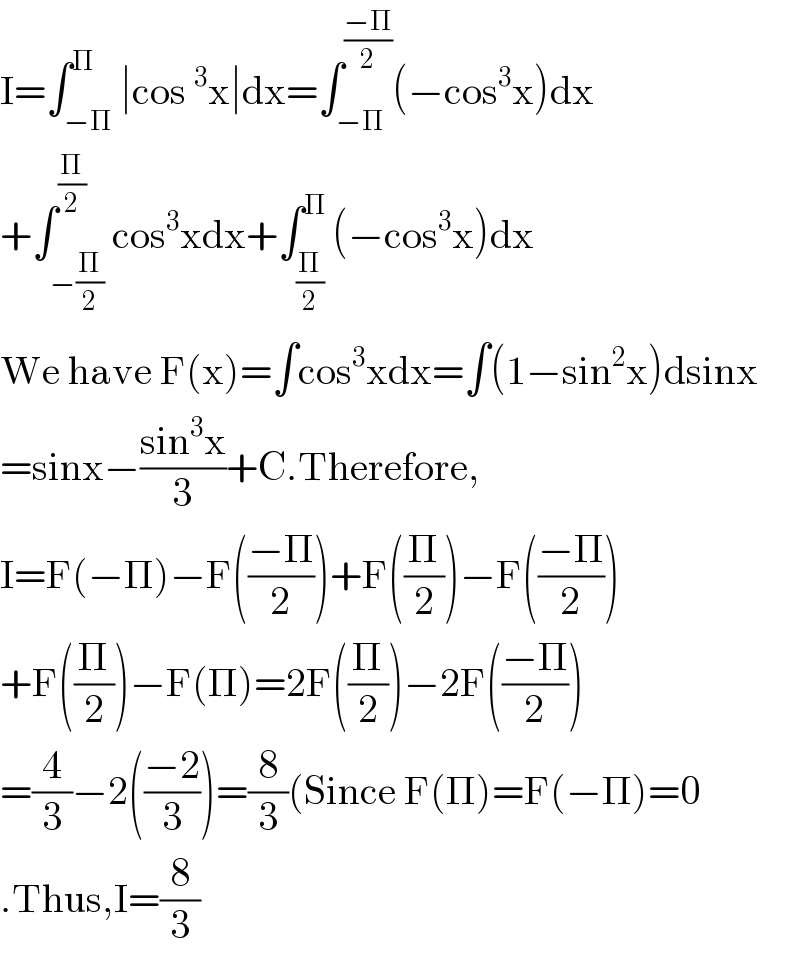
Answered by Ar Brandon last updated on 26/May/20